
Question and Answers Forum
IntegrationQuestion and Answers: Page 315

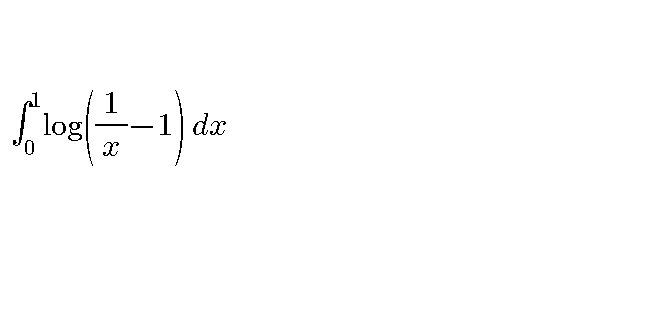
Pg 310 Pg 311 Pg 312 Pg 313 Pg 314 Pg 315 Pg 316 Pg 317 Pg 318 Pg 319
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 315 |
| Show that the integral: ∫ e^(−x^2 ) dx Can′t be calculated trivially. |
| prove that ∫_0 ^∞ (t^(x−1) /(e^t −1))dt =ξ(x)Γ(x) with ξ(x)= Σ_(n=1) ^∝ (1/n^x ) and Γ(x)=∫_0 ^∞ t^(x−1) e^(−t) dt ( x>1) |
| find f(x)= ∫_0 ^∞ (e^(−x(1+t^2 )) /(1+t^2 )) dt interms ofx with x≥0 and calculate ∫_0 ^∞ e^(−t^2 ) dt . |
| prove that ∫_0 ^∞ e^(−(t^2 +(1/t^2 ))) dt is convergeny and find its value . |
| find the value of ∫_0 ^∝ (((−1)^([x]) )/((2x+1)^2 ))dx |
| ∫log(2+x^2 )dx |
| Proof ∫(1/(a^2 −x^2 ))dx =(1/(2a))ln∣((a+x)/(a−x))∣+c |
| find the value of ∫_(−1) ^1 (dx/((√(1−x^2 )) +(√(1+x^2 )))) . |
| find I= ∫_0 ^∝ ((cosx)/(cosh(x)))dx |
| find I=∫_0 ^π (dx/(cosx +2sinx)) . |
| find ∫∫_D (x+y)^2 e^(x^2 −y^2 ) dxdy with D={(x,y)∈R^(2 ) /0<x<1 and 0<y<1−x }. |
| calculate in terms of x f(x)= ∫_0 ^(π/(2 )) (dt/(1+xsint)) . |
| find the value of I= ∫_0 ^1 ((t−1)/(lnt))dt . |
| find the value of I_a = ∫∫_D_a e^(−((x^2 +y^2 )/2)) dxdy with D_a ={(x,y)∈R^2 / x^2 +y^2 ≤ a^2 } |
| ∫3x^2 /x^6 +1 |
| find the value of ∫_(2/π) ^(6/π) x^3 cos([(1/x)])dx |
| xy=(1−x^2 )(dy/dx) x=0 y=1 |
| xy=(1−x^2 )(dy/dx) x=0 y=1 |
| ∫_(1/8) ^(1/2) ⌊ln ⌈(1/x)⌉⌋ dx |

|
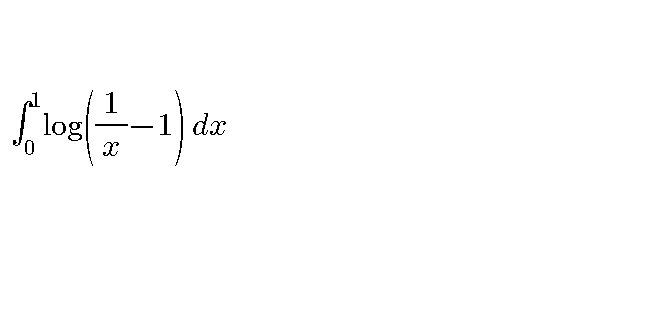
|
| find the value of ∫_0 ^( ∝) (dx/((x+1)(x+2)(x+3))) . |
| let give D={( x,y )∈R^2 /x^2 −x +y^2 ≤ 4 and 0≤y≤1} calculate ∫∫_D ln(xy)(√( x^2 +y^2 dxdy )) |
| give the decomposition of F(x) = (1/(x^(2n) +1)) inside C[x] then find the value of ∫_0 ^∞ (dx/(1+x^(2n) )) n∈N and n≠o |
| prove that ∫_0 ^1 (dx/(x+ e^x )) = Σ_(n=0) ^∝ (((−1)^n )/((n+1)^(n+1) )) A_n with A_n = ∫_0 ^(n+1) t^n e^(−t) dt . |
| find ∫ (dx/(x^6 −1)) . |
Pg 310 Pg 311 Pg 312 Pg 313 Pg 314 Pg 315 Pg 316 Pg 317 Pg 318 Pg 319 |