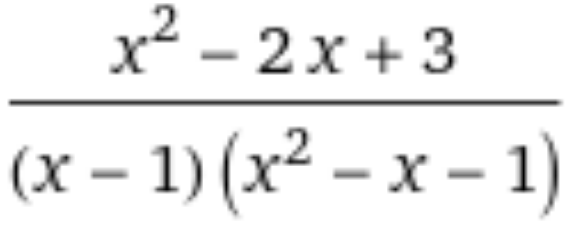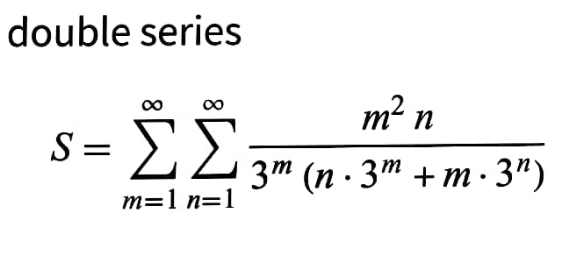Question and Answers Forum

All Questions Topic List

IntegrationQuestion and Answers: Page 37

Question Number 172088 Answers: 1 Comments: 0

Question Number 172072 Answers: 0 Comments: 0

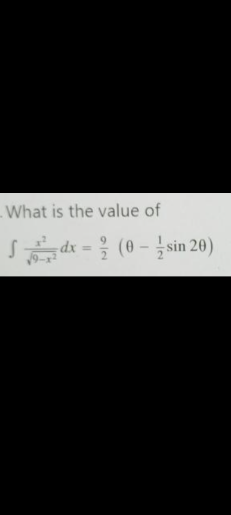
Question Number 172013 Answers: 1 Comments: 0

find: ∫xe^(−ax) ax

$f i n d :$ $\int {x e}^{- a x} a x$

Question Number 172012 Answers: 1 Comments: 0

find ∫e^x sinxdx

$f i n d$ $\int e^{x} s i n x d x$

Question Number 172011 Answers: 1 Comments: 0

find integrate: ∫x^2 e^x dx

$f i n d i n t e g r a t e :$ $\int x^{2} e^{x} d x$

Question Number 172010 Answers: 1 Comments: 0

find integrate: ∫xe^x dx

$f i n d i n t e g r a t e :$ $\int {x e}^{x} d x$

Question Number 171971 Answers: 2 Comments: 0

∫(x/(x^2 +4x+3)) dx=...

$\int \frac{x}{x^{2} + 4 x + 3} d x = . . .$

Question Number 171910 Answers: 3 Comments: 0

∫ (dx/(9 − 4x^2 )) using the trigonometric substitution.

$\int \frac{dx}{9 - {4 x}^{2}}$ $using the trigonometric substitution .$

Question Number 171727 Answers: 3 Comments: 0

Question Number 171708 Answers: 1 Comments: 0

∫_0 ^∞ 2x−3 dx=...

$\int_{0}^{\infty} 2 x - 3 d x = . . .$

Question Number 171601 Answers: 0 Comments: 0

Question Number 171565 Answers: 0 Comments: 4

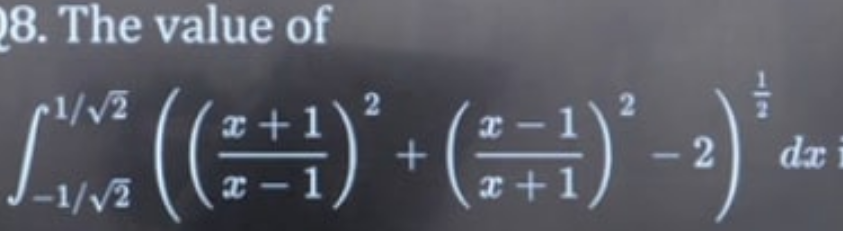
Question Number 171560 Answers: 1 Comments: 1

Nice Integral Ω = ∫_0 ^( (π/4)) (( tan(x))/(( cos^( 2) (x) + 2sin^( 2) (x))))dx =

$Nice Integral$ $Ω = \int_{0}^{\frac{π}{4}} \frac{t a n (x)}{({c o s}^{2} (x) + 2 {s i n}^{2} (x))} d x =$

Question Number 171442 Answers: 0 Comments: 1

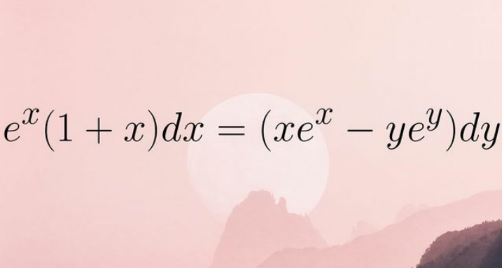
Question Number 171395 Answers: 1 Comments: 2

∫_(−∞) ^(+∞) (x^2 /(1+x^4 ))dx

$\int_{- \infty}^{+ \infty} \frac{x^{2}}{1 + x^{4}} d x$

Question Number 171392 Answers: 0 Comments: 1

∫_0 ^(π/2) ((cos x)/((1+(√(sin 2x)) )^3 )) dx =?

$\underset{0}{\int^{\frac{π}{2}}} \frac{\cos x}{{(1 + \sqrt{\sin 2 x})}^{3}} d x = ?$

Question Number 171371 Answers: 3 Comments: 0

∫_(−∞) ^(+∞) (dx/(1+x^4 ))=?

$\int_{- \infty}^{+ \infty} \frac{d x}{1 + x^{4}} = ?$

Question Number 171367 Answers: 0 Comments: 0

Question Number 171301 Answers: 0 Comments: 1

∫_1 ^2 6x^2 −2x+3

$\int_{1}^{2} 6 x^{2} - 2 x + 3$

Question Number 171260 Answers: 1 Comments: 0

Change to polar coordinates: ∫^( 4a) _0 ∫_(y^2 /4a) ^a (((x^2 −y^2 )/(x^2 +y^2 ))) dx dy

$\underset{―}{C h a n g e t o p o l a r c o o r d i n a t e s :}$ ${\int_{0}}^{4 a} \int_{y^{2} / 4 a} \overset{a}{} (\frac{x^{2} - y^{2}}{x^{2} + y^{2}}) d x d y$

Question Number 171253 Answers: 2 Comments: 0

Question Number 171213 Answers: 0 Comments: 0

In electricity, the electrostatic field is defined as: E = ∫_0 ^π [((a^2 σ sin θ)/(2ε(√(a^2 −x^2 −2ax cosθ))))]dθ where a,σ and ε are constants. Consider that x>a and show that E= ((a^2 σ)/(εx))

$In electricity, the electrostatic field$ $is defined as :$ $E = \int_{0}^{π} [\frac{a^{2} σ \sin θ}{2 ϵ \sqrt{a^{2} - x^{2} - 2 a x \cos θ}}] d θ$ $where a, σ and ϵ are constants . Consider$ $that x > a and show that E = \frac{a^{2} σ}{ϵ x}$

Question Number 171198 Answers: 1 Comments: 0

evaluate ∫_0 ^( π) log (a+cos x)dx

$e v a l u a t e$ $\int_{0}^{π} \log (a + \cos x) d x$

Question Number 171171 Answers: 1 Comments: 2

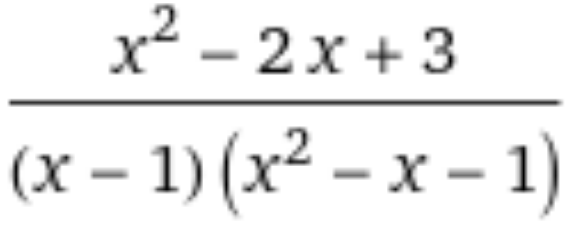
Question Number 171130 Answers: 0 Comments: 0

Question Number 171125 Answers: 1 Comments: 0

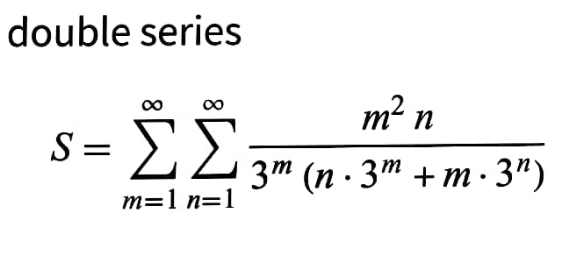
Pg 32 Pg 33 Pg 34 Pg 35 Pg 36 Pg 37 Pg 38 Pg 39 Pg 40 Pg 41

Contact: info@tinkutara.com