
Question and Answers Forum
IntegrationQuestion and Answers: Page 43





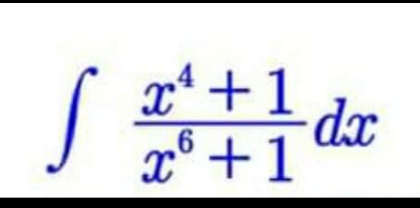
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 43 |
| solve I= ∫_0 ^( (1/2)) ((ln^( 2) (x))/(1−x)) dx =? |
| ∫_(−2) ^(−1) e^(−(t/2)) (√(t+2)) dt = ??? |

|

|
| Ω = ∫_0 ^( 1) ((Li_( 2) (1− x ))/(1+x)) dx = ? −−−−− |

|

|
| prove that Φ= ∫_0 ^( 1) x.ψ (2+x )= 2 −(1/2)ln(8π) −−− |
| ∫_0 ^( (π/2)) (1/(1+sin^6 x)) dx=? |
| ∫_0 ^(π/3) (√(1−(1/(3 ))sin^2 θ)) dθ |
| ∫sec θtan^4 θdθ |
| ∫ ((3x^3 )/((x−1)^3 )) dx=? |
| γ=∫ ((e^x (sin x+1))/(cos x+1)) dx =? |
| calculate ∫_0 ^( ∞) (((√x) arctan(x))/(1+x^( 2) ))dx =? |

|
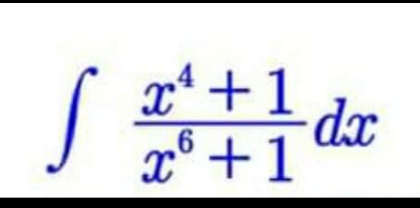
|
| Ω= Σ_(n=1) ^∞ (( H_( n) )/(n(n+1))) = −−−−−− Ω = Σ_(n=1) ^∞ −(1/(n+1)) ∫_(0 ) ^( 1) x^( n−1) ln(1−x )dx = ∫_0 ^( 1) {−(1/x^2 )ln(1−x).Σ_(n=1) (x^( n+1) /(n+1))}dx = ∫_0 ^( 1) {((−ln(1−x))/x^( 2) )Σ_(n=2) ^∞ (x^( n) /n)}dx = ∫_0 ^( 1) ((−ln(1−x))/x^( 2) ) {−x +Σ_(n=1) ^∞ (x^( n) /n) }dx = −li_( 2) ( 1) +[ ∫_0 ^( 1) ((ln^( 2) ( 1−x ))/x^( 2) )dx=_(derived) ^(earlier) (π^( 2) /3) ] = −(π^( 2) /6) + (π^( 2) /3) = (( π^( 2) )/6) = ζ (2) ■ m.n |
| calculate If , f(x)=(( (x^2 +1)(((x^( 2) +x−2)(x^( 4) −1)(x^( 2) +2x−3)+16))^(1/3) + (√(x^( 2) +3)))/(( 1+x +x^( 2) ))) then , f ′ (1 ) =? ■ m.n |
| solve in R i: ⌊ x ⌊ x⌋⌋= 3x ii : ⌊x ⌋^( 2) −3 ⌊x ⌋ +2 ≤ 0 −−−−−− |
| ∫ ((sin^3 x)/((cos^2 x+1)(√(cos^2 x+1)))) dx |
| ∫ (dx/(3+tan x))=? |
| ∫ (((x)^(1/5) −1)/( (√x) + 1)) dx=? |
| (8/(1×5×9))+(8/(5×9×13))+(8/(9×13×17))+…+(1/(41×45×49))=? by M.A |
| ∫e^x ln(x)dx=..??? |
| T = ∫ ((sin (x^2 +2))/(2x+4)) dx=? |
| calculate Ω = Σ_(n=0) ^∞ (1/((3n)!)) = ? |