
Question and Answers Forum
IntegrationQuestion and Answers: Page 51







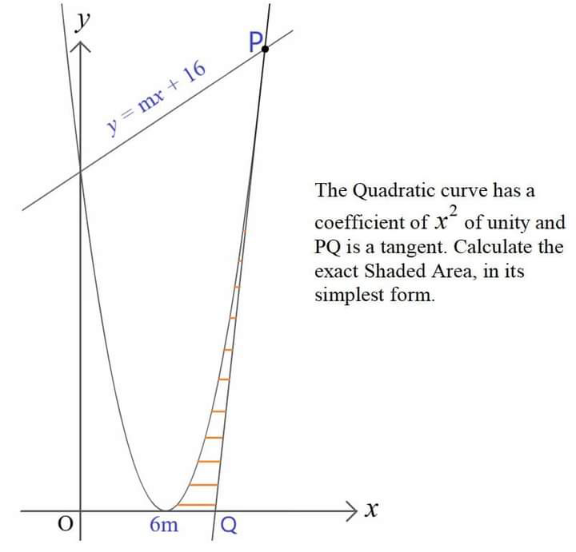
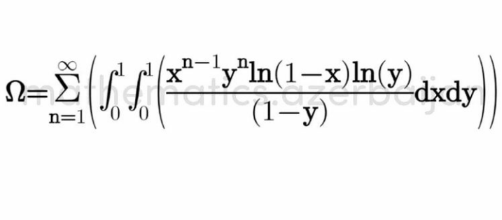
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 51 |
| ∫_0 ^1 ((xln(1+x^4 ))/(1+x^2 ))dx=? |
| ∫_0 ^( (π/4)) ((1+tan^4 (x))/(cot^2 (x))) dx =? |

|

|

|

|

|
| ∫_1 ^( 2) ((tan^(−1) (x−1)log(x))/x)dx |
| (1) ∫ (dx/(1−2cos x)) (2) ∫ ((sin 2x)/(sin x−sin^2 2x)) dx (3) ∫ (dx/(cos 2x−sin x)) |

|

|
| Given f(x)=f(x+2), ∀x∈R If ∫_0 ^2 f(x)dx= p then ∫_0 ^(2020) f(x+2a)dx=? for a∈Z^+ |

|
| Given f(x)= { ((1−∣x∣ ; x≤1)),((∣x∣−1 ; x>1)) :} find ∫_(−3) ^( 8) [f(x−1)+f(x+1)] dx. |
| ∫_( 0) ^( (π/2)) ((x sin x cos x)/(cos^4 x +sin^4 x)) dx =? |
| ∫^∞ _2 ((arctg(x))/(arctg((x/2))))dx=??? |
| calculate Θ := Σ_(n=1) ^∞ (( (−1 )^( n−1) )/(n ( n + (1/3) ))) =? ■ m.n −−−−−−−−−−−−− |
| f(x^2 )= 2+∫_( 0) ^( x^2 ) f(y) (1−tan y)dy , ∀x∈R f(−π)=? |
| prove that I= ∫_0 ^( (π/2)) ln ( 1+ sin (2 α )) dα = 2G − π ln ((√2) ) G: catalan constant |
| Ω = ∫_0 ^( ∞) ((ln (1+ x ))/((1+ x^( 2) )^( 2) )) dx = ? −−−−−−−−−−−− |
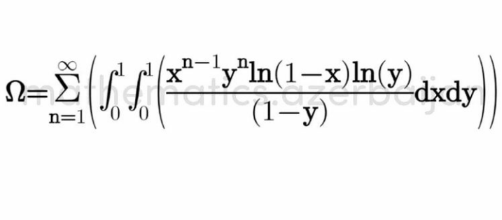
|
| Ω = ∫_0 ^( 1) (( ln (−ln (x)))/(1+x)) dx =^? ((−1)/2) ln^( 2) (2) |
| Ω=∫_0 ^1 x^(n−1) ln(1−x)dx=??? n≥1 |
| calculate Ω = Σ_(n=1) ^∞ (( ζ ( 1+ n ) −1)/(n + 1)) =^? 1− γ −−−−−−−−−−− |
| ∫ (dx/( (√(sin^3 x)) (√(cos^5 x)))) =? |
| ∫ ((sec x)/( (√(1+2sec x)))) (√((cosec x−cot x)/(cosec x+cot x))) dx =? |