
Question and Answers Forum
IntegrationQuestion and Answers: Page 58
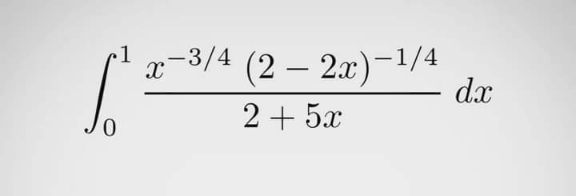
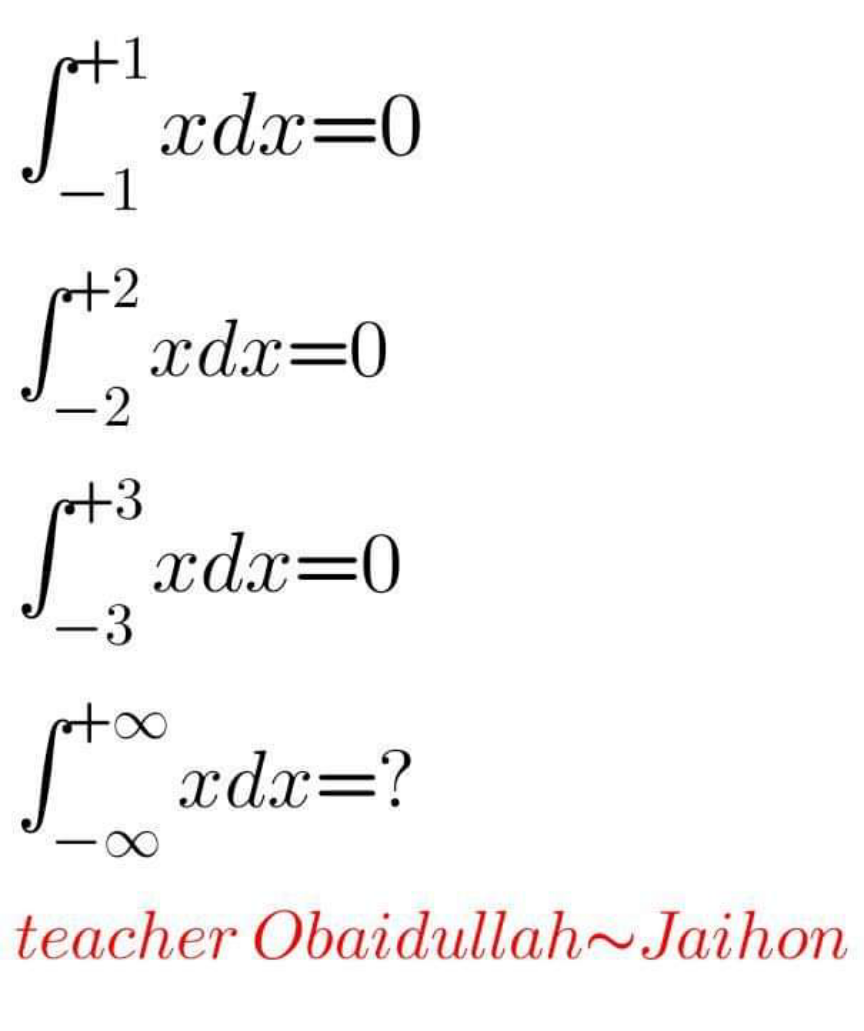

|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 58 |
| ∫ (dx/(3−tan x)) =? |
| ∫(dx/(sin^4 x)) |
| ∫ (dx/((1+(x)^(1/4) )(√x))) =? |
| prove that: I=∫_0 ^( ∞) x^( 2) tanh(x).e^( −x) dx=(π^( 3) /8) −2 |
| prove that tan^(−1) (((xy)/(rz)))+tan^(−1) (((xz)/(ry)))+tan^(−1) (((yz)/(rx)))=(π/2) |
| ∫(dx/(sin x+ sec x)) using wiestress substitution |
| find the integral: ∫{(3x+1)/(x^2 +4)}dx |
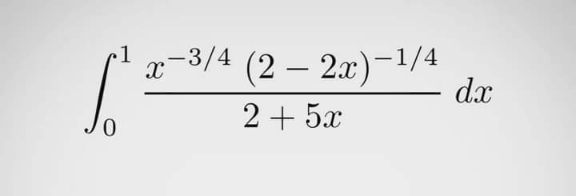
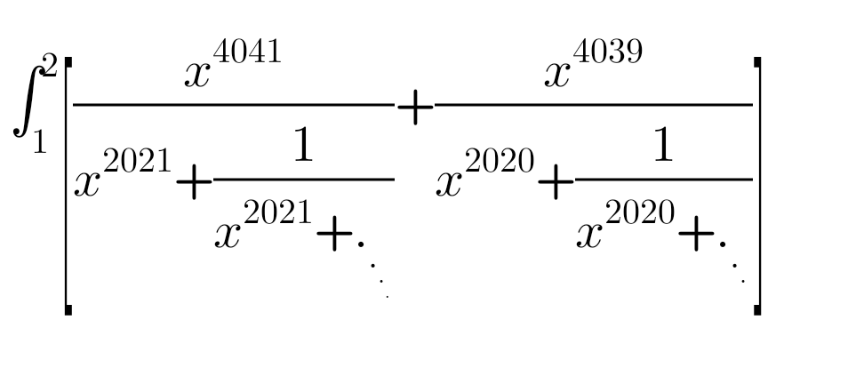
|
| calculate : Ω := Σ_(n=0) ^∞ (( 1)/((4n+1)^( 3) )) = ? |
| ∫_0 ^( 1) (( Li_( 2) (−x ) )/(1+ x))dx=? |
| ∫_0 ^∞ ((1−cos 4x)/(xe^x )) dx=? |
| x^2 f(x^3 )+(1/((1+x)^2 )) f(((1−x)/(1+x)))=4x^3 (1+x^4 )^5 ∫_( 0) ^( 1) f(x) dx =? |

|
| ∫ ((x sin x)/(16x+9)) dx |
| calculate : Ω:= ∫_(0 ) ^( 1) (( ln(1+x).ln(1−x))/(1+x)) dx =? |
| ∫ 5^(3−2x) dx =? |

|
| ∫ (dx/(x−(√(x^2 +2x+2)))) |
| ∫_( 0) ^( (π/2)) (x/(sin^8 x+cos^8 x)) dx ? |
| ∫ (dx/( (√x)+x(√(x+1)))) =? |
| prove that ∫(x^2 /((xsin x+cos x)^2 ))dx=−((xsec x)/(xsin x+cos x))+tan x+c |
| ∫ ((sin^6 x+cos^5 x)/(sin^2 x cos^2 x)) dx |
| # Nice Mathematics # ...calculation ... Ω :=∫_0 ^( 1) (( tanh^( −1) ((√( x)) ))/x) dx =^? (( π^( 2) )/4) −−−−−−−−−−−−− Ω :=^((√x) = t) 2∫_0 ^( 1) (( tanh^( −1) (t ))/t) dt :=^({tanh^( −1) (t )= (1/2) ln( ((1+t)/(1−t)) ) }) ∫_0 ^( 1) ((ln( 1+t )− ln(1−t ))/t) dt : = −Li_( 2) (−1 ) + Li_( 2) (1 ) :=^( {Li_( 2) (z )= Σ_(n=1) ^∞ (( z^( n) )/n^( 2) ) }) η (2) + ζ (2) := (π^( 2) /(12)) + (π^( 2) /6) = (( π^( 2) )/( 4)) ■ m.n |
| Show that ∫_0 ^1 (1/((x+1)(x+2)))dx = ln((4/3)) |

|