
Question and Answers Forum
IntegrationQuestion and Answers: Page 62


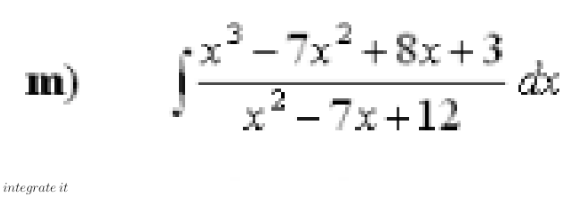
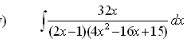



|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 62 |
| ∫_0 ^(π/2) arcos(((cosx)/(1+2cosx)))dx |
| ∫((a^2 sin^2 θ+b^2 cos^2 θ)/(a^4 sin^2 θ+b^4 cos^2 θ))dθ |
| ∫[((x/e))^x +((e/x))^x ]ln xdx |
| nice calculus.. prove that : I:=∫_0 ^( ∞) (( (1+e^( −x) )sin^( 2) (x))/x^( (3/2)) ) =(√(2π)) ( 1+ (√((√2) − 1)) ) m.n |
| ∫(x^(n−1) /(x^(3n+1) (x^n −1)))dx=? |

|

|
| ∫_1 ^3 ⌊x−3⌋dx |
| ∫_0 ^( 1) ∫_0 ^( z^2 ) ∫_0 ^( 3) y cos (z^5 )dxdydz =? |
| prove that: Im( ψ ( i ) )= (( 1)/( 2)) + (( π)/2) coth(π ) m.n |
| ∫_0 ^( 1) (x^( i+1) /(1−x)) dx = [−ln(1−x)x^( i+1) ]_0 ^( 1) + (1+i)∫_0 ^( 1) x^( i) ln (1−x )dx = (1+i ) ∫_0 ^( 1) Σ_(n=1) ^∞ (x^( n+i) /n)dx = (1+i )Σ(1/(n (n+i+1 )))= = Σ(1/(n )) −(1/(n+i+1)) = γ + ψ ( i+2 ) = γ + (1/(1+i)) +ψ (1+i) γ + ((1−i)/2) + (1/i) + ψ (i ) im = −(3/2) + (1/2) +(π/2) coth(π ) −1 +(π/2) tan h(π ) ..✓ |
| ∵∴∵∴prove that ∫_0 ^∞ ∫_0 ^∞ ((log(1−e^(−x) )(yLi_2 (e^(−x−y) )+Li_3 (e^(−x−y) ))/(1−e^(x+y) ))e^(x+y) dxdy=((21)/8)ζ(6)+ζ^2 (3) ∵∴∵by MATH.AMIN∴∵∴ |
| ∫((x+sinx)/(1+cosx))dx please,help me |
| Ω :=∫_0 ^( 1) (( x.sin(ln(x)))/(1−x))dx method 1 Ω= Im[∫_0 ^( 1) (( x^( i+1) )/(1−x)) dx=Φ] Φ = ∫_0 ^( 1) (( x^( i+1) +x^( i+2) )/(1−x^( 2) ))dx =^(x^( 2) =t) (1/2)∫_0 ^( 1) (( t^( (i/2)) −t^((i+1)/2) )/(1−t))dt = (1/2) { ψ (1 +((i+1)/2))−ψ (1+(i/2))} = (1/2) { (2/(1+i)) + ψ ( ((1+i)/2) )−(2/i)−ψ ((i/2))} = ((−i)/(−1+i)) + (1/2) {ψ (((1+i)/2))−ψ((i/2) )} = −(1/2) +(i/(2 )) +(1/2) {ψ(((1+i)/2))−ψ((i/2))} Ω = Im (Φ )= (1/2) +(1/2) Im(ψ((1/2) +(i/2)))−(1/2) Im((i/2)) = (1/(2 )) { 1 +(π/2) tanh((π/2)) −1−(π/2) coth((π/2))} =(π/4) {((−1)/(sinh((π/2)).cosh((π/2))))}=((−π)/(2 sinh (π ))) ✓ |
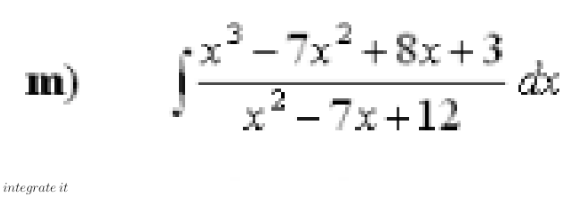
|
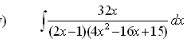
|

|

|
| Ω =∫_0 ^( (π/2)) ln^2 (((1+sin t)/(1−sin t)))dt |

|
| monster integral ∫_(−∞) ^( ∞) sin(x^2 )cos(x^3 ) dx |
| Prove:: Σ_(n=−∞) ^(+∞) arctan (((sinh x)/(cosh n)))=πx |
| ∫_0 ^( ∞) sin(x^2 )cos(x^3 )dx |
| show whether ∫_0 ^( ∞) sin(x^2 )cos((√x))dx is solvable |
| ∫_( 0) ^( ∞) a Π_(p → 1) ^∞ (((p^2 − x^(2n) )/p^2 ))dx, 1 < 2n < n + 1 |
| Prove that.. 𝛗 : =∫_( 1) ^( +∞) (( ln (x ))/(( x^( π) −1 )( ln^( 2) (x) +1 )^2 ))dx= ((π^( 2) − 8)/(16)) ■ |