
Question and Answers Forum
IntegrationQuestion and Answers: Page 63
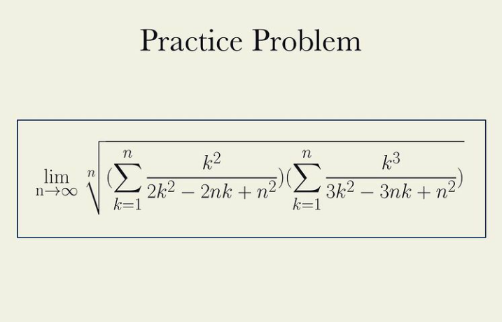

|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 63 |
| Prove that.. 𝛗 : =∫_( 1) ^( +∞) (( ln (x ))/(( x^( π) −1 )( ln^( 2) (x) +1 )^2 ))dx= ((π^( 2) − 8)/(16)) ■ |
| ∫_0 ^( ∞) (( x)/((1 +x^( 2) ) ( e^( 2πx) − 1))) dx =((2γ− 1)/4) |
| Ω= Σ_(n=1) ^∞ {n^2 (∫_0 ^( (π/2)) (( sin^( 2) (x ))/((sin(x)+cos(x))^( 4) )))^( n) dx}=? |

|
| prove that : I:= ∫_0 ^( ∞) (( x^( 3) )/(sinh ( x ))) dx = ((π^4 )/8) ■ m.n |
| Ω := ∫_0 ^( (π/2)) cos(2x).ln(sin(x))dx=^? −(π/4) solution (1 ) Ω := ∫_0 ^( (π/2)) ( 2cos^( 2) (x)−1)ln(sin(x))dx := 2∫_0 ^( (π/2)) cos^( 2) (x).ln(sin(x))dx−∫_0 ^( (π/2)) ln(sin(x))dx we know that : ∫_0 ^(π/2) ln(sin(x))dx=_(earlier) ^(derived) ((−π)/2) ln(2) ∫_0 ^( (π/2)) cos^( 2) (x).ln(sin(x))dx=_(posts) ^(previous) −(π/4)ln(2)−(π/8) ∴ Ω := −(π/2) ln(2) −(π/4) +(π/2) ln(2) ◂ Ω =− (π/4) ▶ m.n |
| find ∫((√(x^2 −9))/x^3 ) dx=? |

|
| ∫_0 ^(e−1) ∫_0 ^(e−x−1) ∫_0 ^(x+y+e) ((ln(z−x−y))/((x−e)(x+y−e)))dxdydz=? |
| ∫_0 ^1 ((ln(x^3 +1))/(x+1))dx |
| ∫_0 ^1 cot^(−1) (1−x+x^2 )dx |
| ∫_0 ^1 ((log (1+x))/(1+x^2 ))dx |
| ∫_(−π) ^π (sin^(75) x+x^(125) )dx=0 |
| ∫_(−1) ^1 e^x dx as limit of the sum |
| ∫_0 ^1 (3x^2 +2x+1)dx as the limit of sum |
| ∫_0 ^π (x^3 /(x^3 +(π−x)^3 ))dx=? |
| show that ∫_(−∞) ^( ∞) (1/( (√(x^2 +1)))) dx is unsolvable |
| prove that.. Ω =∫_0 ^( ∞) (( sin (x ))/(sinh(x)))dx =(π/2) tanh ((π/2)) |
| Q : If a , b are positive numbers and { (( a = 1 + (( 6a −2))^(1/3) )),(( b = 1 + (( 6b −2))^(1/3) )) :} then find the value of , a.b =? ... Compiled by m.n : (E lementary olympiad ). ■ |
| ∫^ x^x^x dx= |
| solve: I := ∫_0 ^( ∞) ((( tanh (x) )/x) )^( 2) dx = ? m.n. |
| ∫_(−∞) ^( ∞) (1/( (√(x^2 +1)))) dx |
| ∫_(−∞) ^( ∞) (((ln((x^(√(x^2 +1)) +1)^2 +1))^(−ln(x^2 +1)) )/( (√(x^(∣⌊x⌋∣) +1)))) dx |
| ∫_(−∞) ^( ∞) ((ln((√(x^4 +1))))/((ln(((√(x^2 +1)))^3 ))^2 )) dx |
| Prove that : Ω=∫_0 ^( 1) (( ln^( 3) (1 + x ))/x^( 2) )dx = (3/4) ζ (3 )− 2ln^( 3) ( 2 ) ■ Prepared by: M.N |
| Solve .......... Ω := ∫_0 ^( 1) x. sin( ln (x ))dx =^? ((−1)/( 5)) solution.... Ω :=^(i.b.p) [ (x^( 2) /2) . sin(ln(x))]_0 ^( 1) −(1/2)∫_0 ^( 1) x.cos( ln (x ))dx := ((−1)/2) ∫_0 ^( 1) x. cos (ln (x ))dx :=^(i.b.p) ((−1)/2) {[ (x^( 2) /2) cos (ln(x ))]_0 ^1 +(1/2) ∫x. sin(ln(x ))dx} := ((−1)/4) −(1/4) Ω (5/4) Ω = ((−1)/4) ⇒ Ω := ((−1)/( 5)) .........■ m.n ................................. |