
Question and Answers Forum
IntegrationQuestion and Answers: Page 66

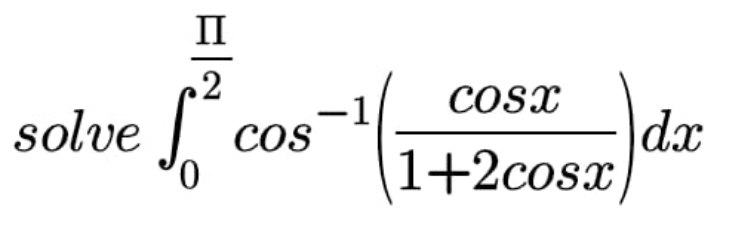
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 66 |

|
| ∫_0 ^( ∞) ((ln(x))/( (√(x^x +1)))) dx |
| ∫_0 ^( ∞) (1/( (√(e^x +1)))) dx |
| ∫_0 ^e (x/( (√(x−ln(x)))))dx |
| ∫_0 ^( ∞) ((ln(⌊x^2 ⌋!))/( (x^x +1)^x )) dx |
| ∫_0 ^( ∞) ((ln x)/( (√x) (√(x+1)) (√(2x+1)))) dx |
| ∫_0 ^( ∞) ((x−1)/( (√(2^x −1)) ln(2^x −1))) dx |
| ∫ (log x + 1)x^x dx |
| ∫_0 ^( 1) ((tan(x^2 +1))/(x^2 +1)) dx |
| ∫((xdx)/(x^4 +4x^2 +5)) |
| prove that... I:= ∫_0 ^( ∞) (( ln (x ))/(1+ e^( x) )) dx= ((−1)/2) ln^( 2) (2) ..■ |
| ∫_0 ^π ((xdx)/((a^2 cos^2 x+b^2 sin^2 x)^2 )) |
| ∫((x+2)/((x^2 +3x+3)(√(x+1))))dx |
| Express (1/(5×9)) in partial fraction |
| ∫_0 ^(π/2) (dx/((cos x+(√3) sin x)^2 ))dx=(1/( (√3))) |
| ∫_0 ^(π/2) (dx/(1+tan^5 x)) |
| ∫_0 ^(π/4) log (1+tan x)dx |
| ∫_0 ^∞ ((ln(x)arctg(x))/(x(x^2 +1)))dx |
| ∫ ((x^8 +1)/( (√(x^9 −2x^8 +1)))) dx ? |
| Ω = ∫_0 ^( ∞) (( ln ((1/x) ))/(1 +e^( 2x) )) dx =^? (3/2) ln^( 2) ( 2 ) m.n |
| find max(ℜ(I)+ℑ(I)) for the integral I = ∫_z ^( z+1) cos(cos(cos(x^(cos(cos(cos(x)))) )))dx z ∈ R |
| ∫_0 ^π (e^(cos x) /(e^(cos x) +e^(−cos x) ))dx |
| ∫_α ^β (dx/(x(√((x−α)(β−x) ))))=(π/( (√(αβ)))) where α,β >0 |
| Evaluate ∫_(−1) ^1 (((2x^(332) +x^(998) +4x^(1668) .sin x^(691) ))/(1+x^(666) ))dx |
| I = ∫_(x=a) ^( x=b) (√(u^2 + v^2 x^2 − 2uvwx)) dx = ? |
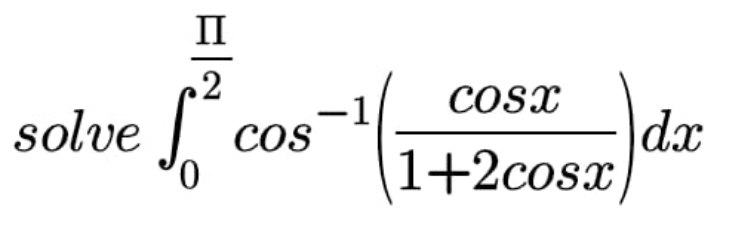
|