
Question and Answers Forum
IntegrationQuestion and Answers: Page 70

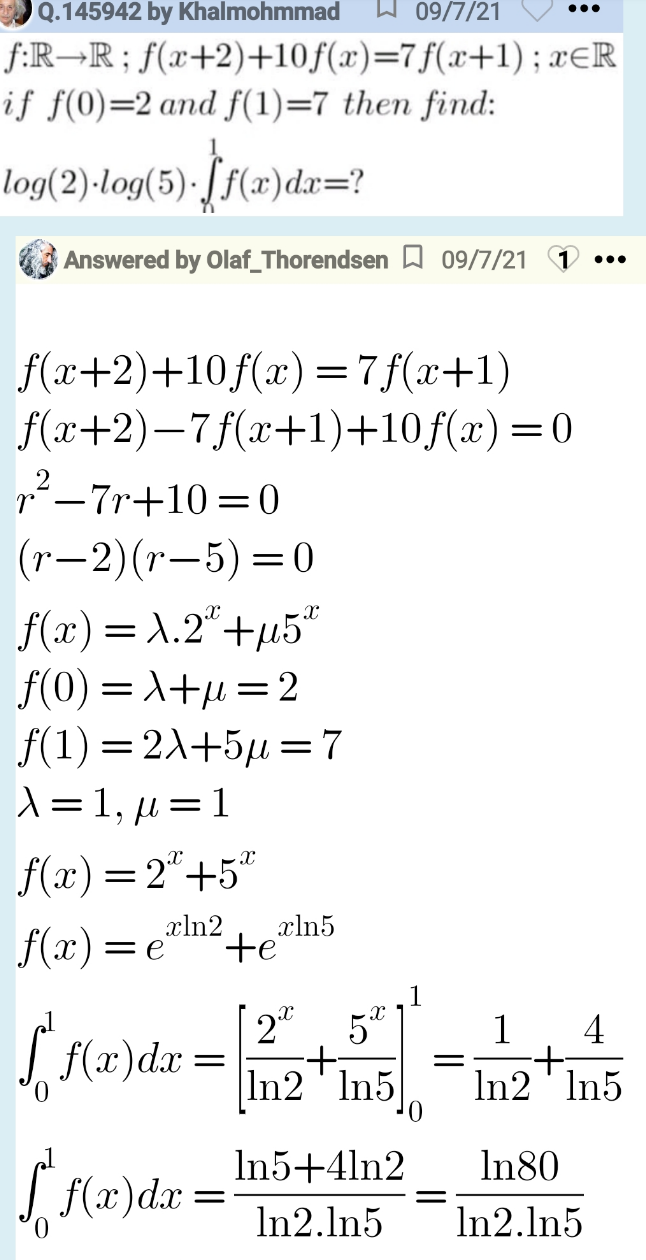
|
Question and Answers Forum |
IntegrationQuestion and Answers: Page 70 |
| Σ_(n=1) ^∞ (1/(ϕ^( n) F_n )) =? |
| calculate ∫_0 ^∞ (dx/((x^2 +3)^2 (x^2 +4)^2 )) |
| ∫(x/(1+cos^2 (x)))dx |
| ∫(1/x) e^(−(1/x^2 )) dx |
| Σ_(n=0) ^∞ (((−1)^n )/(n+1))(1+(1/3)+...+(1/(2n+1)))=? |
| ∫_( 0) ^( 1) t^2 + 1 dt |
| ∫_( 0) ^( 1) t^2 + (1/2)t −6dx |
| 1: S:= Σ_(n=1) ^∞ (((−1)^( n−1) )/(n.2^( n) )) =? 2: A:= Σ(((−1)^( n−1) )/(n^2 . 2^( n) )) =? |
| Σ_(n=1) ^∞ ((1+(1/2)+(1/3)+...+(1/n))/((n+1)(n+2)))=? |
| ∀t≥−1,F(t)=(2/π)∫_0 ^(π/2) (√(1+tcos^2 ϕ))dϕ 1) Show that ∀t≤−1 F(t)=(√(1+t))F(−(1/(1+t))) 2) show that if 0≤t_1 , 0≤F(t_2 )−F(t_1 )≤((t_2 −t_1 )/4) |
| ∫_0 ^π (a−e^(−ix) )^n (a−e^(ix) )^n cos(nx)dx |
| solve :: ( x ∈ R ) [ x ] = [ x^( 2) − x −6 ] note:: [x ] := max { q ∈ Z ∣ q ≤ x } |
| ∫ ((cos 5x+cos 4x)/(1−2cos 3x)) dx =? ∫ ((√(tan x))/(sin 2x)) dx =? ∫ (dx/( (√(cos^3 x sin^5 x)))) =? |
| ......nice ... ... ... calculus...... Ω= ∫_0 ^( 4) x^( 2) d (⌊x+⌊x +⌊x+⌊x⌋⌋⌋)=? |
| ∫ tan^4 x cos^2 x dx =? |

|
| Given that F(x) = ∫_0 ^x (t^2 /( (√(t^2 +1))))dt Show that F(x) is an increasing function |
| Σ_(n=1) ^∞ (n∙ln((2n+1)/(2n−1))−1)=? |
| find ∫(1/(x^n +1))dx for n∈N |
| S_n =Σ_(k=1) ^n (( 1)/(k (k+2)k+4))) lim_( n→∞) ( S_( n) ) = ? |
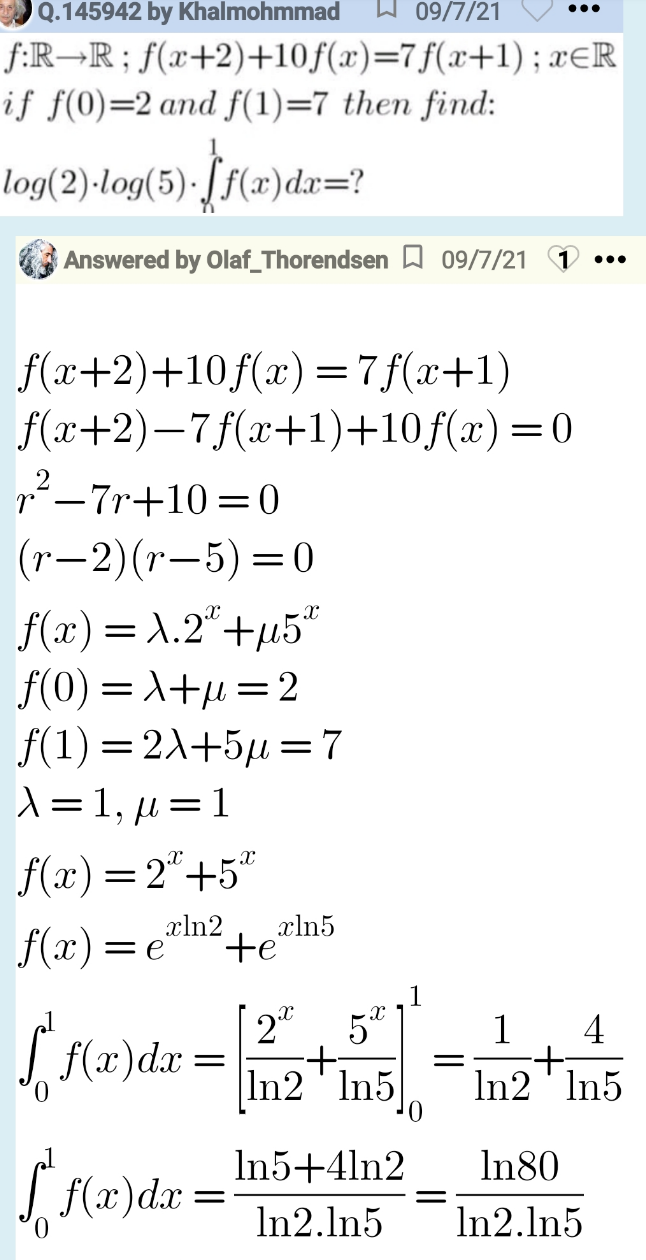
|
| Σ_(n=0) ^∞ (1/(2^( n) (n+1 ) ( n + 2 ))) =? |
| lim_(n→∞) (Arcsin(x))^( n) =0 ∴ x ∈ ? Q : mr liberty |
| Υ = ∫ (dx/(x^4 (√(x^2 −a^2 )))) =? |
| ∫((x+1)/(2x^2 +x+1))dx |
| Solve in Z[X] 1) XP ′ ≡ −1 mod(X^4 +1) 2) X^3 P −P ′ ≡ 1−X^2 mod(X^4 +1) 3) P^2 −X^3 P−X^2 ≡ 0 mod(X^2 +2) |