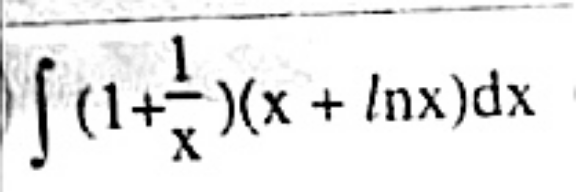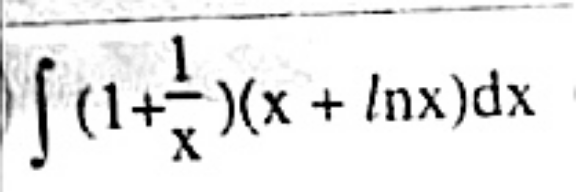Question and Answers Forum

All Questions Topic List

IntegrationQuestion and Answers: Page 71

Question Number 145378 Answers: 1 Comments: 0

∫_0 ^( ∞) ((√( 1+ x^4 )) −x^( 2) )dx=?

$\int_{0}^{\infty} (\sqrt{1 + x^{4}} - x^{2}) dx = ?$

Question Number 145361 Answers: 0 Comments: 0

∫_0 ^(+∞) ((t^2 +3t+3)/((t+1)^3 )) e^(−t) cos(t) dt

$\int_{0}^{+ \infty} \frac{t^{2} + 3 t + 3}{{(t + 1)}^{3}} e^{- t} \cos (t) dt$

Question Number 145358 Answers: 1 Comments: 0

Evaluate:: ∫_0 ^1 ln(1+x^2 )∙arctan(x)dx=?

$Evaluate :: \int_{0}^{1} \ln (1 + x^{2}) \cdot \arctan (x) dx = ?$

Question Number 145345 Answers: 1 Comments: 0

Σ_(n=1) ^∞ (((−1)^n n)/((2n+1)!))=?

$\underset{n = 1}{\sum^{\infty}} \frac{{(- 1)}^{n} n}{(2 n + 1)!} = ?$

Question Number 145339 Answers: 1 Comments: 0

Let f(x)=e^x cos x,Find Σ_(n=0) ^∞ ((f^((n)) (x))/2^n )=?

$Let f (x) = e^{x} \cos x, Find \underset{n = 0}{\sum^{\infty}} \frac{f^{(n)} (x)}{2^{n}} = ?$

Question Number 145319 Answers: 1 Comments: 0

# Calculus# Σ_(n=0) ^∞ (1/(n! + (n + 1 )!)) =?

$You can't use 'macro parameter character #' in math mode$ $\underset{n = 0}{\sum^{\infty}} \frac{1}{n! + (n + 1)!} = ?$

Question Number 145274 Answers: 1 Comments: 0

∫_(∣z∣=1) ((f^− (z))/(z−a))dz

$\int_{∣ z ∣= 1} \frac{\bar{f} (z)}{z - a} d z$

Question Number 145270 Answers: 1 Comments: 0

# Calculus ( I ) # Σ_(n=1) ^∞ Arccot(3 +((n ( n + 1))/3) )= ? .....

$You can't use 'macro parameter character #' in math mode$ $\underset{n = 1}{\sum^{\infty}} Arccot (3 + \frac{n (n + 1)}{3}) = ?$ $. . . . .$

Question Number 145202 Answers: 2 Comments: 1

Question Number 145200 Answers: 1 Comments: 0

evaluate:: Σ_(n=0) ^∞ (1/(n!(n^4 +n^2 +1)))=(e/2)

$evaluate :: \underset{n = 0}{\sum^{\infty}} \frac{1}{n! (n^{4} + n^{2} + 1)} = \frac{e}{2}$

Question Number 145184 Answers: 0 Comments: 0

find lim_(x→0) ((sin(sh(2x))−sh(sin(3x)))/x^2 )

$find \lim_{x \to 0} \frac{\sin (sh (2 x)) - sh (\sin (3 x))}{x^{2}}$

Question Number 145183 Answers: 2 Comments: 1

find ∫_0 ^1 (dx/(((√x)+(√(x+1)))^3 ))

$find \int_{0}^{1} \frac{dx}{{(\sqrt{x} + \sqrt{x + 1})}^{3}}$

Question Number 145081 Answers: 1 Comments: 0

∫_0 ^∞ (x−(x^3 /2)+(x^5 /(2∙4))−(x^7 /(2∙4∙6))+...)∙(1+(x^2 /2^2 )+(x^4 /(2^2 ∙4^2 ))+(x^6 /(2^2 ∙4^2 ∙6^2 ))+...)dx=(√e)

$\int_{0}^{\infty} (x - \frac{x^{3}}{2} + \frac{x^{5}}{2 \cdot 4} - \frac{x^{7}}{2 \cdot 4 \cdot 6} + . . .) \cdot (1 + \frac{x^{2}}{2^{2}} + \frac{x^{4}}{2^{2} \cdot 4^{2}} + \frac{x^{6}}{2^{2} \cdot 4^{2} \cdot 6^{2}} + . . .) dx = \sqrt{e}$

Question Number 145064 Answers: 1 Comments: 0

∫cos 2xln (1+tan x)dx

$\int \cos 2 xln (1 + \tan x) dx$

Question Number 145046 Answers: 0 Comments: 0

find ∫_0 ^∞ ∣cosx +sinx∣e^(−x) dx

$find \int_{0}^{\infty} ∣ cosx + sinx ∣ e^{- x} dx$

Question Number 145044 Answers: 3 Comments: 0

calculate ∫_0 ^∞ e^(−2x) (√(1+sinx))dx

$calculate \int_{0}^{\infty} e^{- 2 x} \sqrt{1 + sinx} dx$

Question Number 144997 Answers: 0 Comments: 0

∫_0 ^1 ((ln(1+x)ln(1+x^2 ))/x)dx=(π/2)G−((33)/(32))ζ(3)

$\int_{0}^{1} \frac{\ln (1 + x) \ln (1 + x^{2})}{x} dx = \frac{π}{2} G - \frac{33}{32} ζ (3)$

Question Number 144985 Answers: 1 Comments: 0

∫ (((2+(√x)))/((x+1+(√x))^2 )) dx =?

$\int \frac{(2 + \sqrt{x})}{{(x + 1 + \sqrt{x})}^{2}} dx = ?$

Question Number 144975 Answers: 3 Comments: 0

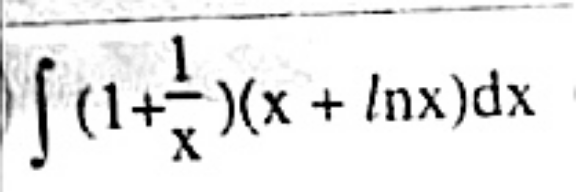
Question Number 144939 Answers: 0 Comments: 0

resoudre I=∫_0 ^(π/2) tan(nx)tan^n (x)dx

$resoudre$ $I = \int_{0}^{\frac{π}{2}} \tan (nx) \tan^{n} (x) dx$

Question Number 144926 Answers: 1 Comments: 0

∫_0 ^∞ x^n (e^(ix) )^z dx=??? (z∈C)

$\int_{0}^{\infty} x^{n} {(e^{i x})}^{z} d x = ? ? ? (z \in C)$

Question Number 144925 Answers: 1 Comments: 0

Σ_(n=1) ^∞ (((2n)!!)/(2^n ∙(n+1)∙(2n+1)!!))=?

$\underset{n = 1}{\sum^{\infty}} \frac{(2 n)!!}{2^{n} \cdot (n + 1) \cdot (2 n + 1)!!} = ?$

Question Number 144924 Answers: 1 Comments: 0

S(x)=Σ_(n=1) ^∞ (((2n)!!)/((2n+1)!!))x^(2n) =?........(∣x∣<1)

$S (x) = \underset{n = 1}{\sum^{\infty}} \frac{(2 n)!!}{(2 n + 1)!!} x^{2 n} = ? . . . . . . . . (∣ x ∣< 1)$

Question Number 144909 Answers: 1 Comments: 0

Γ(a+ib) doesn′t exist ? give her value

$Γ (a + i b) {d o e s n}^{'} t e x i s t ? g i v e h e r v a l u e$

Question Number 144896 Answers: 1 Comments: 0

Evaluate ∫e^x (((1−x)/(1+x^2 )))^2 dx

$Evaluate$ $\int e^{x} {(\frac{1 - x}{1 + x^{2}})}^{2} d x$

Question Number 144905 Answers: 0 Comments: 1

Pg 66 Pg 67 Pg 68 Pg 69 Pg 70 Pg 71 Pg 72 Pg 73 Pg 74 Pg 75

Contact: info@tinkutara.com