
Question and Answers Forum
LimitsQuestion and Answers: Page 6
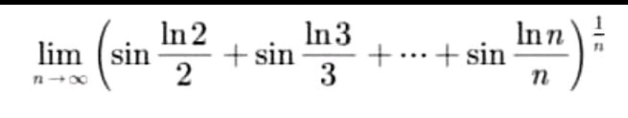
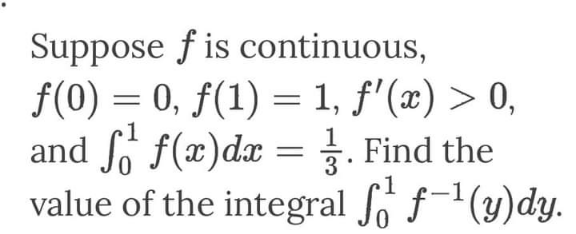




|
Question and Answers Forum |
LimitsQuestion and Answers: Page 6 |
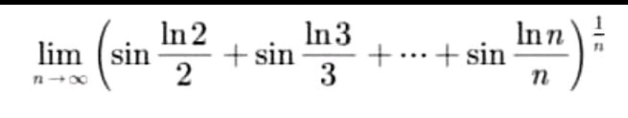
|
| lim_(x→∞) sin (√(x+1))−sin (√x) =? |
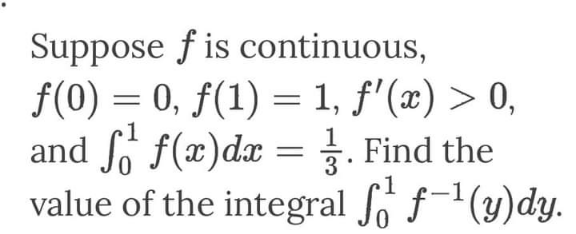
|
| find lim_(x→∞) sin (((πx)/(5+3x))) by sequeeze theorem |
| lim_(x→0) (((2^x +3^x +5^x )/3))^(3/x) =? |
| lim_(x→0) ((1/(ln(1+x) ))−(1/(ln(x+(√(1+x^2 )) )))) = ?? |
| A point moves on the curve of the function f(x)=(√(x^2 +5)) such that it′s x−coordinate increases at a rate of 3(√(10)) cm/s. Find the rate of change of it′s distance from the point (1,0) when x = 2 |
| calculate... Q : lim_( x→ (π/4)) ( 1 + sin(x) −cos(x) )^( tan(2x)) = ? • Nice − Mathematics • |
| lim_(x→0) ((tan ((x/2))−sin ((x/2)))/(x^2 ((√(x^2 +x−2))−(√(x^2 +2x−2)) ))) =? |

|

|
| lim_(x→0) ((4e^(3x) −9e^(2x) +6x+5)/x^3 )=? |
| lim_(n→∞) ∫_0 ^(√n) (1−(x^2 /n))^n dx = ??? |

|
| lim_(x→∞) ((log n)/n)^( n) (√(Σ_(k=1) ^∞ (k^n /(k!)))) = ???? |
| lim_(x→0) ((sin 3x)/(tan 6x)) = ....? |
| lim sin(x) = ? x→+∞ |
| lim_(x→(π/(2n))) ((√((sin 2nx)/(1+cos nx)))/(4n^2 x^2 −π^2 )) =? |
| a_(n+2) = (√(a_n ×a_(n+1) )) ∀ n≥1 , n ∈ N and here a_(1 ) = α and a_2 = β then prove that lim_(n→∞) a_(n+2) = (α×β^2 )^(1/3) |
| lim_(x→∞) (1/( (√(4025x)))) ((1/( (√x))) +(1/( (√(x+1)))) +(1/( (√(x+2)) )) +... +(1/( (√(4025x)))) )=? |
| lim_(x→0) ((x−ln(x+(√(1+x^2 ))))/x^3 )=? with out L′Hospital rul |
| calculate… L = lim _(n→∞) (( (1+(1/2) )(1+(1/3))… (1+(1/n))))^(1/n) = ? |
| Soit I=∫^( 1) _( 0) (√(t(√(t(1−t)))))dt Comment calculer I |
| lim_(x→∞) ((2+(√(cosx)))/(−1+(√(cosx))))=? |
| ∫_0 ^(π/2) (lim_(n→∞) nsin^(2n+1) x cos x)dx = ? |

|