
Question and Answers Forum
LogarithmsQuestion and Answers: Page 13
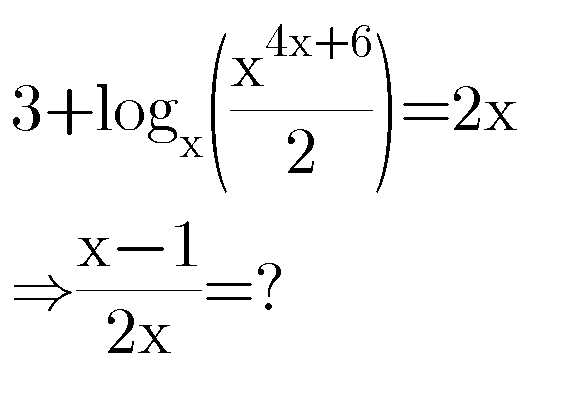



|
Question and Answers Forum |
LogarithmsQuestion and Answers: Page 13 |
| 5^(lnx) = 50 −x^(ln 5) |
| log_2 (log_3 (log_2 (2/x))−1) < 1 has solution (1/a^(26) )<x<b. find a ? |
| ((log_( 0,2) (x−2))/((4^x −8)(∣x∣−5))) ≥ 0 |
| If (log_(10) (ax))(log_(10) (bx))=−1 find x in term a and b |
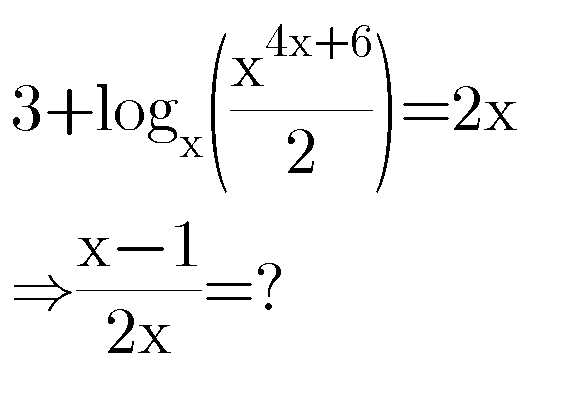
|
| 4^(2x−1) +(1/4)^2 log^2 (2x)>^2 log(x) {^2 log((1/x))−2^(2x) } |
| Solve for x ((8^x + 27^x )/(12^x + 18^x )) = (7/6) |
| 53^(log_x (7)) = (√x) x = ? |

|
| evaluate 5^(√(log 7_5 )) − 7^(√(log 5_7 )) |

|
| prove that e^(lnx) = x or a^(log_a x) = x |
| ln (e+ln (e+ln (e+...)))=? |
| If log_x y = 6 & log_(14x) 8y = 3 then find the value of x & y. |
| If, log x^y = 6 and log 14x^(8y) = 3 then find the value of x, y. |
| Find the value of x. log_8 x + log_4 x + log_2 x = 11. |
| Identify domain and range of this function that f(x)= ln((4−x)/(4+x)). |

|
| given that x and y are two numbers other one. given that a>0 and b>0 and a^x = b^y = (ab)^(xy) show that x + y =0 |
| solve for x the following equations a) log x^3 − 2log x^2 + 2log x + 2log (√x) = 3 b) log_x 24 −3log_x 4 + 2log_x 3 =−3 |
| given that a,b and c are positive numbers other than 1 , show that log_b a × log_c b × log_a c = 1 hence, evaluate log_(10) 25 × log_2 10 × log_5 4 |
| solve for x and y the simultaneous equation log_3 x = y = log(2x − 1) |
| Seja 53^(log_(1/(√e^𝛑 )) [(((x+11)!))^(1/(9999999)) ]) = 1. Calcule (x_1 /x_2 )+0,9. |
| (√(8+log_6 (x!)))+(√(17−log_(x!) (6))) = 7 |
| Value of x satiesfied y=((log_4 (x^2 −1))/(4x^2 +2x+1)) negative value is... a. −1<x<(√2) b. −(√2)<x<1 c. −(√2)<x<(√2) d. −(√2)<x<−1 e. x<−2 |
| If 2x+y=8 and (x+y)=(3/2)log_(10) 2.log_8 36 then x^2 +3y=... a. 28 b. 22 c. 20 d. 16 e. 12 |