
Question and Answers Forum
LogarithmsQuestion and Answers: Page 14

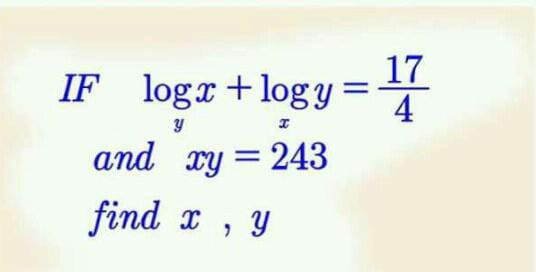



|
Question and Answers Forum |
LogarithmsQuestion and Answers: Page 14 |
| Value of maximum f(x)=^2 log(x+5)+^2 log(3−x) is... a.4 b.8 c. 12 d. 15 e. 16 |
| If ((log_2 a)/(log_3 b))=m and ((log_3 a)/(log_2 b))=n a>1 and b>1 then (m/n)=... a.log_2 3 b. log_3 2 c. log_4 9 d. (log_2 3)^2 e. (log_3 2)^2 |
| If (a^2 /b^2 )=12 then log(^3 (√(b/a)))=.. a. −2 b. −1 c. 0 d. 1 e. 2 |
| log_5 (√(27))×log_9 125+log_(16) 12=... a. ((61)/(36)) b. (9/4) c. ((61)/(20)) d. ((41)/(12)) e. (7/2) |
| log_7 2=a log_2 3=b log_6 98=... |
| (((log_6 36)^2 −(log_3 4)^2 )/(log_3 ((√(12)))))=... |
| If t=((x^2 −3)/(3x+7)) then log(1−∣t∣) can to find for a. 2<x<6 b.− 2<x<5 c.− 2≤x≤6 d. x≤−2 or x>6 e. x<−1 or x>3 |
| If xyz ≠ 0 and x+y+z=0 a=10^z b=10^y c=10^x then a^(((1/y)+(1/z))) . b^(((1/z)+(1/x))) .c^(((1/x)+(1/y))) =... a. 0.001 b. 0.01 c. 0.1 d. 1 e. 10 |

|
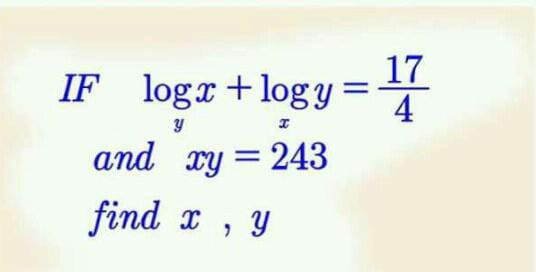
|

|
| 5log_(4(√2)) (3−(√6) ) −6log_8 ((√3)−(√2)) |
| Σ_(1°) ^(89°) log_2 tan r° |
| Solve for x: x^(log_3 2) = (√x) + 1 |
| Given that((log(3x+1)^(2x−1) )/(log(3x+1)))=5,find the value of x. |
| Given that 1+log_3 x =log_(27) y, express y in terms of x. |

|
| given that log2=0.3010 log3=0.477 log5=0.699 find the values of log(√((0.2))) |

|
| 5^(x+2) −95^x =2^(x+9) +1132^x |
| a) if f(x)=log(x+2), solve the equation: 2^(f(x−2)) ×2^(f(2x+2)) =4^(logf(x)) |
| simplify ((log_3 64 × log_4 243)/(log_2 16)) |
| how can I get the x? a)3^x +4^x =5^x b)7^(6−x) =x+2 c)((√(2+(√3))))^x +((√(2−(√3))))^x =2^x d)3^(x−2) =(9/x) |
| Plz solve log (17.92)^(−(1/9)) |

|
| mag∫2x+x^3 = |