
Question and Answers Forum
LogarithmsQuestion and Answers: Page 6


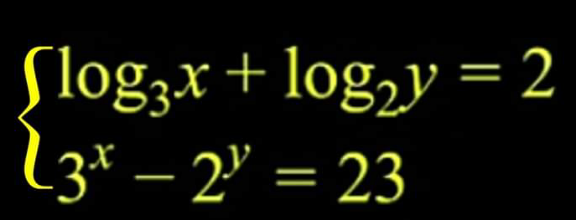






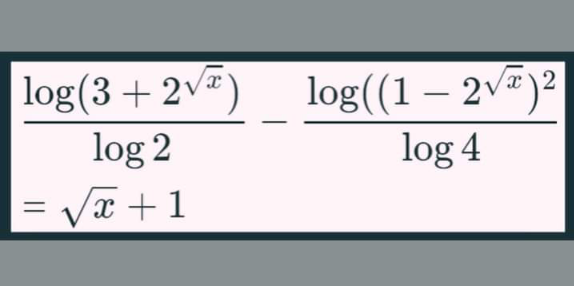



|
Question and Answers Forum |
LogarithmsQuestion and Answers: Page 6 |
| ((x−1)/(log _3 (9−3^x )−3)) ≤ 1 |

|

|
| In order to monitor buses in a travel agency, the manager decides to monitor the number of break downs of the buses using the sequence {x_n } defined by x_(n+1) = 1.05 x_n + 4. Given that x_0 = 40. is the number of break downs by the buses from the 1^(st) of january 2000, and that for every n∈N, we denote x_n the number of breakdowns of the buses as from 1^(st) of january of the year (2000 + n) (a) Calculate x_1 , x_2 , x_3 (b) Consider the sequence {y_n } defined by y_n = x_n + 80 for all n ∈ N (i) express y_(n+1) in terms of y_n and deduce the nature of the sequence {y_n }. (ii) Express y_n in terms of n. deduce x_n in terms of n (iv) find the number of break downs that will be registered by 1^(st) january 2021. |
| log _5 ((√(x−9)))−log _5 (3x^2 −12)−log _5 ((√(2x−1))) ≤ 0 |
| (1+log _3 x).(√(log _(3x) ((x/3))^(1/3) )) ≤ 2 |
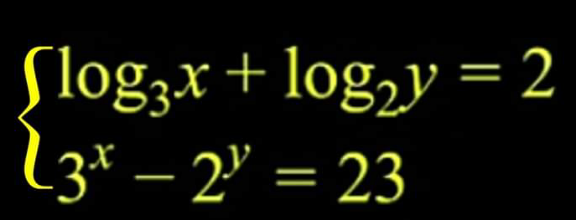
|

|
| x_1 and x_2 is root log_2 x^((1+^2 log x)) =2, the value is x_1 +x_2 = ... a. 2(1/4) b. 2(1/2) c. 4(1/4) d. 4(1/2) e. 6(1/4) |

|
| the n^(th) term of 1 , 2, 6, 24, 120 ........ is? |

|

|

|
| log _e (x)+log _x (e)+log _(((e/x))) (x)=(5/2) x=? |
| 24^(log _(10) (x)) −26^(log _(10) (x)) =1 x=? |
| ∫(dx/(x^2 +2x+2(√(x^2 +2x−4)))) |

|
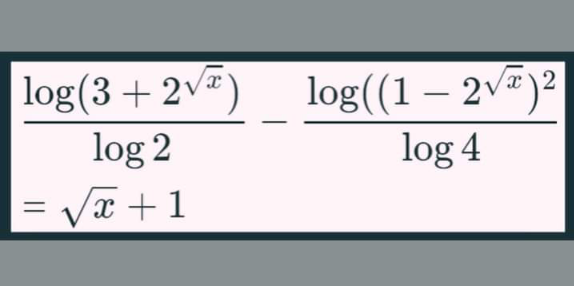
|
| 1+(√3^x )=2^x x=? |
| (((3+2(√2))^(2008) )/((7+5(√2))^(1338) )) + (3−2(√2)) = log _2 (x) x=? |

|
| calculer la differentielle de y=log(x) teste: sachant que log(35)=1,54407, calculer log(3501) NB: on rappelle que (1/(log(10)))=log(e)=0,43429.. |

|

|
| find the remainder when 10^(10^n ) divides 7 |