
Question and Answers Forum
Matrices and DeterminantsQuestion and Answers: Page 10




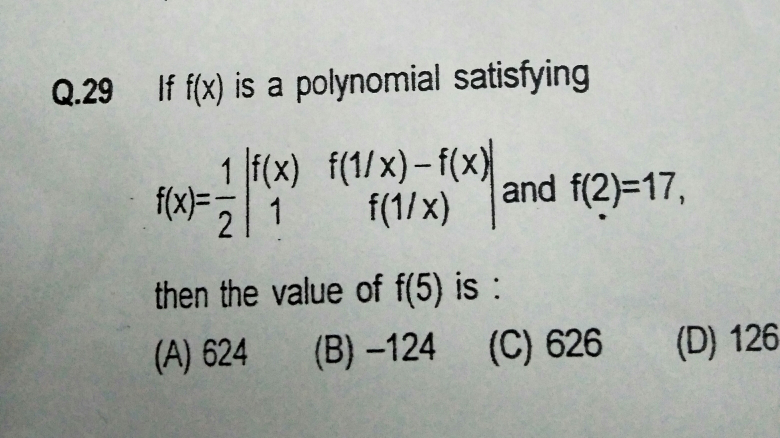

|
Question and Answers Forum |
Matrices and DeterminantsQuestion and Answers: Page 10 |
| let give A= (((1 (α/n))),((−(α/n) 1)) ) with n ∈N^∗ and α∈R find lim_(n→+∞) A^n . |
| let give the matrice ( 0 cosθ cos(2θ)) A= ( cosθ 0 cos(2θ) ) ( cos(θ) cos(2θ 0 ) and D_θ =det A solve inside R D_θ =0 |
| let give ( 1 1 −1) A= ( 1 1 1 ) ( −1 1 1 ) and the matrices I= ( 1 0 0 ) ( 0 1 1 ) ( 0 0 1 ) and J= ( 0 1 −1) ( 1 0 1). ( −1 1 0) 1) find J^2 and J^(−1) . 2) let put J^n = x_n I +y_n J .prove that x_(n+2 ) +x_(n+1) −2x_n =0 3) calculate J^n and A^n . |
| let give A= ( cosθ −sinθ ) ( sinθ cosθ ) 1) calculate^t A. A .prove that A is inversible and find A^(−1) 2) find A^n for n∈ N 3) developp (A +A^(−1) )^n then prove that 2^n cos^n θ = Σ_(k=0) ^n C_n ^k (n−2k)θ and Σ_(k=0) ^n C_n ^n sin(n−2k)θ =0 . |
| let give A = ( 1 1 ) ( 2 −1) find e^(A ) and e^(−tA) . |
| let give ( 2 3 −3) A = ( −1 0 1) ( −1 1 0 ) find a diagoal matrice D and a inversible matrice P wich verify A = P.D.P^(−1) and calculate A^n . |
| let give p=(_(1 −2) ^(1 −1) ) and D= (_(3 −6) ^(2 −2) ) calculate A= p.D.p^(−1) . |
| let give A= _( () −1 1 1) ( 1 −1 1) find A^n for n integr. ( 1 1 −1) |

|
| let give A=(_(2 2) ^(1 2) ) find A^n and e^A and e^(tA) . we remind that e^A = Σ_ (A^n /(n!)) |
| (x/3)+2x=14 |

|
| solve for A and B if 2A+B [((6 3)),((6 −2)) ] and 3A+2B [((1 0)),((0 5)) ] |
| Let matrice A = ((a,b),(c,d) ), and A^T = A^(−1) Find d − bc |

|

|
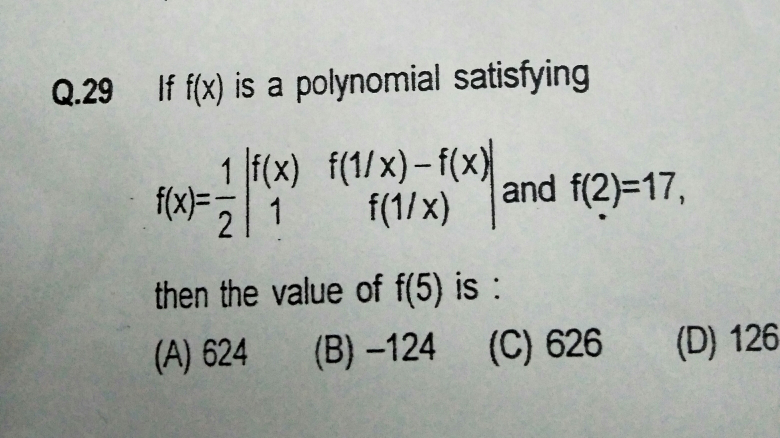
|
| (((5 3)),((3 2)) )A + (((2 5)),((5 1)) ) = (((4 7)),((6 2)) ) Find ∣4A^(−1) ∣ |
| equivalent matrices are obtained by |
| Let A and B is 3×3 matrix of equal number where A=symmetric matrix ....B=skew symmetric matrix and the relation... (A+B)(A−B)=(A−B)(A+B) then..the value of.. ... k (AB)^T =(−1)^k (AB) (a) −1 (c) 2 (b) 1 (d) 3 |
| A matrix has N rows and 2k−1 columns. Each column is filled with M ones and N−M zeros. A given row j is “cool” if and only if Σ_(i=1) ^(2k−1) a_(ji) ≥ k. Find the minimum and the maximum number of cool rows for given N, k and M. |

|
| how much matrices of integers number A= [(a,b),(c,d) ]if A^2 +A=2I, c=0, det(A)=4 |
| A∈M_(n×n) A^2 =A (I+A)^(−1) =....??? |
| A∈M_(2016×2016) with the entries a_(ij) {_(0, if i+j≠2016) ^(1, if i+j=2016) find the determinant?? |
| reduce the matrix below to echelon form and then to row canonical form A = [((2 4 2 −2 5 1)),((3 6 2 2 0 4)),((4 8 2 6 −5 7)) ] |