
Question and Answers Forum
Matrices and DeterminantsQuestion and Answers: Page 3




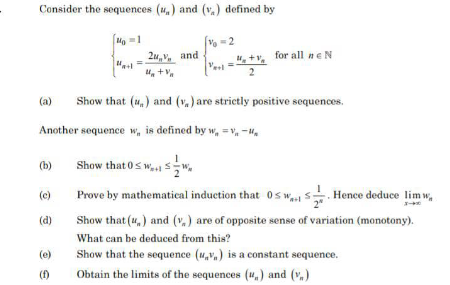






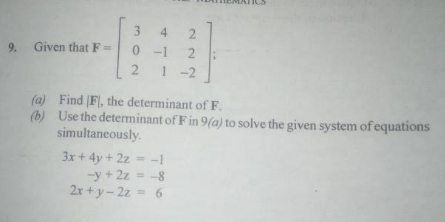

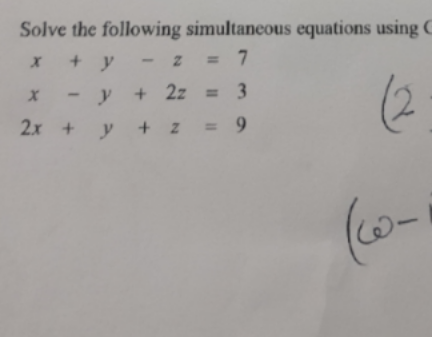

|
Question and Answers Forum |
Matrices and DeterminantsQuestion and Answers: Page 3 |
| Prove that determinant ((1,1,1),(a,b,c),(a^2 ,b^2 ,c^2 ))= (a−b)(b−c)(c−a) |

|

|

|
| find x: (log_(10) x)^2 −log_(10) x=0 |
| I_n = −((2n)/(2n + 1)) I_(n−1) I_0 = 1 Show that I_n = (((−4)^n (n!)^2 )/((2n+1)!)) |
| When A^(−1) = [(3,1),(8,4) ] find the A=? ,∣A^(−1) ∣∙A=? |
| 2. [((1 2)),((2 −1)) ] Soln: [((1 2)),((2 −1)) ]= [((1 0)),((0 1)) ].A ⇒ [((1 2)),((0 −5)) ]= [(( 1 0)),((−2 1)) ].A [R_2 →R_2 −2R_1 ] ⇒ [((1 2)),((0 1)) ]= [(( 1 0)),(((2/5) −(1/5))) ].A [R_2 →(−(1/5))R_2 ] ⇒ [((1 0)),((0 1)) ]= [(((1/5) (2/5))),(((2/5) −(1/5))) ].A [R_1 →R_1 −2R_2 ] ∴A^(−1) =(1/5) [((1 2)),((2 −1)) ] |
| A point in rectangular coordinates (x,y,z) can be represented in spherical coordinates (r,θ,ϕ) by: x = r sin θ sin ϕ, y = sin θ sin ϕ, z = sin ϕ, 0 ≤ θ ≤ 2π , 0 ≤ ϕ ≤ π (a) Calculate the Jacobian of the transformation ((∂(x,y,z))/(∂(r,θ,ϕ))) (b) Calculate the volume of the region delimited by the sphere: S = {x,y,z ∈R^3 , x^2 +y^2 +z^2 ≤ R^2 , R>0} |

|

|
| Given A= ((((1/2) (1/2))),(( a b)) ) . If A^3 = A^2 then 2a−3b=? |

|

|

|
| help me ! { ((x+3y+z=2)),((−3x+4y+2z=3)),((−2x+7y+3z=5)) :} Gauss Method... |
| find an explicit formula for the sequence (2/3), (4/5), (8/9), ((16)/(17)), ((32)/(33)), ... |

|
| If A and B are invertible matrices,then: (AB)^(−1) =B^(−1) A^(−1) ≠ A^(−1) B^(−1) proove. |

|

|
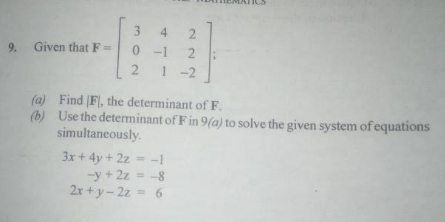
|
| let D= [((v 5)),(((1/3) m)) ] find number (v) and (m) such that D^2 =5I (I=identity matrix) |

|
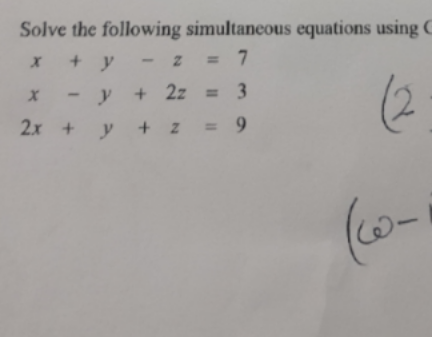
|

|