
Question and Answers Forum
Operation ResearchQuestion and Answers: Page 3

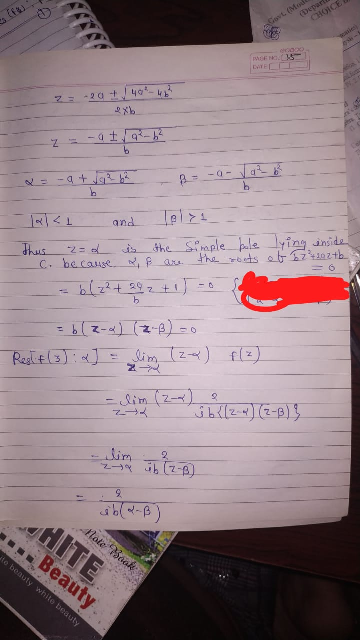





|
Question and Answers Forum |
Operation ResearchQuestion and Answers: Page 3 |
| how do you solve (D^3 +12D^2 +36D)y=0 by constant coefficients |
| (dy/dx) − y.tan x = e^x .sec x |
| i^i^(i.∞) =? |
| Solve x^2 y′′−3xy′−5y=0 |
| xy′ + y^2 = x^2 e^x |
| prove by contradiction that (√(2 )) is irrational. |

|
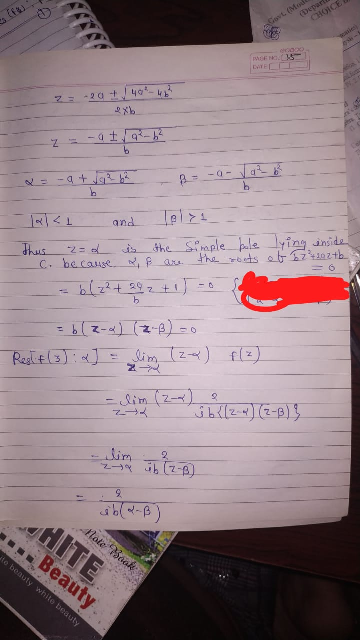
|

|

|
| what operation on interger used in 9(7.8)=(9.7).8?? |
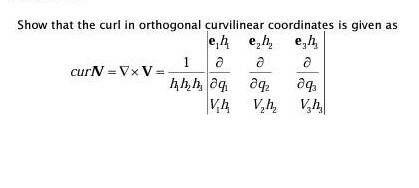
| A particle P, moves on the curve with polar equation r = e^(kθ) , where (r,θ) are polar coordinates referred to a fixed pole and k is a positive constant. Given that the radial velocity of P is (k/r) show that the transverse acceleration of th particle is zero. |
| the force acting on a particle P of mass 2kg is (2ti +4j)N. P is initially at rest at point with position vector (i+2j). Find the velocity of P when t=2 and the position vector when t=2. |
| the force acting on a particle P of mass 2kg is (2ti +4j)N. P is initially at rest at point with position vector (i+2j). Find the velocity of P when t=2 and the position vector when t=2. |
| the force acting on a particle P of mass 2kg is (2ti +4j)N. P is initially at rest at point with position vector (i+2j). Find the velocity of P when t=2 and the position vector when t=2. |

|

|

|

|

|
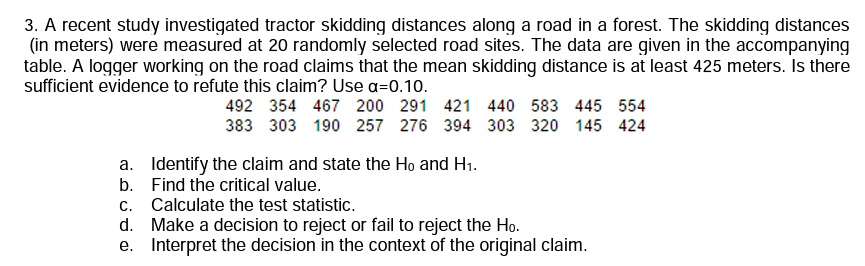
| The n^(th) term of a progression is np+q and the sum of n terms is denoted by S_n . Given that the 6^(th) term is 4 times 2^(nd) term and that S_3 =12, find the value of p and q. Express S_n in terms of n. |
| x and y are two binary numbers which are in 4 − bit 2′s complement formate, where x = 00102 and y = 11012 : clearly, y is a negative number. what is the result of x + y in decimal formate. |
| Develop an algorithm and draw a flowchat to find the sum of numbers from 1 − 100 |
| gn |
| =7↽ |
| f:[0,+1]→R η(f):=∫_0 ^1 f^2 dx and if ∀x∈[0,1],f∈[m,M] for some (m,M)∈R^2 f↑:=max(f)−f f↓:=f−min(f) μ(f):=∫_0 ^1 f↓f↑dx then f=e^x , η(f)=^? μ(f) f=x,η(f)=^? μ(f) ∃f,η(f)=0??? ∃f,μ(f)=0??? μ(f)=0, does η(f)=0??? η(f)=0, does μ(f)=0??? |