
Question and Answers Forum
OthersQuestion and Answers: Page 118

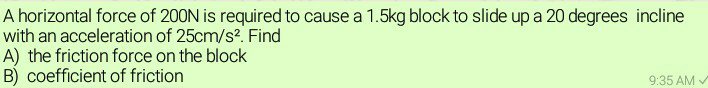
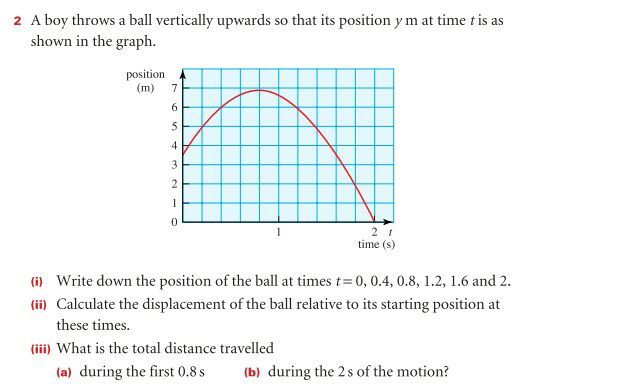

Pg 113 Pg 114 Pg 115 Pg 116 Pg 117 Pg 118 Pg 119 Pg 120 Pg 121 Pg 122
|
Question and Answers Forum |
OthersQuestion and Answers: Page 118 |
| Calculate Σ_(j≤k≤i) (−1)^k ((i),(k) ) ((k),(j) ) |
| Find the value of : Σ_(i=0) ^∞ Σ_(j=0) ^∞ Σ_(k=0) ^∞ (1/(3^i 3^j 3^k )). case 1: i≠j≠k. case 2: i<j<k. |
| let p_n (x) polinom Maclaurin for function f(x)=e^x . How many degree minimal polinom (n) so ∣e^x −p_n (x)∣≤ 10^(−2) , for −1≤x≤1? |
| let f diferensiabel on continues x=a and f(a)≠ 0 lim_(n→∞) [((f(a+(1/n)))/(f(a)))]^n value is ? |
| how to read this ⟨x⟩. p |
| (a,(1/a)),(b,(1/b)),(c,(1/c)),(d,(1/d)) are four distinct points on a circle of radius is 4 units then abcd is equal to ? |
| ∫((x^3 +x+1)/(x^2 +1)) dx |
| find f(x)= ∫_0 ^1 ln(1+xt^2 )dt with x>0. 2) give thevalue of ∫_0 ^1 ln(1+t^2 )dt and ∫_0 ^1 ln(1+2t^2 )dt. |

|
| Deduce the power series of sin^2 x. Hence show that if x is small then (sin^2 x − x^2 cosx)/x^4 =(1/6) − (x^2 /(360)) |
| let x={(1/n)}_(n=1) ^∞ and y={(1/(n+1))}_(n=1) ^∞ be a sequence of real numbers and l_(2 ) ={x=(x_1 ,x_2 ,x_3 ,...):Σ_(n=1) ^∞ ∣xi∣^2 <∞} a linear space. (1) verify that x and y are in l_2 . (2) compute the inner product of x and y on l_2 please help me solve this question. |
| Complete the square in y^2 +8y+9k and hence find the value of k that makes it a perfect square. |
| find three nos in AP whose product is equal to the square of their sum. |
| calculate ∫∫_(x^2 +y^2 −2x≤0) xdxdy. |
| solve (√(1+tan^2 x/1+cot^2 x= tanx)) |
| let I_n = ∫_0 ^(π/4) (dx/(cos^(2n+1) )) (n∈N) 1) find a and b fromR /∀x∈[0,(π/4)] (1/(cosx))=((acosx)/(1−sinx)) +((bcosx)/(1+sinx)) .find I_0 2) verify the relation (1/(cos^(2n+3) x))=(1/(cos^(2n+1) x)) +((sinx sinx)/(cos^(2n+3) )) .find the relation of recurrence between I_n and I_(n+1) . |
| find I= ∫_(1/2) ^1 arctan((√(1−x^2 )) dx . |
| find Σ_(n=0) ^∞ (x^n /(3n+2)) for ∣x∣<1 then find Σ_(n=0) ^∞ (((−1)^n )/((3n+2)2^n )) . |
| find the elements of the ellipse given the following equation 1.(x^2 /(25))+(y^2 /4)=1 2.x^2 +9y^2 =9 |

|
| 32^(32^(32) ) /7...find remainder |
| i^(i ) what is iota to power iota |
| How many arrangements are there of 4 letters chosen from the word COMMUNICATION |
| Prove the convergence of each of the following sequence (i) {((n − 1)/n)}_(n = 1) ^∞ (ii) {(1/(2)^(1/n) )}_(n = 1) ^∞ (iii) {((n + 1)/n)}_(n = 1) ^∞ |

|

|
Pg 113 Pg 114 Pg 115 Pg 116 Pg 117 Pg 118 Pg 119 Pg 120 Pg 121 Pg 122 |