
Question and Answers Forum
OthersQuestion and Answers: Page 50


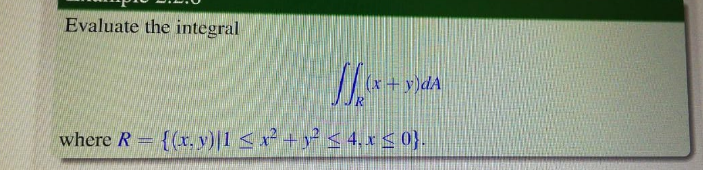

|
Question and Answers Forum |
OthersQuestion and Answers: Page 50 |
| Σ_(n=1) ^∞ ((coth(n))/n^3 ) |
| 1−5((1/2))^3 +9((1/2).(3/4))^3 −13((1/2).(3/4).(5/6))^3 +..=(2/π) (prove) |
| ∫_0 ^a e^(−x^2 ) dx=((√π)/2)−(e^(−a^2 ) /(2a+(1/(a+(2/(2a+(3/(a+(4/(2a+...)))))))))) (Prove) |
| Σ_(n=1) ^∞ (1/(e^(−φn) +((e^(2πn) −e^(−2φn) )/(2e^(−φn) +((e^(2πn) −e^(−2φn) )/(2e^(−φn) +((e^(2πn) −e^(−2φn) )/(2e^(−2φn) ...)))))))) |

|
| Σ_(n=1) ^∞ (n^7 /7^n ) |
| (1/(1!))+((1!^2 )/(3!))+((2!^2 )/(5!))+((3!^2 )/(7!))+((4!^2 )/(9!))+.... |
| to Tinku tara equation editor is not available in playstore now...pls check..i suggested a few students to dowmload it |
| Merry christmas !! 🎅🤶☃️🌄🎄🦌 🔔🔔🔔🔔🔔🔔🔔🔔🔔 🎄🎄🎄🎄🎄🎄🎄🎄 ∫_0 ^(1/2) ((tanh^(−1) x)/( (x)^(1/5) ))dx |

|
| ((e^π −1)/(e^π +1))=(π/(2+(π^2 /(6+(π^2 /(10+(π^2 /(14+....)))))))) |
| prove that ∣z∣ > ((∣Re(z)∣ +∣Im(z)∣)/2) , ∀z∈C |
| Σ_(n=1) ^∞ (H_n ^2 /n^4 ) |
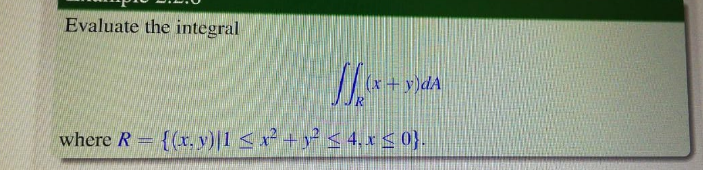
|
| If a_n =6^n +8^n find (a_(1991) /(49)). |
| (1/1^2 )−(1/2^3 )+(1/3^5 )−(1/4^7 )+(1/5^(11) )−(1/6^(13) )+.... |
| A particle starts from rest at time t = 0 and moves in a straightline with variable acceleration a m/s^2 where a = (t/5) , 0 ≤ t ≤ 5 , a = (t/5) + ((10)/t^2 ) , t ≥ 5, t being measured in seconds. Show that the velocity is 22(1/2) m/s when t = 5 and 11 m/s when t = 10. Show also that the distance travelled by the particle in the first 10 seconds is (43(1/3)−10 ln 2) m. |
| ((Σ_(n=0) ^∞ e^(−n^2 ) )/(Σ_(n=0) ^∞ e^(−2n^2 ) )) |
| ((i!)/(π!)) (Exact form) |
| ∫_0 ^1 ((cos2x−tanx.cot(tanx))/(sin2x−tan(tanx)log(cos^2 x)))dx |
| Σ_(n=0) ^∞ ((((√5)−2)^n (((2n)),(n) ))/(((2n+1)(((√5)+(1/( (√5))))^(n+(1/2)) +((√5)−(1/( (√5))))^(n−(1/2)) )))) |
| 1+4((1/2))^7 +7(((1.3)/(2.4)))^7 +10(((1.3.5)/(2.4.6)))^7 +... |
| ∫_0 ^∞ ((√x)/(1−x^2 )).(1/(e^(2πx) −1))dx |
| ∫_0 ^∞ ((1−tanhx)/( ((tanhx))^(1/5) ))dx |

|
| ((((1/(1!)))^2 −((1/(2!)))^2 +((1/(3!)))^2 −((1/(4!)))^2 +... )/(((1/(1!)))^2 +((1/(2!)))^2 +((1/(3!)))^2 +......)) |