
Question and Answers Forum
Permutation and CombinationQuestion and Answers: Page 2

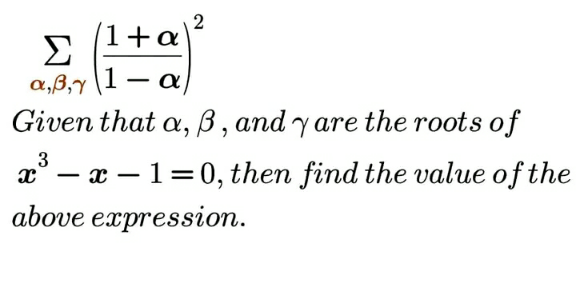





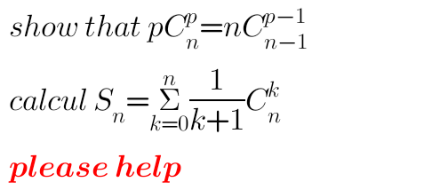
|
Question and Answers Forum |
Permutation and CombinationQuestion and Answers: Page 2 |

|
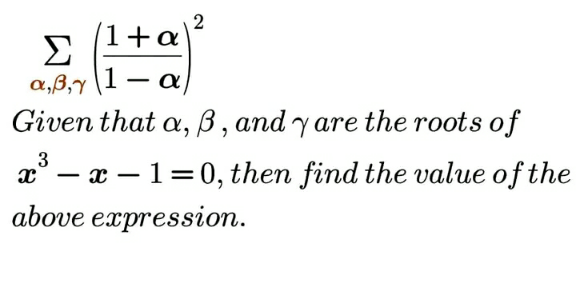
|

|

|
| There are many ways to arrange 3 red balls and 9 black balls in a circle so that there are a minimum of 2 black balls between 2 adjacent red balls. (a) 180×8! (b) 240×7! (c) 364×6! (d) 282×4! (e) 144×5! |

|
| Find the number of integers greater than 6200 that can be formed from the digits 1,3,6,8 and 9, where each digit is used at most once. |

|
| What is the probability that in a class of 18 people, there exists exactly a group of exactly 3 people born on the same day of the week? |
| x |

|
| You want to arrange 17 books on the shelf of a bookstore. The shelf is dedicated to the three Toni Morrison novels published between 1977 and 1987: Song of Solomon, Tar Baby, and Beloved. You have many copies of each, but on the shelf you want an even number of Song of Solomon, at least three copies of Tar Baby, and at most four copies of Beloved. How many different arrangements are possible? |
| sum of roots log _3 x + log _3 (2,5) + log _x 9 = 3+ log _x 5 |
| How many numbers with a maximum of 5 digits, greater than 4000, can be formed with the digits 2, 3, 4, 5, 6; if repetition is allowed for 2 and 3 only? |
| How many numbers with a maximum of 5 digits, greater than 4000, can be formed with the digits 2, 3, 4, 5, 6; if repetition is allowed for 2 and 3 only? |
|
| Five letters are selected from |
| sir...number of 3 digit numbers which are divisible by a)3 b)4 c)6 d)7 e)8 f)9 g)11 when repetetion is 1)Allowwd 2)Not allowed.. kindly help me sir |
| Prove that _(n+1) C_r = _n C_r + _n C_(r−1) |
| how many natural numbers with 4 different digits are divisible by 3? |
| Given the number of consisting of 4 digits abcd such that a≤b≤c≤d is ... (A) 495 (B) 385 (C) 275 (D) 165 (E) 55 |
| f^((1/2)) (x)= (d/dx)(∫_0 ^x ((f(x−t))/( (√(πt))))dt) Prove that (f^((1/2)) )^((1/2)) = f ′ At least for f = 1 then f = x |
| say you have 3 (different) books about mathematics, 4 (different) books about physics and 5 (different) books about chemistry. in how many ways can you arrange them in a shelf such that no two books from the same subject are adjacent? |
| the family A has 5 members and the family B has 4 members. there are 6 personsfrom other families. in how many ways can you arrange these 15 persons around a round table such that no member from family A and no member from family B are next to each other? |
| sequence of string said to be orderly if element index i different to i+1 for example aba has orderly value 2 abab has orderly value 3 abaabb has orderly value 3 if there are 7 a and 13 b example aaaaaaabbbbbbbbbbbbb has orderly value 1 what is the mean of its orderly value for all possible sequences? |