
Question and Answers Forum
Question Number 69231 by ~ À ® @ 237 ~ last updated on 21/Sep/19
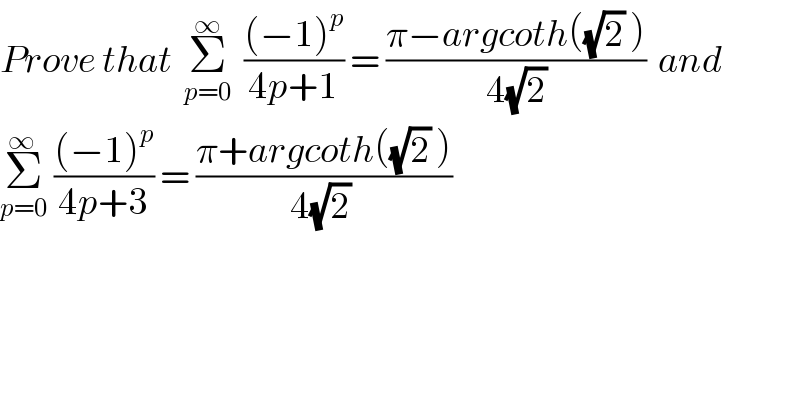
Commented by mathmax by abdo last updated on 21/Sep/19
![let s(x)=Σ_(n=0) ^∞ (((−1)^n )/(4n+1))x^(4n+1) with ∣x∣<1 ⇒s^′ (x)=Σ_(n=0) ^∞ (−1)^n x^(4n) =Σ_(n=0) ^∞ (−x^4 )^n =(1/(1+x^4 )) ⇒s(x) =∫_0 ^x (dt/(1+t^4 )) +c c=s(0)=0 ⇒s(x) =∫_0 ^x (dt/(1+t^4 )) and Σ_(n=0) ^∞ (((−1)^n )/(4n+1)) =s(1)=∫_0 ^1 (dt/(t^4 +1)) let decompose F(t)=(1/(t^4 +1)) ⇒F(t)=(1/((t^2 +1)^2 −2t^2 )) =(1/((t^2 +1+(√2)t)(t^2 +1−(√2)t))) =((at +b)/(t^2 +(√2)t +1)) +((ct+d)/(t^2 −(√2)t +1)) F(−t)=F(t) ⇒((−at+b)/(t^2 −(√2)t +1)) +((−ct+d)/(t^2 +(√2)t +1)) =F(t) ⇒ c=−a and d=b ⇒ F(t) =((at+b)/(t^2 +(√2)t +1)) +((−at+b)/(t^2 −(√2)t +1)) F(o) =1 =2b ⇒b=(1/2) F(1) =((a+b)/(2+(√2))) +((−a+b)/(2−(√2))) =(1/2) ⇒(((2−(√2))(a+b)+(2+(√2))(−a+b))/2)=(1/2) ⇒(2−(√2))a +(2−(√2))b −(2+(√2))a+(2+(√2))b =1 ⇒ −2(√2)a +2 =1 ⇒a =(1/(2(√2))) ⇒ F(t) =(((1/(2(√2)))t +(1/2))/(t^2 +(√2)t +1)) +((−(1/(2(√2)))t+(1/2))/(t^2 −(√2)t +1)) =(1/(2(√2))){ ((t+(√2))/(t^2 +(√2)t+1)) −((t−(√2))/(t^2 −(√2)t +1))} ⇒ ∫_0 ^1 F(t)dt =(1/(4(√2))) ∫_0 ^1 ((2t+(√2)+(√2))/(t^2 +(√2)t +1))dt −(1/(4(√2))) ∫_0 ^1 ((2t−(√2)−(√2))/(t^2 −(√2)t +1))dt =(1/(4(√2)))[ln(t^2 +(√2)t +1)]_0 ^(1 ) +(1/4) ∫_0 ^1 (dt/(t^2 +(√2)t +1)) −(1/(4(√2))) [ln(t^2 −(√2)t +1)]_0 ^1 +(1/4) ∫_0 ^1 (dt/(t^2 −(√2)t +1)) =(1/(4(√2))){ln(2+(√2))−ln(2−(√2)) +(1/4) { ∫_0 ^1 (...)dt +∫_0 ^1 (....)dt} ∫_0 ^1 (dt/(t^2 +(√2)t +1)) =∫_0 ^1 (dt/(t^2 +2((√2)/2)t +(1/2)+(1/2))) =∫_0 ^1 (dt/((t+(1/(√2)))^(2 ) +(1/2))) =_(t+(1/(√2))=(u/(√2))) 2∫_1 ^(1+(√2)) (1/(u^2 +1)) (du/(√2)) =(√2)[arctan(u)]_1 ^(1+(√2)) =(√2){arctan(1+(√2))−(π/4)} =(√2){(π/2) −arctan((√2)−1)−(π/4)} =(√2){(π/4)−(π/8)} =(√2)×(π/8) ∫_0 ^1 (dt/(t^2 −(√2)t +1)) =∫_0 ^1 (dt/((t−(1/(√2)))^2 +(1/2))) =_(t−(1/(√2))=(u/(√2))) 2 ∫_(−1) ^((√2)−1) (1/(u^2 +1))(du/(√2)) =(√2)[arctan(u)]_(−1) ^((√2)−1) =(√2){(π/8) +(π/4)} =(√2)×((3π)/8) ⇒ ∫_0 ^1 F(t)dt =(1/(4(√2)))ln(((2+(√2))/(2−(√2)))) +(1/4){((π(√2))/8) +((3π(√2))/8)} =(1/(4(√2)))ln(((4+4(√2)+2)/2))+(1/4)((π(√2))/2) =(1/(4(√2)))ln(3+2(√2))+((π(√2))/8) ⇒ Σ_(n=0) ^∞ (((−1)^n )/(4n+1)) =(1/(4(√2)))ln(3+2(√2)) +((π(√2))/8)](Q69250.png)
| ||
Question and Answers Forum | ||
Question Number 69231 by ~ À ® @ 237 ~ last updated on 21/Sep/19 | ||
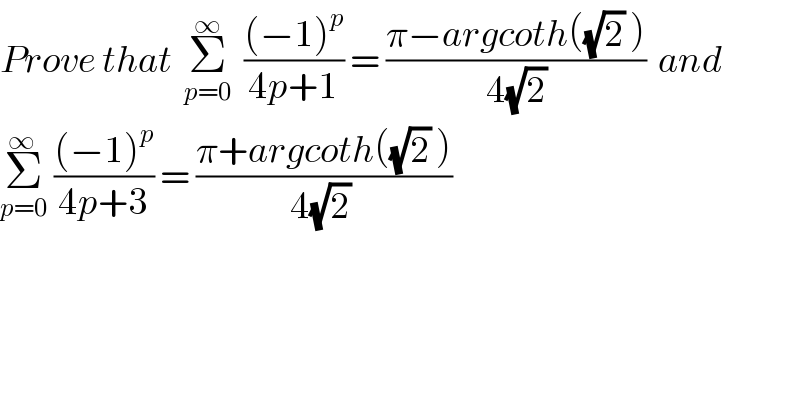 | ||
Commented by mathmax by abdo last updated on 21/Sep/19 | ||
![let s(x)=Σ_(n=0) ^∞ (((−1)^n )/(4n+1))x^(4n+1) with ∣x∣<1 ⇒s^′ (x)=Σ_(n=0) ^∞ (−1)^n x^(4n) =Σ_(n=0) ^∞ (−x^4 )^n =(1/(1+x^4 )) ⇒s(x) =∫_0 ^x (dt/(1+t^4 )) +c c=s(0)=0 ⇒s(x) =∫_0 ^x (dt/(1+t^4 )) and Σ_(n=0) ^∞ (((−1)^n )/(4n+1)) =s(1)=∫_0 ^1 (dt/(t^4 +1)) let decompose F(t)=(1/(t^4 +1)) ⇒F(t)=(1/((t^2 +1)^2 −2t^2 )) =(1/((t^2 +1+(√2)t)(t^2 +1−(√2)t))) =((at +b)/(t^2 +(√2)t +1)) +((ct+d)/(t^2 −(√2)t +1)) F(−t)=F(t) ⇒((−at+b)/(t^2 −(√2)t +1)) +((−ct+d)/(t^2 +(√2)t +1)) =F(t) ⇒ c=−a and d=b ⇒ F(t) =((at+b)/(t^2 +(√2)t +1)) +((−at+b)/(t^2 −(√2)t +1)) F(o) =1 =2b ⇒b=(1/2) F(1) =((a+b)/(2+(√2))) +((−a+b)/(2−(√2))) =(1/2) ⇒(((2−(√2))(a+b)+(2+(√2))(−a+b))/2)=(1/2) ⇒(2−(√2))a +(2−(√2))b −(2+(√2))a+(2+(√2))b =1 ⇒ −2(√2)a +2 =1 ⇒a =(1/(2(√2))) ⇒ F(t) =(((1/(2(√2)))t +(1/2))/(t^2 +(√2)t +1)) +((−(1/(2(√2)))t+(1/2))/(t^2 −(√2)t +1)) =(1/(2(√2))){ ((t+(√2))/(t^2 +(√2)t+1)) −((t−(√2))/(t^2 −(√2)t +1))} ⇒ ∫_0 ^1 F(t)dt =(1/(4(√2))) ∫_0 ^1 ((2t+(√2)+(√2))/(t^2 +(√2)t +1))dt −(1/(4(√2))) ∫_0 ^1 ((2t−(√2)−(√2))/(t^2 −(√2)t +1))dt =(1/(4(√2)))[ln(t^2 +(√2)t +1)]_0 ^(1 ) +(1/4) ∫_0 ^1 (dt/(t^2 +(√2)t +1)) −(1/(4(√2))) [ln(t^2 −(√2)t +1)]_0 ^1 +(1/4) ∫_0 ^1 (dt/(t^2 −(√2)t +1)) =(1/(4(√2))){ln(2+(√2))−ln(2−(√2)) +(1/4) { ∫_0 ^1 (...)dt +∫_0 ^1 (....)dt} ∫_0 ^1 (dt/(t^2 +(√2)t +1)) =∫_0 ^1 (dt/(t^2 +2((√2)/2)t +(1/2)+(1/2))) =∫_0 ^1 (dt/((t+(1/(√2)))^(2 ) +(1/2))) =_(t+(1/(√2))=(u/(√2))) 2∫_1 ^(1+(√2)) (1/(u^2 +1)) (du/(√2)) =(√2)[arctan(u)]_1 ^(1+(√2)) =(√2){arctan(1+(√2))−(π/4)} =(√2){(π/2) −arctan((√2)−1)−(π/4)} =(√2){(π/4)−(π/8)} =(√2)×(π/8) ∫_0 ^1 (dt/(t^2 −(√2)t +1)) =∫_0 ^1 (dt/((t−(1/(√2)))^2 +(1/2))) =_(t−(1/(√2))=(u/(√2))) 2 ∫_(−1) ^((√2)−1) (1/(u^2 +1))(du/(√2)) =(√2)[arctan(u)]_(−1) ^((√2)−1) =(√2){(π/8) +(π/4)} =(√2)×((3π)/8) ⇒ ∫_0 ^1 F(t)dt =(1/(4(√2)))ln(((2+(√2))/(2−(√2)))) +(1/4){((π(√2))/8) +((3π(√2))/8)} =(1/(4(√2)))ln(((4+4(√2)+2)/2))+(1/4)((π(√2))/2) =(1/(4(√2)))ln(3+2(√2))+((π(√2))/8) ⇒ Σ_(n=0) ^∞ (((−1)^n )/(4n+1)) =(1/(4(√2)))ln(3+2(√2)) +((π(√2))/8)](Q69250.png) | ||