Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 174267 by mnjuly1970 last updated on 28/Jul/22

$$$$$$\mathrm{Q}::\mathrm{Find}\mathrm{the}\mathrm{value}\mathrm{of}:$$$$$$$$\mathbb{M}{\mathrm{ax}}_{x\in [0,\frac{\pi }{2}]}\{\sin \left(x\right).{\cos }^3\left(x\right)\mid \}=?$$$$$$$$$$

Commented by kaivan.ahmadi last updated on 28/Jul/22

$$f^{\prime }\left(x\right)={cos}^{4}x-3{sin}^2{xcos}^{2}x=0$$$$\Rightarrow {cos}^{2}x({cos}^{2}x-3{sin}^{2}x)=0\Rightarrow$$$${cos}^{2}x=0\Rightarrow x=\frac{\pi }{2}$$$${cos}^{2}x-3{sin}^{2}x=0\Rightarrow {tan}^{2}x=\frac{1}{3}$$$$\Rightarrow tanx=\frac{\sqrt{3}}{3}\Rightarrow x=\frac{\pi }{6}$$$$f\left(0\right)=0$$$$f\left(\frac{\pi }{2}\right)=0$$$$f\left(\frac{\pi }{3}\right)=\frac{1}{2}\times {\left(\frac{\sqrt{3}}{2}\right)}^3=\frac{3\sqrt{3}}{16}$$$$Maxf=\frac{3\sqrt{3}}{16}$$

Commented by mnjuly1970 last updated on 28/Jul/22

$$thanksalotsir$$$$also...\frac{3\sqrt{3}}{16}typo$$

Commented by kaivan.ahmadi last updated on 28/Jul/22

$$anotherway$$$$a={sin}^{2}x,b={cos}^{2}x,a+b=1$$$$Max\left(f\right)=Max{\left({sin}^{2}x\right)}^{\frac{1}{2}}.{\left({cos}^{2}x\right)}^{\frac{3}{2}}=$$$$a^{\frac{1}{2}}{b}^{\frac{3}{2}}:\frac{a}{\frac{1}{2}}=\frac{b}{\frac{3}{2}}$$$$b=3a,a+b=1\Rightarrow a=\frac{1}{4},b=\frac{3}{4}$$$$Max\left(f\right)={\left(\frac{1}{4}\right)}^{\frac{1}{2}}{\left(\frac{3}{4}\right)}^{\frac{3}{2}}=$$$$\frac{1}{2}\sqrt{{\left(\frac{3}{4}\right)}^3}=\frac{1}{2}\times \frac{3\sqrt{3}}{8}=\frac{3\sqrt{3}}{16}$$

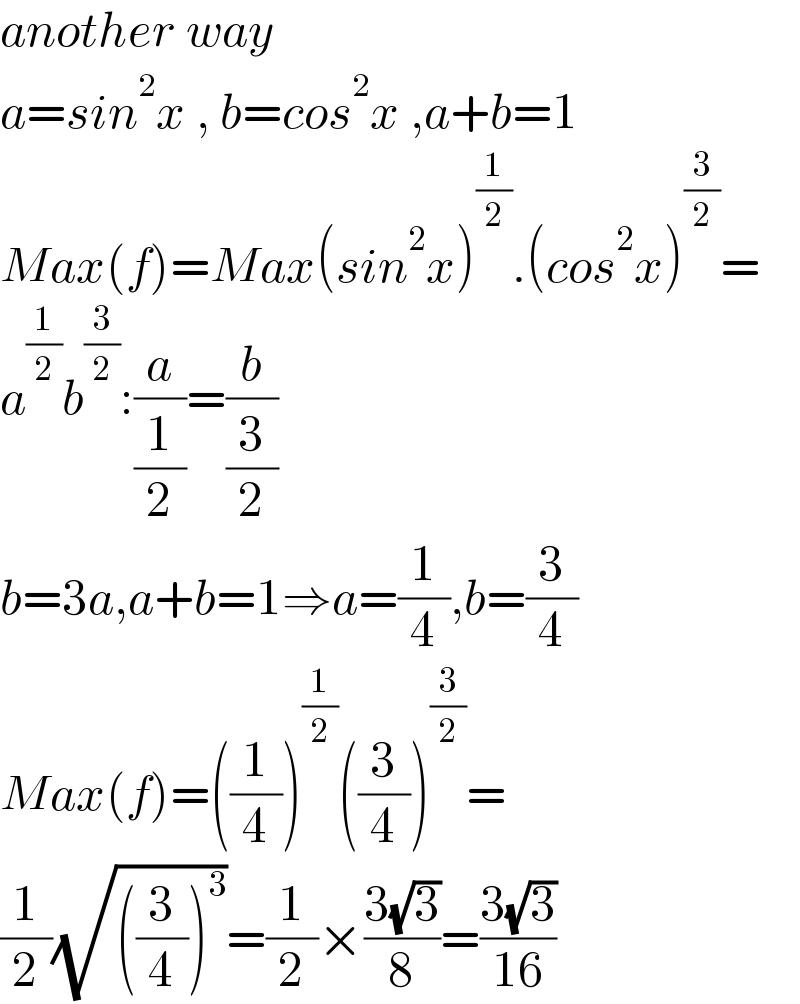
Commented by infinityaction last updated on 28/Jul/22

$$how$$$$\frac{a}{\frac{1}{2}}=\frac{b}{\frac{3}{2}}$$

Commented by mnjuly1970 last updated on 28/Jul/22

$$becausea+b=cte$$$$$$

Commented by kaivan.ahmadi last updated on 28/Jul/22

$$proposition:$$$$ifa,b>0anda+b=k>0\left(cte\right)$$$$\Rightarrow Max(a^m.b^n):\frac{a}{m}=\frac{b}{n}$$

Commented by Tawa11 last updated on 28/Jul/22

$$\mathrm{Great}\mathrm{sirs}$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com