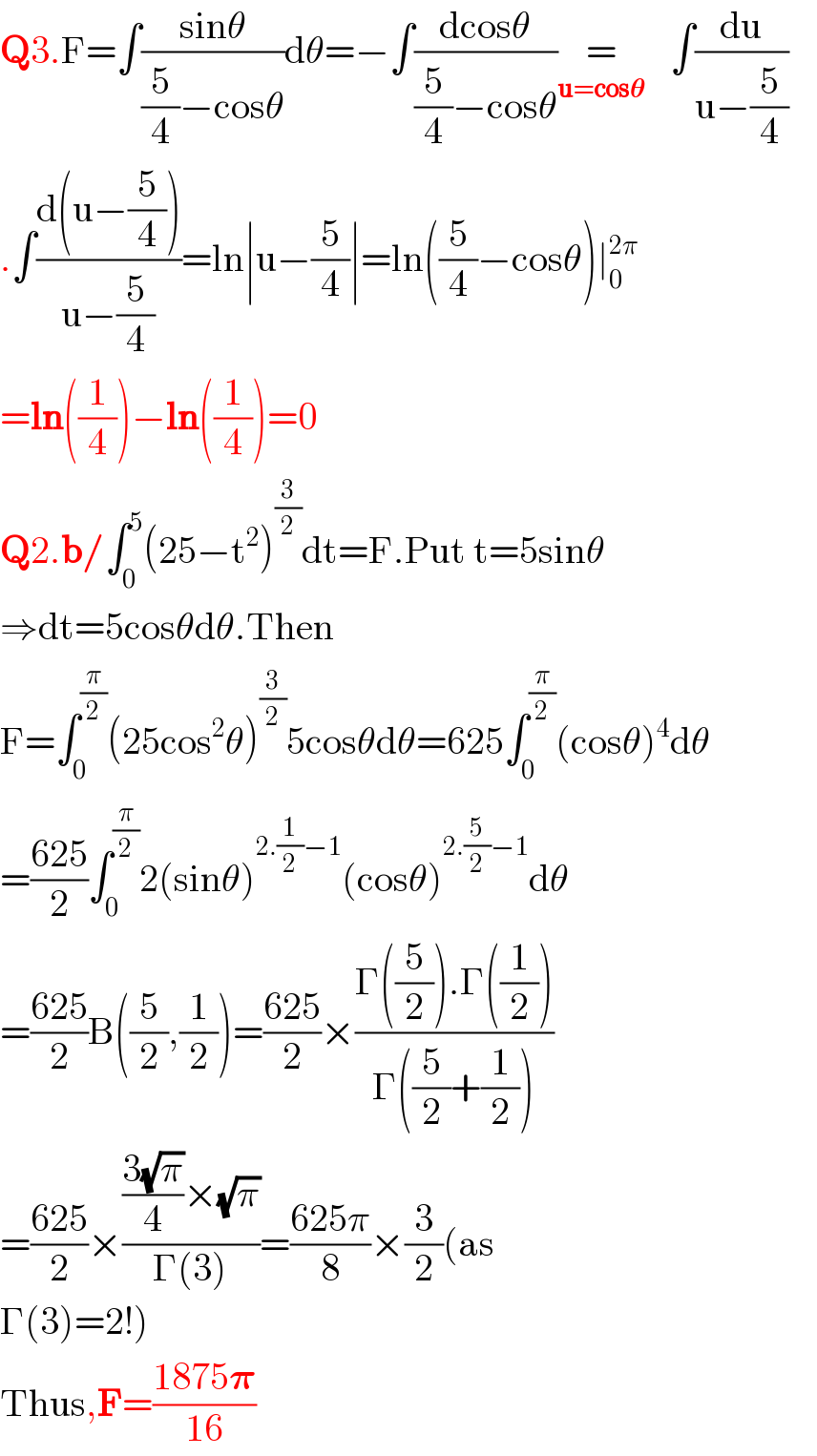Math Question and Answers


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 103345 by harckinwunmy last updated on 14/Jul/20

Commented by harckinwunmy last updated on 14/Jul/20

$please help$
Commented by Dwaipayan Shikari last updated on 14/Jul/20
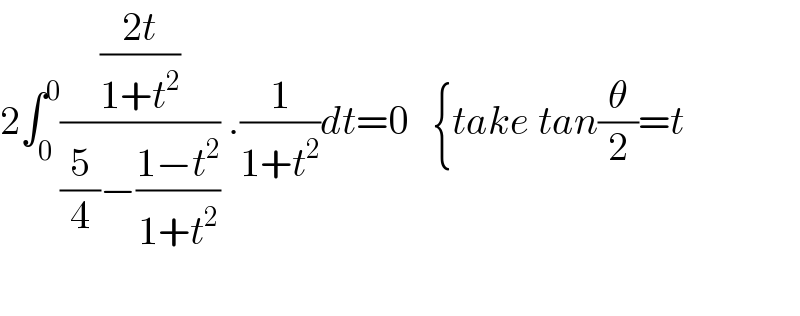
$2∫_0 ^0 (((2t)/(1+t^2 ))/((5/4)−((1−t^2 )/(1+t^2 )))) .(1/(1+t^2 ))dt=0 {take tan(θ/2)=t$
$2 \int_{0}^{0} \frac{\frac{2 t}{1 + t^{2}}}{\frac{5}{4} - \frac{1 - t^{2}}{1 + t^{2}}} . \frac{1}{1 + t^{2}} d t = 0 {t a k e t a n \frac{θ}{2} = t$
Commented by mathmax by abdo last updated on 14/Jul/20

$not correct sir you must have a bijection . . .!$
Commented by Dwaipayan Shikari last updated on 14/Jul/20

$\int_{0}^{0} f (x) d x = 0$
Commented by mathmax by abdo last updated on 15/Jul/20

$\tan is bijective on] - \frac{π}{2}, \frac{π}{2} [not [0, 2 π] be carefull$
	Answered by abdomathmax last updated on 14/Jul/20

	$Q 2 \frac{\partial u}{\partial x} (x, y) = \frac{1 (x^{2} + y^{2}) - 2 x (x - y)}{{(x^{2} + y^{2})}^{2}}$ $= \frac{- x^{2} + y^{2} + 2 xy}{{(x^{2} + y^{2})}^{2}}$ $\frac{\partial^{2} u}{\partial x^{2}} (x, y) = \frac{(- 2 x + 2 y) {(x^{2} + y^{2})}^{2} - 2 (2 x) (x^{2} + y^{2})}{{(x^{2} + y^{2})}^{4}}$ $= \frac{(- 2 x + 2 y) (x^{2} + y^{2}) - 4 x}{{(x^{2} + y^{2})}^{3}}$ $= \frac{- {2 x}^{3} - {2 xy}^{2} + {2 yx}^{2} - 4 x}{{(x^{2} + y^{2})}^{3}} after we calculate$ $\frac{\partial^{2} u}{\partial y^{2}} (x, y) and prove that \frac{\partial^{2} u}{\partial x^{2}} + \frac{\partial^{2} u}{\partial y^{2}} = 0$
	Answered by abdomathmax last updated on 14/Jul/20

	$let I = \int_{0}^{5} {(25 - t^{2})}^{\frac{3}{2}} dt we do the changeme 7 t$ $t = 5 x \Rightarrow I = \int_{0}^{1} {(25 - {25 x}^{2})}^{\frac{3}{2}} 5 dx$ $= 5 . {(5^{2})}^{\frac{3}{2}} \int_{0}^{1} {(1 - x^{2})}^{\frac{3}{2}} dx$ $=_{x = u^{\frac{1}{2}}} 5^{4} \int_{0}^{1} {(1 - u)}^{\frac{3}{2}} \frac{1}{2} u^{- \frac{1}{2}} du$ $= \frac{5^{4}}{2} \int_{0}^{1} u^{- \frac{1}{2}} {(1 - u)}^{\frac{3}{2}} du we havd$ $B (p, q) = \int_{0}^{1} x^{p - 1} {(1 - x)}^{q - 1} dx$ $p - 1 = - \frac{1}{2} \Rightarrow p = \frac{1}{2} and q - 1 = \frac{3}{2} \Rightarrow q = 1 + \frac{3}{2} = \frac{5}{2}$ $\Rightarrow I = \frac{5^{4}}{2} \times B (\frac{1}{2}, \frac{5}{2}) .$
	Answered by abdomathmax last updated on 14/Jul/20
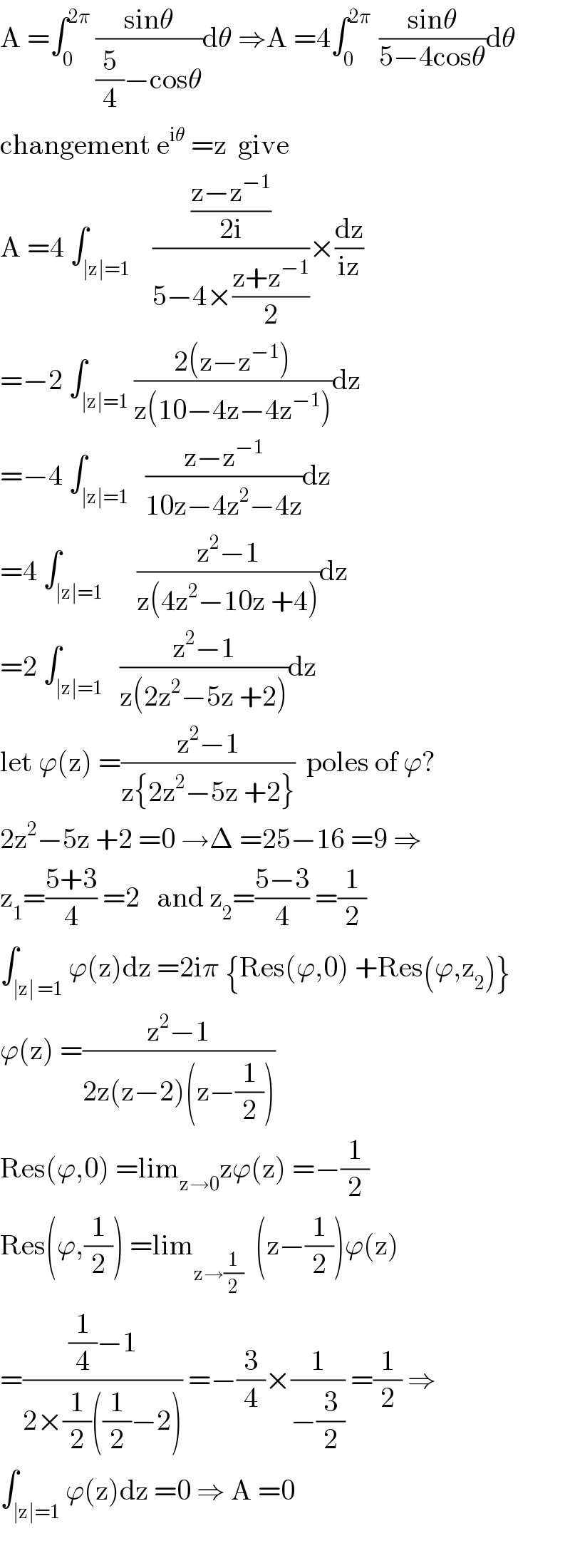
	$A =∫_0 ^(2π) ((sinθ)/((5/4)−cosθ))dθ ⇒A =4∫_0 ^(2π ) ((sinθ)/(5−4cosθ))dθ changement e^(iθ) =z give A =4 ∫_(∣z∣=1) (((z−z^(−1) )/(2i))/(5−4×((z+z^(−1) )/2)))×(dz/(iz)) =−2 ∫_(∣z∣=1) ((2(z−z^(−1) ))/(z(10−4z−4z^(−1) )))dz =−4 ∫_(∣z∣=1) ((z−z^(−1) )/(10z−4z^2 −4z))dz =4 ∫_(∣z∣=1) ((z^2 −1)/(z(4z^2 −10z +4)))dz =2 ∫_(∣z∣=1) ((z^2 −1)/(z(2z^2 −5z +2)))dz let ϕ(z) =((z^2 −1)/(z{2z^2 −5z +2})) poles of ϕ? 2z^2 −5z +2 =0 →Δ =25−16 =9 ⇒ z_1 =((5+3)/4) =2 and z_2 =((5−3)/4) =(1/2) ∫_(∣z∣ =1) ϕ(z)dz =2iπ {Res(ϕ,0) +Res(ϕ,z_2 )} ϕ(z) =((z^2 −1)/(2z(z−2)(z−(1/2)))) Res(ϕ,0) =lim_(z→0) zϕ(z) =−(1/2) Res(ϕ,(1/2)) =lim_(z→(1/2)) (z−(1/2))ϕ(z) =(((1/4)−1)/(2×(1/2)((1/2)−2))) =−(3/4)×(1/(−(3/2))) =(1/2) ⇒ ∫_(∣z∣=1) ϕ(z)dz =0 ⇒ A =0$
	$A = \int_{0}^{2 π} \frac{\sin θ}{\frac{5}{4} - \cos θ} d θ \Rightarrow A = 4 \int_{0}^{2 π} \frac{\sin θ}{5 - 4 \cos θ} d θ$ $changement e^{i θ} = z give$ $A = 4 \int_{∣ z ∣= 1} \frac{\frac{z - z^{- 1}}{2 i}}{5 - 4 \times \frac{z + z^{- 1}}{2}} \times \frac{dz}{iz}$ $= - 2 \int_{∣ z ∣= 1} \frac{2 (z - z^{- 1})}{z (10 - 4 z - {4 z}^{- 1})} dz$ $= - 4 \int_{∣ z ∣= 1} \frac{z - z^{- 1}}{10 z - {4 z}^{2} - 4 z} dz$ $= 4 \int_{∣ z ∣= 1} \frac{z^{2} - 1}{z ({4 z}^{2} - 10 z + 4)} dz$ $= 2 \int_{∣ z ∣= 1} \frac{z^{2} - 1}{z ({2 z}^{2} - 5 z + 2)} dz$ $let φ (z) = \frac{z^{2} - 1}{z {{2 z}^{2} - 5 z + 2}} poles of φ ?$ ${2 z}^{2} - 5 z + 2 = 0 \to Δ = 25 - 16 = 9 \Rightarrow$ $z_{1} = \frac{5 + 3}{4} = 2 and z_{2} = \frac{5 - 3}{4} = \frac{1}{2}$ $\int_{∣ z ∣ = 1} φ (z) dz = 2 i π {Res (φ, 0) + Res (φ, z_{2})}$ $φ (z) = \frac{z^{2} - 1}{2 z (z - 2) (z - \frac{1}{2})}$ $Res (φ, 0) = \lim_{z \to 0} z φ (z) = - \frac{1}{2}$ $Res (φ, \frac{1}{2}) = \lim_{z \to \frac{1}{2}} (z - \frac{1}{2}) φ (z)$ $= \frac{\frac{1}{4} - 1}{2 \times \frac{1}{2} (\frac{1}{2} - 2)} = - \frac{3}{4} \times \frac{1}{- \frac{3}{2}} = \frac{1}{2} \Rightarrow$ $\int_{∣ z ∣= 1} φ (z) dz = 0 \Rightarrow A = 0$
	Answered by 1549442205 last updated on 15/Jul/20

	$Q 3 . F = \int \frac{\sin θ}{\frac{5}{4} - \cos θ} d θ = - \int \frac{dcos θ}{\frac{5}{4} - \cos θ} \underset{u = \cos θ}{=} \int \frac{du}{u - \frac{5}{4}}$ $. \int \frac{d (u - \frac{5}{4})}{u - \frac{5}{4}} = \ln ∣ u - \frac{5}{4} ∣= \ln (\frac{5}{4} - \cos θ) ∣_{0}^{2 π}$ $= \ln (\frac{1}{4}) - \ln (\frac{1}{4}) = 0$ $Q 2 . b / \int_{0}^{5} {(25 - t^{2})}^{\frac{3}{2}} dt = F . Put t = 5 \sin θ$ $\Rightarrow dt = 5 \cos θ d θ . Then$ $F = \int_{0}^{\frac{π}{2}} {({25 \cos}^{2} θ)}^{\frac{3}{2}} 5 \cos θ d θ = 625 \int_{0}^{\frac{π}{2}} {(\cos θ)}^{4} d θ$ $= \frac{625}{2} \int_{0}^{\frac{π}{2}} 2 {(\sin θ)}^{2 . \frac{1}{2} - 1} {(\cos θ)}^{2 . \frac{5}{2} - 1} d θ$ $= \frac{625}{2} B (\frac{5}{2}, \frac{1}{2}) = \frac{625}{2} \times \frac{Γ (\frac{5}{2}) . Γ (\frac{1}{2})}{Γ (\frac{5}{2} + \frac{1}{2})}$ $= \frac{625}{2} \times \frac{\frac{3 \sqrt{π}}{4} \times \sqrt{π}}{Γ (3)} = \frac{625 π}{8} \times \frac{3}{2} (as$ $Γ (3) = 2!)$ $Thus, F = \frac{1875 π}{16}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum