Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 108864 by I want to learn more last updated on 19/Aug/20

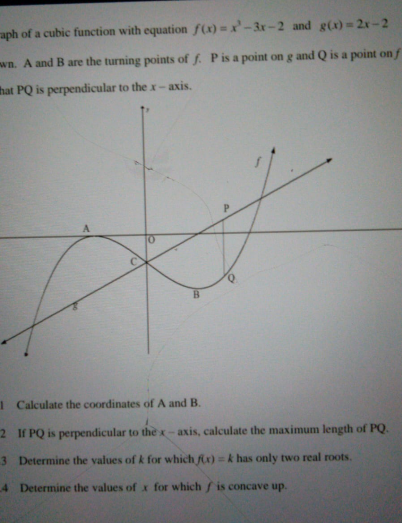
Commented by I want to learn more last updated on 19/Aug/20

$$\mathrm{What}\mathrm{is}\mathrm{the}\mathrm{maximum}\mathrm{length}\mathrm{of}\mathrm{PQ}$$

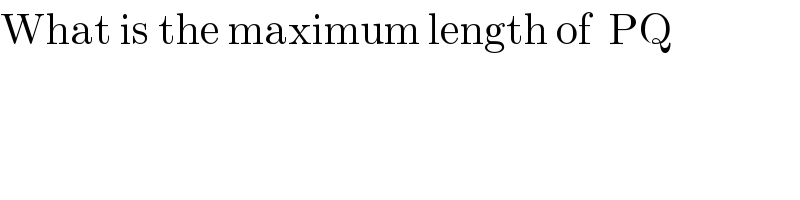
Answered by mr W last updated on 19/Aug/20

$$f\left(x\right)=x^3-3x-2$$$$g\left(x\right)=2x-2$$$$f^{\prime }\left(x\right)=3x^2-3$$$$suchthatPQismaximum,tangent$$$$atQmustbeparalleltog\left(x\right).$$$$f^{\prime }\left(x\right)=3x^2-3=g^{\prime }\left(x\right)=2$$$$\Rightarrow x=\pm \sqrt{\frac{5}{3}}$$$$atx=-\sqrt{\frac{5}{3}}:$$$$y_P=-2\sqrt{\frac{5}{3}}-2$$$$y_Q=-\frac{5}{3}\sqrt{\frac{5}{3}}+3\sqrt{\frac{5}{3}}-2$$$$PQ=-\frac{5}{3}\sqrt{\frac{5}{3}}+3\sqrt{\frac{5}{3}}-2-(-2\sqrt{\frac{5}{3}}-2)$$$$=\frac{10\sqrt{15}}{9}$$$$atx=\sqrt{\frac{5}{3}}:$$$$y_P=2\sqrt{\frac{5}{3}}-2$$$$y_Q=\frac{5}{3}\sqrt{\frac{5}{3}}-3\sqrt{\frac{5}{3}}-2$$$$PQ=2\sqrt{\frac{5}{3}}-2-(\frac{5}{3}\sqrt{\frac{5}{3}}-3\sqrt{\frac{5}{3}}-2)$$$$=\frac{10\sqrt{15}}{9}$$$$$$$$\Rightarrow {PQ}_{max,local}=\frac{10\sqrt{15}}{9}$$$$$$$${PQ}_{max}=\mathrm{\infty }whenx\to \pm \mathrm{\infty }$$

Commented by I want to learn more last updated on 25/Aug/20

$$\mathrm{Thanks}\mathrm{sir},\mathrm{I}\mathrm{appreciate}$$

Answered by Aziztisffola last updated on 19/Aug/20

$$\mathrm{P}(\mathrm{x};2\mathrm{x}-2)\mathrm{and}\mathrm{Q}(\mathrm{x};{\mathrm{x}}^3-3\mathrm{x}-2)$$$$\mathrm{PQ}\left(\mathrm{x}\right)=\sqrt{0^2+{({\mathrm{x}}^3-3\mathrm{x}-2-2\mathrm{x}+2)}^2}$$$$={\mathrm{x}}^3-5\mathrm{x}$$$$\mathrm{PQ}{\left(\mathrm{x}\right)}^{\prime }={3\mathrm{x}}^2-5$$$$\mathrm{PQ}{\left(\mathrm{x}\right)}^{\prime }=0\Rightarrow {3\mathrm{x}}^2-5=0\Rightarrow \mathrm{x}=\underset{-}{+}\sqrt{\frac{5}{3}}$$$$\min \mathrm{in}\mathrm{x}=\sqrt{\frac{5}{3}}$$$$\mathrm{Max}\mathrm{local}\mathrm{in}\mathrm{x}=-\sqrt{\frac{5}{3}};\mathrm{look}\mathrm{at}\mathrm{variation}\mathrm{of}\mathrm{PQ}$$$$\mathrm{then}\mathrm{PQ}(-\sqrt{\frac{5}{3}})={(-\sqrt{\frac{5}{3}})}^3+5\sqrt{\frac{5}{3}}$$$$=\frac{10\sqrt{15}}{9}$$

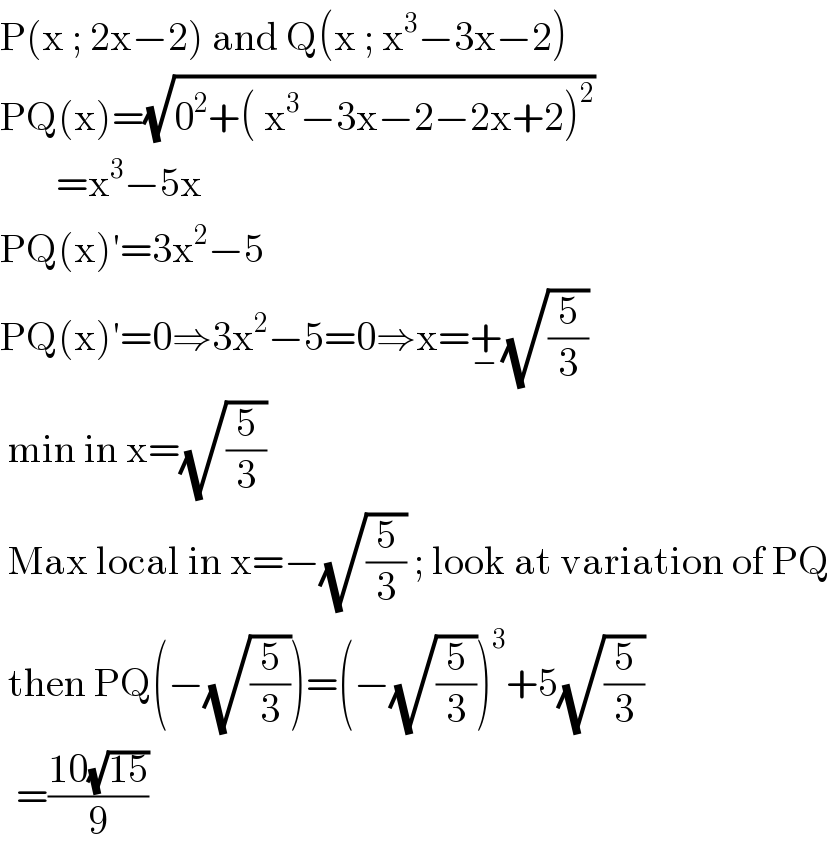
Commented by I want to learn more last updated on 25/Aug/20

$$\mathrm{Thanks}\mathrm{sir},\mathrm{i}\mathrm{appreciate}$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com