
Question and Answers Forum
Question Number 162117 by CM last updated on 27/Dec/21

Commented by cortano last updated on 27/Dec/21
![∫ x^2 (√(4 −(1−x)^2 )) dx =? [ 1−x = 2sin t ⇒dx=−2cos t dt ] ∫ (1−2sin t)^2 (−2cos^2 t)dt = −∫(3−4sin t−2cos 2t)(1+cos 2t)dt =−∫(2+cos 2t−4sin t−4sin t cos 2t−cos 4t)dt =−2t−((sin 2t)/2)−4cos t+((sin 4t)/4)+∫4sin t cos 2t dt = −2arcsin (((1−x)/2))−2(√(3+2x−x^2 ))−(((1−x)(√(3+2x−x^2 )))/4) +4∫(2cos^2 t−1)d(cos t) = −2arcsin (((1−x)/2))−(((9−x)/4))(√(3+2x−x^2 )) +(8/3)cos^3 t−4cos t + c](Q162122.png)
Answered by FongXD last updated on 27/Dec/21
/2))^2 ))=(1/( 8))(3+2x−x^2 )(√(3+2x−x^2 )) • sin2θ=2sinθ(√(1−sin^2 θ))=2(((x−1)/2))(√(1−(((x−1)/2))^2 ))=(1/2)(x−1)(√(3+2x−x^2 )) • sin4θ=2sin2θcos2θ=2sin2θ(1−2sin^2 θ) ⇔ sin4θ=(x−1)(√(3+2x−x^2 ))[1−2(((x−1)/2))^2 ] ⇒ sin4θ=(1/2)(x−1)(1+2x−x^2 )(√(3+2x−x^2 )) (∗): I=4arcsin(((x−1)/2))−(1/4)(x−1)(1+2x−x^2 )(√(3+2x−x^2 ))+(1/2)(x−1)(√(3+2x−x^2 ))−(2/3)(3+2x−x^2 )(√(3+2x−x^2 ))+c I=4arcsin(((x−1)/2))+(1/4)(x−1)(x^2 −2x+1)(√(3+2x−x^2 ))−(2/3)(3+2x−x^2 )(√(3+2x−x^2 ))+c I=4arcsin(((x−1)/2))+(1/(12))[3(x^3 −3x^2 +3x−1)−8(3+2x−x^2 )](√(3+2x−x^2 ))+c ⇒ I=4arcsin(((x−1)/2))+(1/(12))(3x^3 −x^2 −7x−27)(√(3+2x−x^2 ))+c](Q162123.png)
Answered by mathmax by abdo last updated on 27/Dec/21
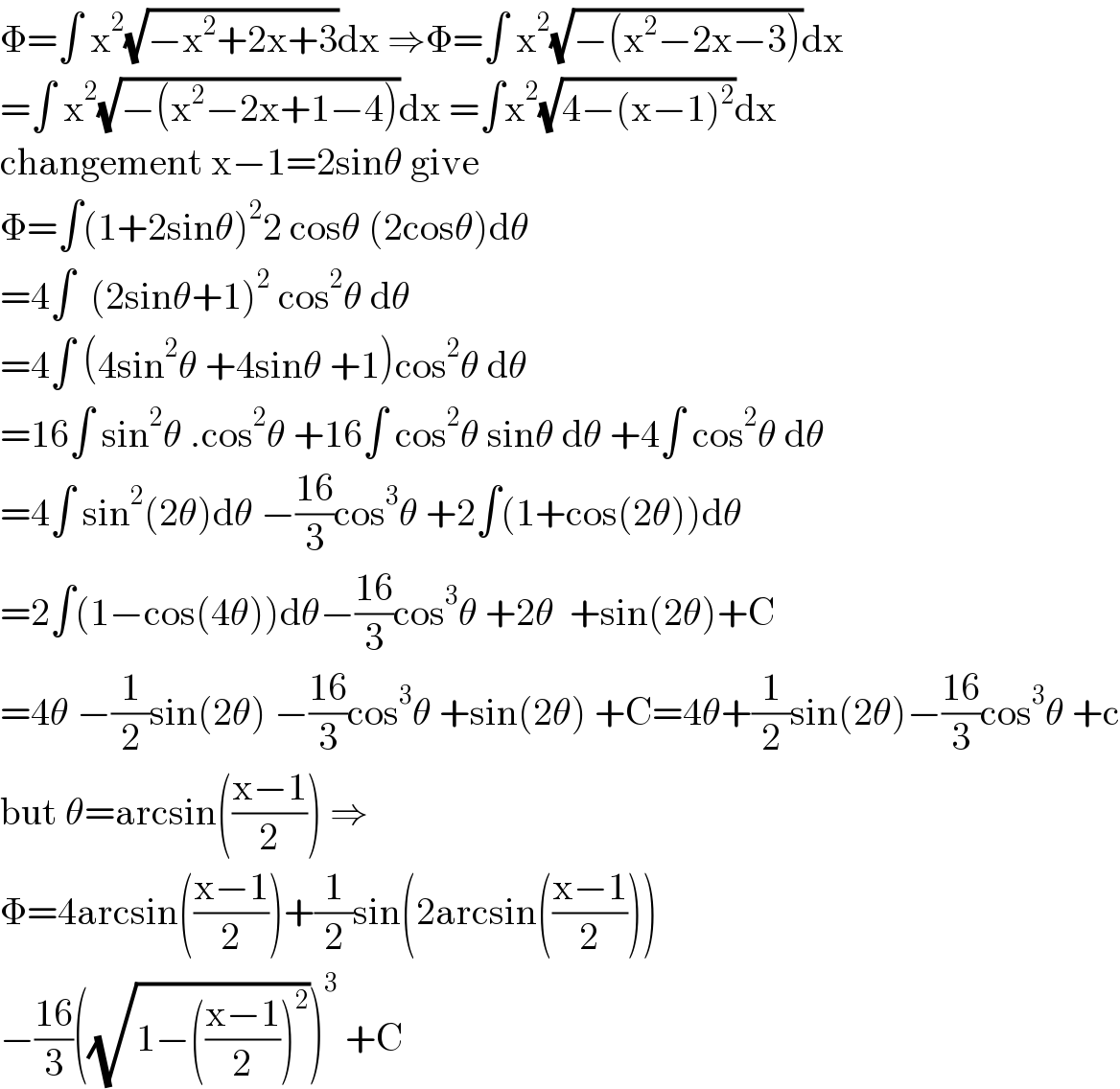
| ||
Question and Answers Forum | ||
Question Number 162117 by CM last updated on 27/Dec/21 | ||
 | ||
Commented by cortano last updated on 27/Dec/21 | ||
![∫ x^2 (√(4 −(1−x)^2 )) dx =? [ 1−x = 2sin t ⇒dx=−2cos t dt ] ∫ (1−2sin t)^2 (−2cos^2 t)dt = −∫(3−4sin t−2cos 2t)(1+cos 2t)dt =−∫(2+cos 2t−4sin t−4sin t cos 2t−cos 4t)dt =−2t−((sin 2t)/2)−4cos t+((sin 4t)/4)+∫4sin t cos 2t dt = −2arcsin (((1−x)/2))−2(√(3+2x−x^2 ))−(((1−x)(√(3+2x−x^2 )))/4) +4∫(2cos^2 t−1)d(cos t) = −2arcsin (((1−x)/2))−(((9−x)/4))(√(3+2x−x^2 )) +(8/3)cos^3 t−4cos t + c](Q162122.png) | ||
Answered by FongXD last updated on 27/Dec/21 | ||
/2))^2 ))=(1/( 8))(3+2x−x^2 )(√(3+2x−x^2 )) • sin2θ=2sinθ(√(1−sin^2 θ))=2(((x−1)/2))(√(1−(((x−1)/2))^2 ))=(1/2)(x−1)(√(3+2x−x^2 )) • sin4θ=2sin2θcos2θ=2sin2θ(1−2sin^2 θ) ⇔ sin4θ=(x−1)(√(3+2x−x^2 ))[1−2(((x−1)/2))^2 ] ⇒ sin4θ=(1/2)(x−1)(1+2x−x^2 )(√(3+2x−x^2 )) (∗): I=4arcsin(((x−1)/2))−(1/4)(x−1)(1+2x−x^2 )(√(3+2x−x^2 ))+(1/2)(x−1)(√(3+2x−x^2 ))−(2/3)(3+2x−x^2 )(√(3+2x−x^2 ))+c I=4arcsin(((x−1)/2))+(1/4)(x−1)(x^2 −2x+1)(√(3+2x−x^2 ))−(2/3)(3+2x−x^2 )(√(3+2x−x^2 ))+c I=4arcsin(((x−1)/2))+(1/(12))[3(x^3 −3x^2 +3x−1)−8(3+2x−x^2 )](√(3+2x−x^2 ))+c ⇒ I=4arcsin(((x−1)/2))+(1/(12))(3x^3 −x^2 −7x−27)(√(3+2x−x^2 ))+c](Q162123.png) | ||
| ||
Answered by mathmax by abdo last updated on 27/Dec/21 | ||
 | ||
| ||