Question and Answers Forum

All Questions      Topic List

Mechanics Questions

Previous in All Question      Next in All Question      

Previous in Mechanics      Next in Mechanics      

Question Number 178037 by mr W last updated on 12/Oct/22

Commented by mr W last updated on 12/Oct/22

$$aropewithmassmandlengthbis$$$$connectedononeendwitharodand$$$$itsotherendisfixedontheceiling$$$$inaheighthabovetheground.the$$$$rodwithmassMandlengthLis$$$$connectedthroughahingewiththe$$$$ground.$$$$findtheangle\theta .$$$$(M>m,L+b>h)$$

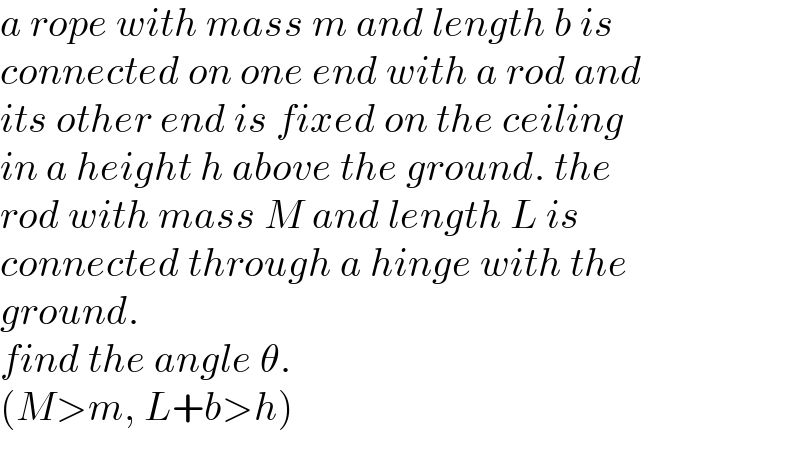
Answered by mr W last updated on 12/Oct/22

Commented by Tawa11 last updated on 12/Oct/22

$$\mathrm{Great}\mathrm{sir}.\mathrm{Weldone}\mathrm{sir}$$

Commented by mr W last updated on 14/Oct/22

$$\rho =\frac{m}{b}$$$$let\mu =\frac{m}{M},\eta =\frac{h}{L},\lambda =\frac{b}{L}$$$$Mg\frac{L\sin \theta }{2}=T_{1}L\sin (\frac{\pi }{2}-\theta +\varphi )$$$$T_1=\frac{Mg\sin \theta }{2\cos (\theta -\varphi )}$$$$T_0=T_1\cos \varphi$$$$a=\frac{T_0}{\rho g}=\frac{Mgb\sin \theta \cos \varphi }{2mg\cos (\theta -\varphi )}=\frac{b}{2\mu (\frac{1}{\tan \theta }+\tan \varphi )}$$$$\sinh \frac{d_1}{a}=\tan \varphi$$$$\Rightarrow \frac{d_1}{a}={\sinh }^{-1}\left(\tan \varphi \right)$$$$\frac{h_1}{a}=\cosh \frac{d_1}{a}=\sqrt{1+{\tan }^2\varphi }$$$$$$$$\frac{s_1}{a}=\tan \varphi$$$$h_2=h_1+h-L\cos \theta$$$$\frac{h_2}{a}=\cosh \frac{d_1+L\sin \theta }{a}$$$$\frac{h_1}{a}+\frac{h-L\cos \theta }{a}=\cosh \frac{d_1+L\sin \theta }{a}$$$$\sqrt{1+{\tan }^2\varphi }+\frac{h-L\cos \theta }{a}=\cosh (\frac{d_1}{a}+\frac{L\sin \theta }{a})$$$${\sinh }^{-1}\left(\tan \varphi \right)+\frac{2\mu \sin \theta }{\lambda }(\frac{1}{\tan \theta }+\tan \varphi )={\cosh }^{-1}[\sqrt{1+{\tan }^2\varphi }+\frac{2\mu (\eta -\cos \theta )}{\lambda }(\frac{1}{\tan \theta }+\tan \varphi )]$$$$\frac{s_1+b}{a}=\sinh \frac{d_1+L\sin \theta }{a}$$$$\Rightarrow {\sinh }^{-1}\left(\tan \varphi \right)+\frac{2\mu \sin \theta }{\lambda }(\frac{1}{\tan \theta }+\tan \varphi )={\sinh }^{-1}[(1+2\mu )\tan \varphi +\frac{2\mu }{\tan \theta }]$$$$\Rightarrow {\cosh }^{-1}[\sqrt{1+{\tan }^2\varphi }+\frac{2\mu (\eta -\cos \theta )}{\lambda }(\frac{1}{\tan \theta }+\tan \varphi )]={\sinh }^{-1}[(1+2\mu )\tan \varphi +\frac{2\mu }{\tan \theta }]$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com