Math Question and Answers


Question and Answers Forum

All Questions Topic List
Others Questions
Previous in All Question Next in All Question
Previous in Others Next in Others
Question Number 180667 by cortano1 last updated on 15/Nov/22

Commented by JDamian last updated on 15/Nov/22
I never expected this from you :(
	Answered by a.lgnaoui last updated on 15/Nov/22

	Commented by a.lgnaoui last updated on 15/Nov/22

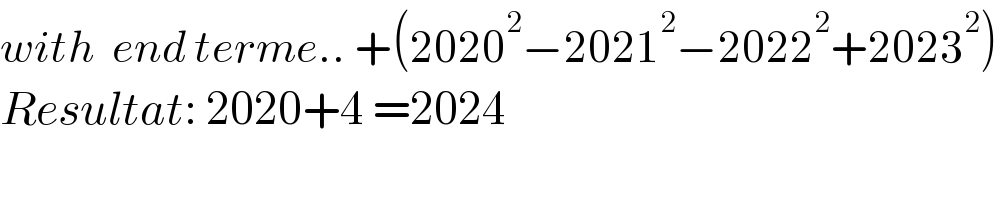
	$w i t h e n d t e r m e . . + (2020^{2} - 2021^{2} - 2022^{2} + 2023^{2})$ $R e s u l t a t : 2020 + 4 = 2024$
	Answered by a.lgnaoui last updated on 15/Nov/22
	$Probleme 5 { ((xy+(x/y)=3p(x^2 +y^2 ) (1))),((xy−(x/y)=p(x^2 +y^2 ) (2))) :} with y≠0 (((1))/((2)))⇒((xy+(x/y))/(xy−(x/y)))=3 ⇒(y^2 +1)=3(y^2 −1) y=±(√2) 1)y=−(√2) x^2 +2+((√2)/(2p)) x=0 x^2 +((x(√2))/(2p))+2 △=(1/(2p^2 ))−8 x_1 =(((√2) (1±(√(1−16p^2 )) ))/(4p)) 2)y=(√2) x(√2) −((√2)/2)x=p(x^2 +2) x^2 −((x(√2))/(2p))+2 x_2 =−x_1 ((−1)/4)<p<(1/4) (2 racines reels) p∈]−∞,((−1)/4)]∪[(1/4),+∞[ (2 racines coppmplexes) Conclusion S={((((√(2()) 1±(√(1−16p^2 )))/(2p)),−(√2) );(−(((√2) (±1(√(1−16p^2 )))/(4p)),+(√2))}$
	$Probleme 5$ ${\begin{cases} xy + \frac{x}{y} = 3 p (x^{2} + y^{2}) (1) \\ xy - \frac{x}{y} = p (x^{2} + y^{2}) (2) \end{cases}$ $with y \neq 0$ $\frac{(1)}{(2)} \Rightarrow \frac{xy + \frac{x}{y}}{xy - \frac{x}{y}} = 3 \Rightarrow (y^{2} + 1) = 3 (y^{2} - 1)$ $y = \pm \sqrt{2}$ $1) y = - \sqrt{2}$ $x^{2} + 2 + \frac{\sqrt{2}}{2 p} x = 0$ $x^{2} + \frac{x \sqrt{2}}{2 p} + 2 △ = \frac{1}{{2 p}^{2}} - 8$ $x_{1} = \frac{\sqrt{2} (1 \pm \sqrt{1 - 16 p^{2}})}{4 p}$ $2) y = \sqrt{2}$ $x \sqrt{2} - \frac{\sqrt{2}}{2} x = p (x^{2} + 2)$ $x^{2} - \frac{x \sqrt{2}}{2 p} + 2 x_{2} = - x_{1}$ $\frac{- 1}{4} < p < \frac{1}{4} (2 r a c i n e s r e e l s)$ $p \in] - \infty, \frac{- 1}{4}] \cup [\frac{1}{4}, + \infty [(2 r a c i n e s c o p p m p l e x e s)$ $C o n c l u s i o n$ $S = {(\frac{\sqrt{2 (} 1 \pm \sqrt{1 - 16 p^{2}}}{2 p}, - \sqrt{2}); (- \frac{\sqrt{2} (\pm 1 \sqrt{1 - 16 p^{2}}}{4 p}, + \sqrt{2})}$
	Answered by a.lgnaoui last updated on 15/Nov/22
	$probleme 5(partie 2) (√(x+(√x) )) (−)(√(x+(√x) )) =(3/2)(√(x/(x+(√x)))) si signe −alors 0=(3/2)(√(x/(x+(√x)))) ⇒(x/(x+(√x)))=0 x=∅(pas de solutions) signe(+) 2(√(x+(√x))) =(3/2)×(√(x/(x+(√x)))) 4(x+(√x))=(9/4)×(x/(x+(√x))) 16(x+(√x) )^2 −9x=0 16x^2 +32x(√x) +7x=0 x+32(√x) +7=0 (avec x≠0) (√x) =t t^2 +32t+7=0 16^2 −7=[15,78]^2 x=−16±(√(249)) =−16±(√(3×83)) ≅−16±9(√3) x={31,78i ; 0,22i}$
	$probleme 5 (partie 2)$ $\sqrt{x + \sqrt{x}} (-) \sqrt{x + \sqrt{x}} = \frac{3}{2} \sqrt{\frac{x}{x + \sqrt{x}}}$ $si signe - alors$ $0 = \frac{3}{2} \sqrt{\frac{x}{x + \sqrt{x}}} \Rightarrow \frac{x}{x + \sqrt{x}} = 0$ $x = \emptyset (p a s d e s o l u t i o n s)$ $s i g n e (+)$ $2 \sqrt{x + \sqrt{x}} = \frac{3}{2} \times \sqrt{\frac{x}{x + \sqrt{x}}}$ $4 (x + \sqrt{x}) = \frac{9}{4} \times \frac{x}{x + \sqrt{x}}$ $16 {(x + \sqrt{x})}^{2} - 9 x = 0$ ${16 x}^{2} + 32 x \sqrt{x} + 7 x = 0$ $x + 32 \sqrt{x} + 7 = 0 (avec x \neq 0)$ $\sqrt{x} = t t^{2} + 32 t + 7 = 0$ $16^{2} - 7 = {[15, 78]}^{2}$ $x = - 16 \pm \sqrt{249} = - 16 \pm \sqrt{3 \times 83} ≅ - 16 \pm 9 \sqrt{3}$ $x = {31, 78 i; 0, 22 i}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum