Math Question and Answers


Question and Answers Forum

All Questions Topic List
Differential Equation Questions
Previous in All Question Next in All Question
Previous in Differential Equation Next in Differential Equation
Question Number 181858 by KINMATICS last updated on 01/Dec/22

	Answered by hmr last updated on 01/Dec/22

	$T h e g e n e r a l s o l u t i o n o f$ $t h e l i n e a r n o n h o m o g e n e o u s$ $e q u a t i o n i s e q u a l t o :$ $(1) g e n e r a l s o l u t i o n o f t h e$ $c o r r e s p o n d i n g h o m o g e n e o u s e q u a t i o n$ $+$ $(2) p a r t i c u l a r s o l u t i o n o f t h e$ $n o n h o m o g e n e o u s e q u a t i o n .$ $(1)$ $y^{″} + 4 y = 0$ $\to r^{2} + 4 = 0 \to r = \pm 2 i$ $y_{h} (x) = c_{1} C o s (2 x) + c_{2} S i n (2 x)$ $(2)$ $y (x) = (A_{1} x + A_{0}) C o s (x) + (B_{1} x + B_{0}) S i n (x)$ $y^{'} (x) = (A_{1}) C o s (x) - (A_{1} x + A_{0}) S i n (x)$ $+ (B_{1}) S i n (x) + (B_{1} x + B_{0}) C o s (x)$ $\to$ $y^{'} (x) = (A_{1} + B_{1} x + B_{0}) C o s (x) + (- A_{1} x - A_{0} + B_{1}) S i n (x)$ $y^{″} (x) = (B_{1}) C o s (x) - (A_{1} + B_{1} x + B_{0}) S i n (x)$ $+ (- A_{1}) S i n (x) + (- A_{1} x - A_{0} + B_{1}) C o s (x)$ $\to$ $y^{″} (x) = (- A_{1} x - A_{0} + 2 B_{1}) C o s (x) - (2 A_{1} + B_{1} x + B_{0}) S i n (x)$ $∙ y^{″} + 4 y = x C o s (x)$ $\to (- A_{1} x - A_{0} + 2 B_{1}) C o s (x) - (2 A_{1} + B_{1} x + B_{0}) S i n (x)$ $+ 4 ((A_{1} x + A_{0}) C o s (x) + (B_{1} x + B_{0}) S i n (x))$ $= x C o s (x)$ $\to$ $(3 A_{1} x + 3 A_{0} + 2 B_{1}) C o s (x) + (- 2 A_{1} + 3 B_{1} x + 3 B_{0}) S i n (x)$ $= x C o s (x)$ $\to A_{0} = B_{1} = 0 A_{1} = \frac{1}{3} B_{0} = \frac{2}{9}$ $\to$ $y (x) = \frac{1}{3} x C o s (x) + \frac{2}{9} S i n (x)$ $t h u s t h e a n s w e r i s :$ $φ (x) = c_{1} C o s (2 x) + c_{2} S i n (2 x) + \frac{1}{3} x C o s (x) + \frac{2}{9} S i n (x)$
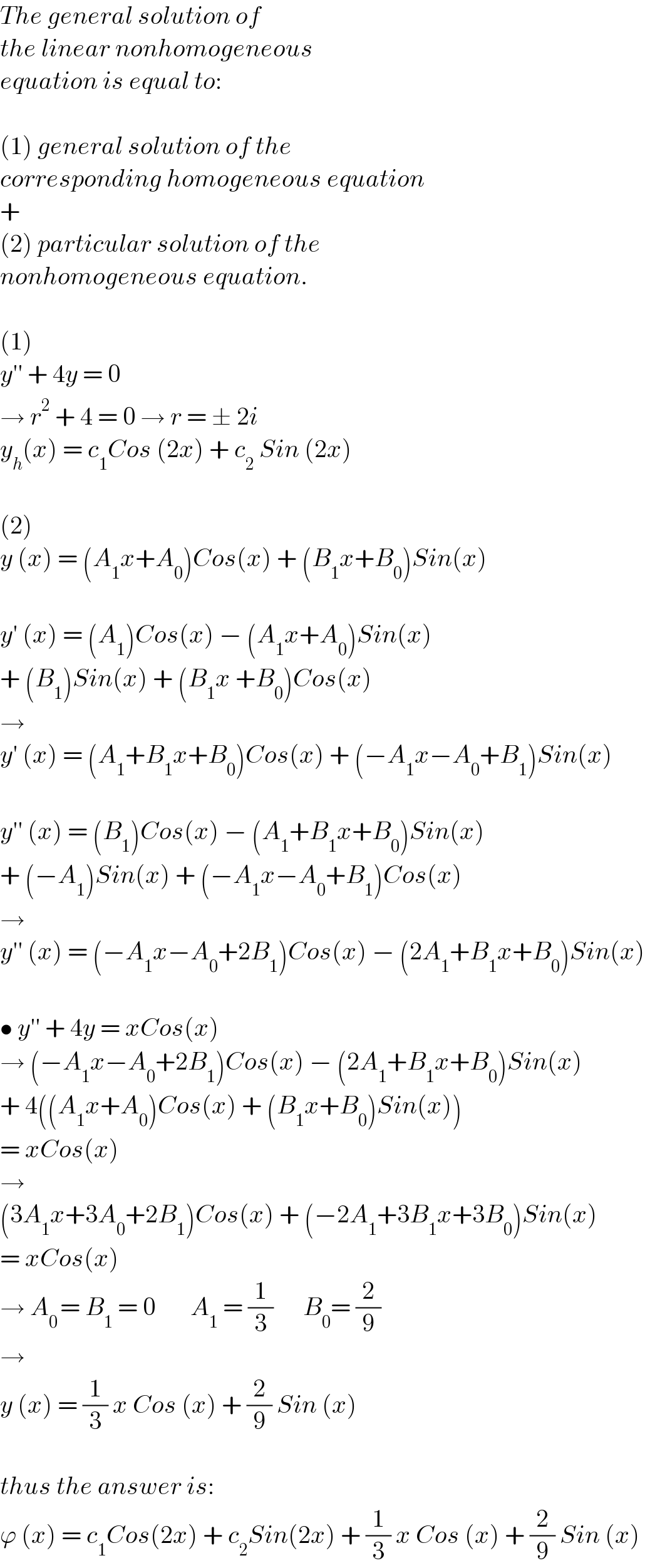
	Commented by hmr last updated on 01/Dec/22

	$φ (x) = c_{1} C o s (2 x) + c_{2} S i n (2 x) + \frac{1}{3} x C o s (x) + \frac{2}{9} S i n (x)$ $∙ φ (0) = 1$ $\to$ $c_{1} = 1$ $∙ ∙ φ (\frac{3 π}{4}) = \frac{- π \sqrt{2}}{8}$ $\to$ $- c_{2} - \frac{π \sqrt{2}}{8} + \frac{\sqrt{2}}{9} = \frac{- π \sqrt{2}}{8}$ $\to$ $c_{2} = \frac{\sqrt{2}}{9}$ $φ (x) = C o s (2 x) + \frac{\sqrt{2}}{9} S i n (2 x) + \frac{1}{3} x C o s (x) + \frac{2}{9} S i n (x)$ $1 - \frac{π}{3}$ $φ (π) + φ (- π) = (1 - \frac{π}{3}) + (1 + \frac{π}{3}) = 2$
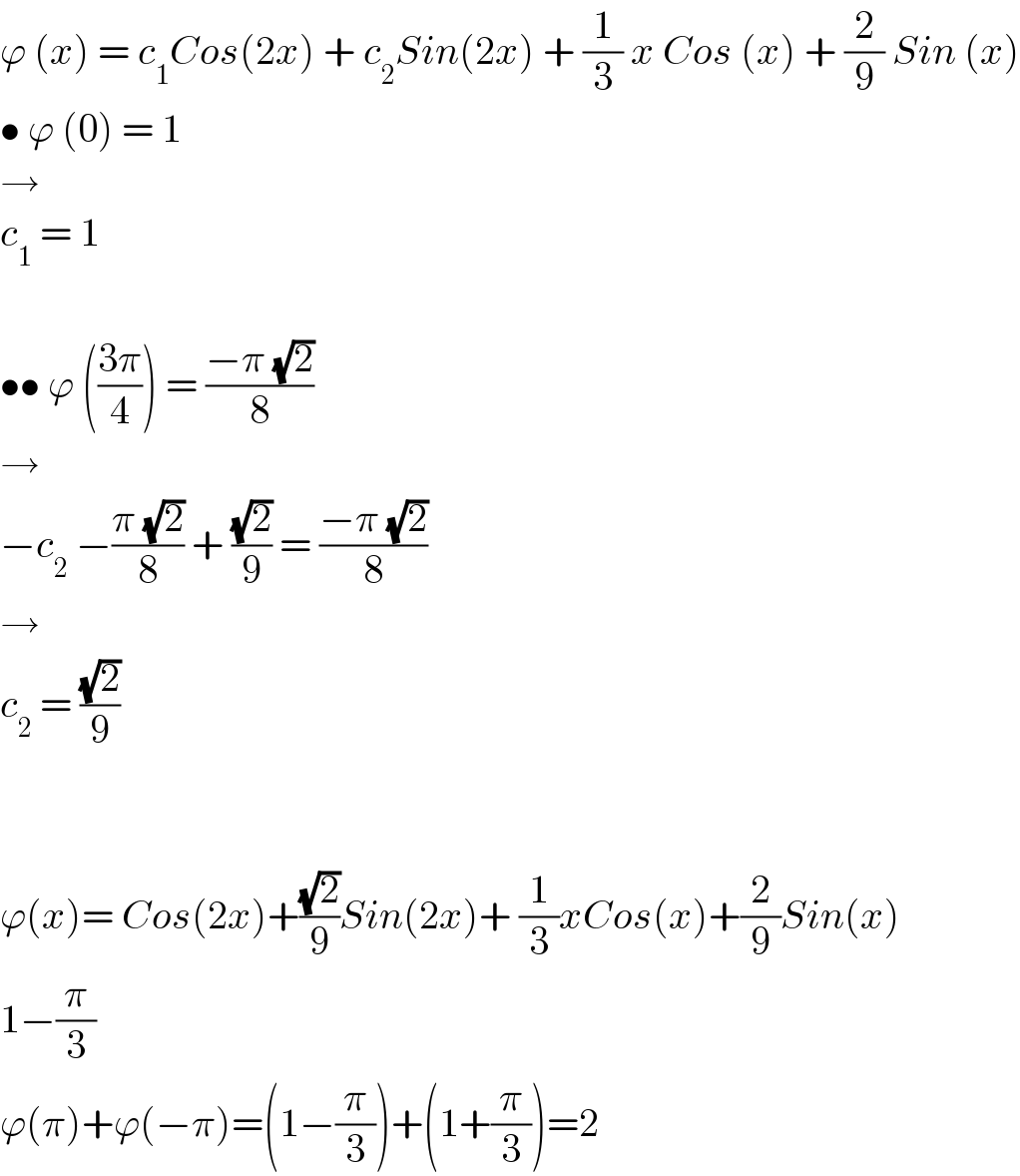
	Answered by qaz last updated on 01/Dec/22

	$y_{p} = \frac{1}{D^{2} + 4} x \cos x = (x - \frac{2 D}{D^{2} + 4}) \frac{1}{D^{2} + 4} \cos x$ $= (x - \frac{2 D}{D^{2} + 4}) \frac{1}{3} \cos x = \frac{1}{3} x \cos x + \frac{2}{9} \sin x$ $\Rightarrow y = C_{1} \sin 2 x + C_{2} \cos 2 x + \frac{1}{3} x \cos x + \frac{2}{9} \sin x$ $y (0) = C_{2} = 1$ $y (\frac{3 π}{4}) = - C_{1} - \frac{π}{4 \sqrt{2}} + \frac{\sqrt{2}}{9} = - \frac{π}{4 \sqrt{2}} \Rightarrow C_{1} = \frac{\sqrt{2}}{9}$ $\Rightarrow y = \frac{\sqrt{2}}{9} \sin 2 x + \cos 2 x + \frac{1}{3} x \cos x + \frac{2}{9} \sin x$ $\Rightarrow y (π) + y (- π) = \frac{\sqrt{2}}{9}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum