Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 23472 by ajfour last updated on 31/Oct/17

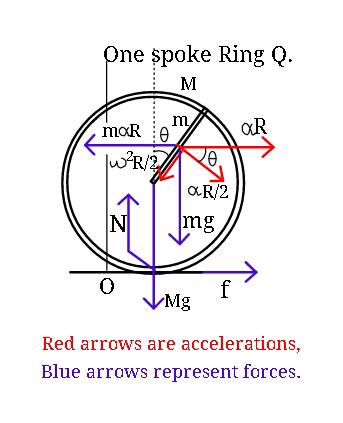
Commented by ajfour last updated on 31/Oct/17

$$Q.23390(continued..)$$

Answered by ajfour last updated on 31/Oct/17

$$Applying\mathrm{\Sigma }{F}_x=\mathrm{\Sigma }{ma}_{x}fromthe$$$$groundframe:$$$$f=(M+m)\left(\alpha R\right)+\frac{m\left(\alpha R\right)\cos \theta }{2}$$$$-m{\omega }^2\left(\frac{R}{2}\right)\sin \theta ...\left(i\right)$$$$Applying\mathrm{\Sigma }\tau =I_{net}\alpha fromthe$$$$frameofcentreofring:$$$$\frac{mgR\sin \theta }{2}-fR-\left(m\alpha R\right)\left(\frac{R}{2}\cos \theta \right)$$$$=({MR}^2+\frac{{mR}^2}{3})\alpha ...\left(ii\right)$$$$eliminating\mathit{f}from\left(i\right)and\left(ii\right):$$$$\frac{mg\sin \theta }{2}-(M+m)\left(\alpha R\right)$$$$-\frac{m\left(\alpha R\right)\cos \theta }{2}+\frac{m{\omega }^{2}R\sin \theta }{2}$$$$-\frac{m\left(\alpha R\right)\cos \theta }{2}=(M+\frac{m}{3})\left(\alpha R\right)$$$$\Rightarrow \left(\alpha R\right)[M+\frac{m}{3}+M+m+m\cos \theta ]$$$$=\frac{m\sin \theta }{2}({\omega }^{2}R+g)$$$$\Rightarrow \alpha =\frac{m({\omega }^2+g/R)\sin \theta }{2[2M+\frac{4m}{3}+m\cos \theta ]}$$$$\Rightarrow \frac{\omega d\omega }{d\theta }=\frac{m({\omega }^2+\frac{g}{R})\sin \theta }{2[2M+\frac{4m}{3}+m\cos \theta ]}$$$${\int }_0^{\omega }\frac{2\omega d\omega }{{\omega }^2+\frac{g}{R}}={\int }_0^{\theta }\frac{m\sin \theta }{2M+\frac{4m}{3}+m\cos \theta }$$$$\ln \left(\frac{{\omega }^2+\frac{g}{R}}{\frac{g}{R}}\right)=\ln \left(\frac{2M+\frac{4m}{3}+m}{2M+\frac{4m}{3}+m\cos \theta }\right)$$$$\frac{{\omega }^{2}R}{g}+1=1-\frac{m(1-\cos \theta )}{2M+\frac{4m}{3}+m\cos \theta }$$$${\mathit{\omega }}^2=\frac{\mathit{g}}{\mathit{R}}\left(\frac{1-\cos \mathit{\theta }}{\frac{2\mathit{M}}{\mathit{m}}+\frac{4}{3}+\cos \mathit{\theta }}\right)$$$$And\mathit{\alpha }=\frac{1}{2}\frac{\mathit{d}\left({\mathit{\omega }}^2\right)}{\mathit{d}\mathit{\theta }}.$$$$Toobtainthetimeforthereturn,$$$$\Rightarrow \mathit{\omega }=\frac{\mathit{d}\mathit{\theta }}{\mathit{d}\mathit{t}}=\sqrt{\frac{g}{R}}{\left[\frac{1-\cos \theta }{b^2+\cos \theta }\right]}^{1/2}$$$$\Rightarrow T=4\sqrt{\frac{R}{g}}{\int }_0^{\pi }{\left[\frac{b^2+\cos \theta }{1-\cos \theta }\right]}^{1/2}d\theta$$$$where{b}^2=\frac{2M}{m}+\frac{4}{3}.$$$$....mightcontinue...$$

Commented by mrW1 last updated on 31/Oct/17

$$\mathrm{how}\mathrm{is}\mathrm{it}\mathrm{to}\mathrm{explain},\mathrm{that}\mathrm{the}\mathrm{time}$$$$\mathrm{from}\theta =0\mathrm{is}\mathrm{not}\mathrm{convergent}?$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com