
Question and Answers Forum
Question Number 51691 by peter frank last updated on 29/Dec/18

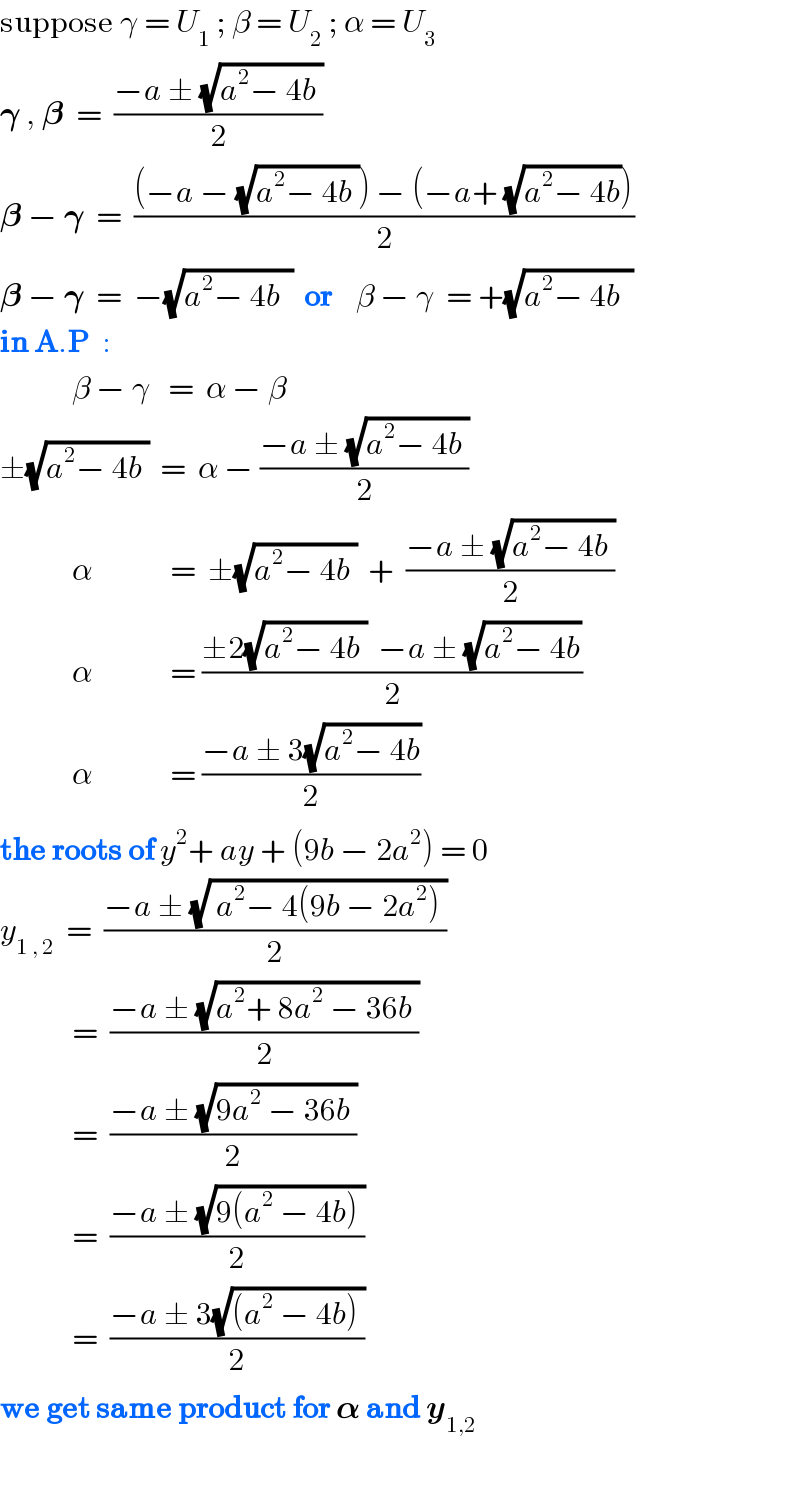
Answered by afachri last updated on 29/Dec/18
| ||
Question and Answers Forum | ||
Question Number 51691 by peter frank last updated on 29/Dec/18 | ||
 | ||
Answered by afachri last updated on 29/Dec/18 | ||
 | ||
| ||