Math Question and Answers


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 61208 by Tawa1 last updated on 30/May/19

Commented by maxmathsup by imad last updated on 30/May/19
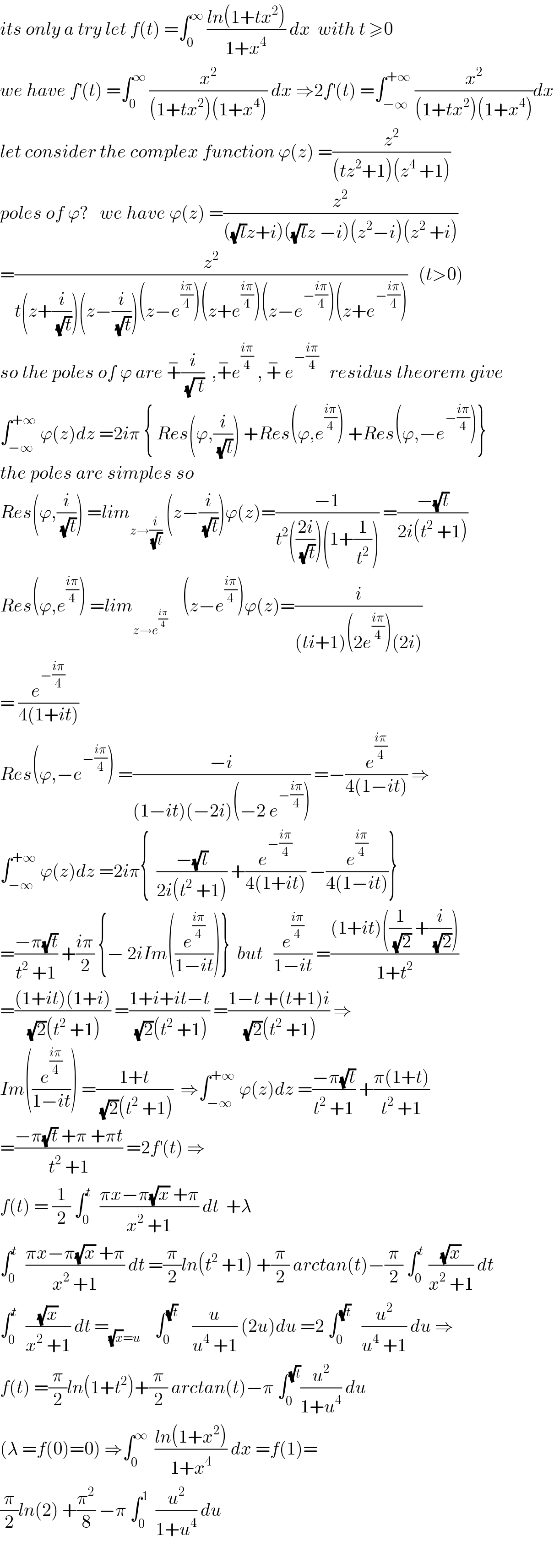
$its only a try let f(t) =∫_0 ^∞ ((ln(1+tx^2 ))/(1+x^4 )) dx with t ≥0 we have f^′ (t) =∫_0 ^∞ (x^2 /((1+tx^2 )(1+x^4 ))) dx ⇒2f^′ (t) =∫_(−∞) ^(+∞) (x^2 /((1+tx^2 )(1+x^4 )))dx let consider the complex function ϕ(z) =(z^2 /((tz^2 +1)(z^4 +1))) poles of ϕ? we have ϕ(z) =(z^2 /(((√t)z+i)((√t)z −i)(z^2 −i)(z^2 +i))) =(z^2 /(t(z+(i/(√t)))(z−(i/(√t)))(z−e^((iπ)/4) )(z+e^((iπ)/4) )(z−e^(−((iπ)/4)) )(z+e^(−((iπ)/4)) ))) (t>0) so the poles of ϕ are +^− (i/(√( t))) ,+^− e^((iπ)/4) , +^− e^(−((iπ)/4)) residus theorem give ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ { Res(ϕ,(i/(√t))) +Res(ϕ,e^((iπ)/4) ) +Res(ϕ,−e^(−((iπ)/4)) )} the poles are simples so Res(ϕ,(i/(√t))) =lim_(z→(i/(√t))) (z−(i/(√t)))ϕ(z)=((−1)/(t^2 (((2i)/(√t)))(1+(1/t^2 )))) =((−(√t))/(2i(t^2 +1))) Res(ϕ,e^((iπ)/4) ) =lim_(z→e^((iπ)/4) ) (z−e^((iπ)/4) )ϕ(z)=(i/((ti+1)(2e^((iπ)/4) )(2i))) = (e^(−((iπ)/4)) /(4(1+it))) Res(ϕ,−e^(−((iπ)/4)) ) =((−i)/((1−it)(−2i)(−2 e^(−((iπ)/4)) ))) =−(e^((iπ)/4) /(4(1−it))) ⇒ ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ{ ((−(√t))/(2i(t^2 +1))) +(e^(−((iπ)/4)) /(4(1+it))) −(e^((iπ)/4) /(4(1−it)))} =((−π(√t))/(t^2 +1)) +((iπ)/2) {− 2iIm((e^((iπ)/4) /(1−it)))} but (e^((iπ)/4) /(1−it)) =(((1+it)((1/(√2)) +(i/(√2))))/(1+t^2 )) =(((1+it)(1+i))/((√2)(t^2 +1))) =((1+i+it−t)/((√2)(t^2 +1))) =((1−t +(t+1)i)/((√2)(t^2 +1))) ⇒ Im((e^((iπ)/4) /(1−it))) =((1+t)/((√2)(t^2 +1))) ⇒∫_(−∞) ^(+∞) ϕ(z)dz =((−π(√t))/(t^2 +1)) +((π(1+t))/(t^2 +1)) =((−π(√t) +π +πt)/(t^2 +1)) =2f^′ (t) ⇒ f(t) = (1/2) ∫_0 ^t ((πx−π(√x) +π)/(x^2 +1)) dt +λ ∫_0 ^t ((πx−π(√x) +π)/(x^2 +1)) dt =(π/2)ln(t^2 +1) +(π/2) arctan(t)−(π/2) ∫_0 ^t ((√x)/(x^2 +1)) dt ∫_0 ^t ((√x)/(x^2 +1)) dt =_((√x)=u) ∫_0 ^(√t) (u/(u^4 +1)) (2u)du =2 ∫_0 ^(√t) (u^2 /(u^4 +1)) du ⇒ f(t) =(π/2)ln(1+t^2 )+(π/2) arctan(t)−π ∫_0 ^(√t) (u^2 /(1+u^4 )) du (λ =f(0)=0) ⇒∫_0 ^∞ ((ln(1+x^2 ))/(1+x^4 )) dx =f(1)= (π/2)ln(2) +(π^2 /8) −π ∫_0 ^1 (u^2 /(1+u^4 )) du$
$i t s o n l y a t r y l e t f (t) = \int_{0}^{\infty} \frac{l n (1 + {t x}^{2})}{1 + x^{4}} d x w i t h t ⩾ 0$ $w e h a v e f^{^{'}} (t) = \int_{0}^{\infty} \frac{x^{2}}{(1 + {t x}^{2}) (1 + x^{4})} d x \Rightarrow 2 f^{^{'}} (t) = \int_{- \infty}^{+ \infty} \frac{x^{2}}{(1 + {t x}^{2}) (1 + x^{4})} d x$ $l e t c o n s i d e r t h e c o m p l e x f u n c t i o n φ (z) = \frac{z^{2}}{({t z}^{2} + 1) (z^{4} + 1)}$ $p o l e s o f φ ? w e h a v e φ (z) = \frac{z^{2}}{(\sqrt{t} z + i) (\sqrt{t} z - i) (z^{2} - i) (z^{2} + i)}$ $= \frac{z^{2}}{t (z + \frac{i}{\sqrt{t}}) (z - \frac{i}{\sqrt{t}}) (z - e^{\frac{i π}{4}}) (z + e^{\frac{i π}{4}}) (z - e^{- \frac{i π}{4}}) (z + e^{- \frac{i π}{4}})} (t > 0)$ $s o t h e p o l e s o f φ a r e \bar{+} \frac{i}{\sqrt{t}}, \bar{+} e^{\frac{i π}{4}}, \bar{+} e^{- \frac{i π}{4}} r e s i d u s t h e o r e m g i v e$ $\int_{- \infty}^{+ \infty} φ (z) d z = 2 i π {R e s (φ, \frac{i}{\sqrt{t}}) + R e s (φ, e^{\frac{i π}{4}}) + R e s (φ, - e^{- \frac{i π}{4}})}$ $t h e p o l e s a r e s i m p l e s s o$ $R e s (φ, \frac{i}{\sqrt{t}}) = {l i m}_{z \to \frac{i}{\sqrt{t}}} (z - \frac{i}{\sqrt{t}}) φ (z) = \frac{- 1}{t^{2} (\frac{2 i}{\sqrt{t}}) (1 + \frac{1}{t^{2}})} = \frac{- \sqrt{t}}{2 i (t^{2} + 1)}$ $R e s (φ, e^{\frac{i π}{4}}) = {l i m}_{z \to e^{\frac{i π}{4}}} (z - e^{\frac{i π}{4}}) φ (z) = \frac{i}{(t i + 1) (2 e^{\frac{i π}{4}}) (2 i)}$ $= \frac{e^{- \frac{i π}{4}}}{4 (1 + i t)}$ $R e s (φ, - e^{- \frac{i π}{4}}) = \frac{- i}{(1 - i t) (- 2 i) (- 2 e^{- \frac{i π}{4}})} = - \frac{e^{\frac{i π}{4}}}{4 (1 - i t)} \Rightarrow$ $\int_{- \infty}^{+ \infty} φ (z) d z = 2 i π {\frac{- \sqrt{t}}{2 i (t^{2} + 1)} + \frac{e^{- \frac{i π}{4}}}{4 (1 + i t)} - \frac{e^{\frac{i π}{4}}}{4 (1 - i t)}}$ $= \frac{- π \sqrt{t}}{t^{2} + 1} + \frac{i π}{2} {- 2 i I m (\frac{e^{\frac{i π}{4}}}{1 - i t})} b u t \frac{e^{\frac{i π}{4}}}{1 - i t} = \frac{(1 + i t) (\frac{1}{\sqrt{2}} + \frac{i}{\sqrt{2}})}{1 + t^{2}}$ $= \frac{(1 + i t) (1 + i)}{\sqrt{2} (t^{2} + 1)} = \frac{1 + i + i t - t}{\sqrt{2} (t^{2} + 1)} = \frac{1 - t + (t + 1) i}{\sqrt{2} (t^{2} + 1)} \Rightarrow$ $I m (\frac{e^{\frac{i π}{4}}}{1 - i t}) = \frac{1 + t}{\sqrt{2} (t^{2} + 1)} \Rightarrow \int_{- \infty}^{+ \infty} φ (z) d z = \frac{- π \sqrt{t}}{t^{2} + 1} + \frac{π (1 + t)}{t^{2} + 1}$ $= \frac{- π \sqrt{t} + π + π t}{t^{2} + 1} = 2 f^{^{'}} (t) \Rightarrow$ $f (t) = \frac{1}{2} \int_{0}^{t} \frac{π x - π \sqrt{x} + π}{x^{2} + 1} d t + λ$ $\int_{0}^{t} \frac{π x - π \sqrt{x} + π}{x^{2} + 1} d t = \frac{π}{2} l n (t^{2} + 1) + \frac{π}{2} a r c t a n (t) - \frac{π}{2} \int_{0}^{t} \frac{\sqrt{x}}{x^{2} + 1} d t$ $\int_{0}^{t} \frac{\sqrt{x}}{x^{2} + 1} d t =_{\sqrt{x} = u} \int_{0}^{\sqrt{t}} \frac{u}{u^{4} + 1} (2 u) d u = 2 \int_{0}^{\sqrt{t}} \frac{u^{2}}{u^{4} + 1} d u \Rightarrow$ $f (t) = \frac{π}{2} l n (1 + t^{2}) + \frac{π}{2} a r c t a n (t) - π \int_{0}^{\sqrt{t}} \frac{u^{2}}{1 + u^{4}} d u$ $(λ = f (0) = 0) \Rightarrow \int_{0}^{\infty} \frac{l n (1 + x^{2})}{1 + x^{4}} d x = f (1) =$ $\frac{π}{2} l n (2) + \frac{π^{2}}{8} - π \int_{0}^{1} \frac{u^{2}}{1 + u^{4}} d u$
Commented by maxmathsup by imad last updated on 30/May/19
$let decompose F(u) =(u^2 /(u^4 +1)) ⇒F(u) =(u^2 /((u^2 +1)^2 −2u^2 )) =(u^2 /((u^2 +(√2)u +1)(u^2 −(√2)u +1))) =((au +b)/(u^2 +(√2)u +1)) +((cu +d)/(u^2 −(√2)u +1)) F(−u) =F(u) ⇒((−au +b)/(u^2 −(√2)u +1)) +((−cu +d)/(u^2 +(√2)u +1)) =F(u) ⇒c=−a and b=d ⇒F(u) =((au +b)/(u^2 +(√2)u +1)) +((−au +b)/(u^2 −(√2)u +1)) F(0) =0 =2b ⇒b=0 F(1) =(1/2) = (a/(2+(√2))) −(a/(2−(√2))) =(((2−(√2)−2−(√2))a)/2) ⇒1=−2(√2)a ⇒ a =−(1/(2(√2))) ⇒F(u) =−(1/(2(√2))) (u/(u^2 +(√2)u +1)) +(1/(2(√2))) (u/(u^2 −(√2)u +1)) ⇒ ∫_0 ^1 F(u)du =−(1/(4(√2))) ∫_0 ^1 ((2u+(√2)−(√2))/(u^2 +(√2)u +1)) du +(1/(4(√2))) ∫_0 ^1 ((2u−(√2) +(√2))/(u^2 −(√2) u +1))du =−(1/(4(√2)))[ln(u^2 +(√2)u +1)]_0 ^1 +(1/4) ∫_0 ^1 (du/(u^2 +(√2)u +1)) +(1/(4(√2)))[ln(u^2 −(√2)u +1)]_0 ^1 +(1/4) ∫_0 ^1 (du/(u^2 −(√2)u +1)) ∫_0 ^1 (du/(u^2 +(√2)u +1)) =∫_0 ^1 (du/(u^2 +2(1/(√2))u +(1/2) +(1/2))) =∫_0 ^1 (du/((u+(1/(√2)))^2 +(1/2))) =_(u+(1/(√2))=(t/(√2))) ∫_1 ^((√2) +1) (dt/((√2)((1/2))(1+t^2 ))) =(√2) [arctan(t)]_1 ^((√2) +1) =(√2){ arctan(1+(√2))−(π/4)} ∫_0 ^1 (du/(u^2 −(√2)u +1)) =∫_0 ^1 (du/((u−(1/(√2)))^2 +(1/2))) =_(u−(1/(√2))=(t/(√2))) ∫_(−1) ^((√2)−1) (dt/((√2)((1/2))(1+t^2 ))) =(√2) [arctan(t)]_(−1) ^((√2)−1) =(√2){ arctan((√2)−1)+(π/4)} ⇒ ∫_0 ^1 F(u)du =−(1/(4(√2)))ln(2+(√2)) +(1/(4(√2)))ln(2−(√2))+((√2)/4){arctan(1+(√2))−(π/4)} +((√2)/4){arctan((√2)−1)−(π/4)} ⇒ ∫_0 ^∞ ((ln(1+x^2 ))/(1+x^4 )) dx =(π/2)ln(2)+(π^2 /8) +(π/(4(√2))){ln(((2−(√2))/(2+(√2))))} −π((√2)/4){ arctan((√2) +1) +arctan((√2) −1)−(π/2)} .$
$l e t d e c o m p o s e F (u) = \frac{u^{2}}{u^{4} + 1} \Rightarrow F (u) = \frac{u^{2}}{{(u^{2} + 1)}^{2} - 2 u^{2}}$ $= \frac{u^{2}}{(u^{2} + \sqrt{2} u + 1) (u^{2} - \sqrt{2} u + 1)} = \frac{a u + b}{u^{2} + \sqrt{2} u + 1} + \frac{c u + d}{u^{2} - \sqrt{2} u + 1}$ $F (- u) = F (u) \Rightarrow \frac{- a u + b}{u^{2} - \sqrt{2} u + 1} + \frac{- c u + d}{u^{2} + \sqrt{2} u + 1} = F (u) \Rightarrow c = - a a n d$ $b = d \Rightarrow F (u) = \frac{a u + b}{u^{2} + \sqrt{2} u + 1} + \frac{- a u + b}{u^{2} - \sqrt{2} u + 1}$ $F (0) = 0 = 2 b \Rightarrow b = 0$ $F (1) = \frac{1}{2} = \frac{a}{2 + \sqrt{2}} - \frac{a}{2 - \sqrt{2}} = \frac{(2 - \sqrt{2} - 2 - \sqrt{2}) a}{2} \Rightarrow 1 = - 2 \sqrt{2} a \Rightarrow$ $a = - \frac{1}{2 \sqrt{2}} \Rightarrow F (u) = - \frac{1}{2 \sqrt{2}} \frac{u}{u^{2} + \sqrt{2} u + 1} + \frac{1}{2 \sqrt{2}} \frac{u}{u^{2} - \sqrt{2} u + 1} \Rightarrow$ $\int_{0}^{1} F (u) d u = - \frac{1}{4 \sqrt{2}} \int_{0}^{1} \frac{2 u + \sqrt{2} - \sqrt{2}}{u^{2} + \sqrt{2} u + 1} d u + \frac{1}{4 \sqrt{2}} \int_{0}^{1} \frac{2 u - \sqrt{2} + \sqrt{2}}{u^{2} - \sqrt{2} u + 1} d u$ $= - \frac{1}{4 \sqrt{2}} {[l n (u^{2} + \sqrt{2} u + 1)]}_{0}^{1} + \frac{1}{4} \int_{0}^{1} \frac{d u}{u^{2} + \sqrt{2} u + 1}$ $+ \frac{1}{4 \sqrt{2}} {[l n (u^{2} - \sqrt{2} u + 1)]}_{0}^{1} + \frac{1}{4} \int_{0}^{1} \frac{d u}{u^{2} - \sqrt{2} u + 1}$ $\int_{0}^{1} \frac{d u}{u^{2} + \sqrt{2} u + 1} = \int_{0}^{1} \frac{d u}{u^{2} + 2 \frac{1}{\sqrt{2}} u + \frac{1}{2} + \frac{1}{2}} = \int_{0}^{1} \frac{d u}{{(u + \frac{1}{\sqrt{2}})}^{2} + \frac{1}{2}}$ $=_{u + \frac{1}{\sqrt{2}} = \frac{t}{\sqrt{2}}} \int_{1}^{\sqrt{2} + 1} \frac{d t}{\sqrt{2} (\frac{1}{2}) (1 + t^{2})} = \sqrt{2} {[a r c t a n (t)]}_{1}^{\sqrt{2} + 1}$ $= \sqrt{2} {a r c t a n (1 + \sqrt{2}) - \frac{π}{4}}$ $\int_{0}^{1} \frac{d u}{u^{2} - \sqrt{2} u + 1} = \int_{0}^{1} \frac{d u}{{(u - \frac{1}{\sqrt{2}})}^{2} + \frac{1}{2}} =_{u - \frac{1}{\sqrt{2}} = \frac{t}{\sqrt{2}}} \int_{- 1}^{\sqrt{2} - 1} \frac{d t}{\sqrt{2} (\frac{1}{2}) (1 + t^{2})}$ $= \sqrt{2} {[a r c t a n (t)]}_{- 1}^{\sqrt{2} - 1} = \sqrt{2} {a r c t a n (\sqrt{2} - 1) + \frac{π}{4}} \Rightarrow$ $\int_{0}^{1} F (u) d u = - \frac{1}{4 \sqrt{2}} l n (2 + \sqrt{2}) + \frac{1}{4 \sqrt{2}} l n (2 - \sqrt{2}) + \frac{\sqrt{2}}{4} {a r c t a n (1 + \sqrt{2}) - \frac{π}{4}}$ $+ \frac{\sqrt{2}}{4} {a r c t a n (\sqrt{2} - 1) - \frac{π}{4}} \Rightarrow$ $\int_{0}^{\infty} \frac{l n (1 + x^{2})}{1 + x^{4}} d x = \frac{π}{2} l n (2) + \frac{π^{2}}{8} + \frac{π}{4 \sqrt{2}} {l n (\frac{2 - \sqrt{2}}{2 + \sqrt{2}})}$ $- π \frac{\sqrt{2}}{4} {a r c t a n (\sqrt{2} + 1) + a r c t a n (\sqrt{2} - 1) - \frac{π}{2}} .$
Commented by ajfour last updated on 30/May/19

$C o n g r a t u l a t i o n s S i r .$ $W o n d e r f u l s p i r i t .$
Commented by Tawa1 last updated on 30/May/19

$Wow . God bless you sir .$
Commented by Tawa1 last updated on 30/May/19

$I appreciate your time .$
Commented by maxmathsup by imad last updated on 30/May/19

$y o u a r e w e l c o m e s i r .$
Commented by perlman last updated on 30/May/19

$v e r r y g o o d w o r c k!$
Commented by maxmathsup by imad last updated on 31/May/19

$t h a n k s s i r .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum