Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 64185 by Hope last updated on 15/Jul/19

Commented by Hope last updated on 15/Jul/19

$$allquestionbasedonTito-lemmainequality$$

Commented by Tony Lin last updated on 15/Jul/19

$$a+b+c=1$$$$\Rightarrow provethat\frac{1}{1-c}+\frac{16(1-c)}{c}⩾1,0<c<1$$$$\frac{\frac{1}{1-c}+\frac{16(1-c)}{c}}{2}⩾\frac{4}{\sqrt{c}}$$$$\Rightarrow \frac{1}{1-c}+\frac{16(1-c)}{c}⩾\frac{8}{\sqrt{c}}⩾1$$$$\Rightarrow \frac{1}{a+b}+\frac{16}{c}+\frac{81}{a+b+c}⩾98$$

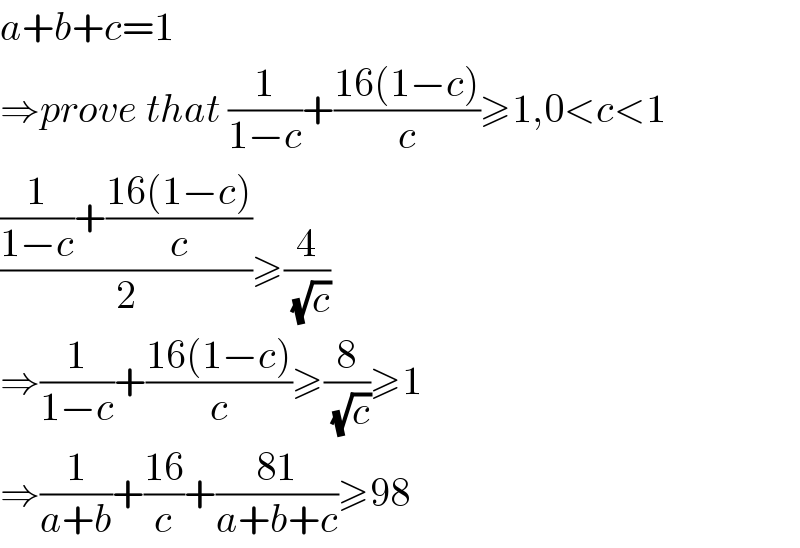
Commented by Hope last updated on 15/Jul/19

$$thankyousirbothofyou$$

Answered by MJS last updated on 15/Jul/19

$$c=1-a-b>0$$$$\frac{1}{a+b}-\frac{16}{a+b-1}⩾17[\times (a+b)>0;\times (a+b-1)<0$$$$-(15a+15b+1)⩽17(a+b)(a+b-1)$$$$17a^2+34ab+17b^2-2a-2b+1⩾0$$$$a=1-b-c(\mathrm{or}b=1-a-c)$$$$17c^2-32c+16⩾0$$$$\min (17c^2-32c+16)=\frac{16}{17}⩾0$$$$\mathrm{hence}\mathrm{proved}$$

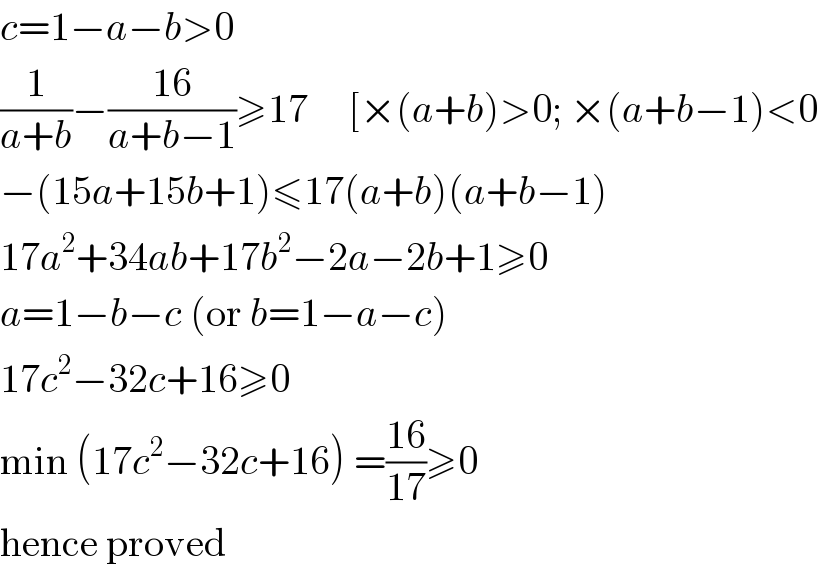
Commented by Hope last updated on 15/Jul/19

$$thankyousir...$$

Commented by Hope last updated on 15/Jul/19

Answered by Hope last updated on 15/Jul/19

$$\frac{1}{a+b}+\frac{16}{c}+\frac{81}{a+b+c}$$$$\frac{1^2}{a+b}+\frac{4^2}{c}+\frac{9^2}{a+b+c}⩾\frac{{(1+4+9)}^2}{2(a+b+c)}$$$$\frac{1^2}{a+b}+\frac{4^2}{c}+\frac{9^2}{a+b+c}⩾\frac{196}{2}=98$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com