
Question and Answers Forum
Question Number 65723 by bshahid010@gmail.com last updated on 02/Aug/19

Answered by Tanmay chaudhury last updated on 03/Aug/19
![7a=2π 4a=2π−3a cos(4a)=cos(2π−3a) (2cos^2 2a−1)=cos3π cosa=c so cos2a=2c^2 −1 cos3a=4c^3 −3c {2(2c^2 −1)^2 −1}=(4c^3 −3c) {2(4c^4 −4c^2 +1)−1}=(4c^3 −3c) 8c^4 −8c^2 +1=4c^3 −3c 8c^4 −4c^3 −8c^2 +3c+1=0 8c^4 −8c^3 +4c^3 −4c^2 −4c^2 +4c−c+1=0 8c^3 (c−1)+4c^2 (c−1)−4c(c−1)−1(c−1)=0 (c−1)(8c^3 +4c^2 −4c−1)=0 8c^3 +4c^2 −4c−1=0 c=cosa=cos(((2π)/7)) [since 7a=2π] cos(((2π)/7)) is a root of 8x^3 +4x^2 −4x−1=0](Q65744.png)
Commented by bshahid010@gmail.com last updated on 03/Aug/19

Commented by Tanmay chaudhury last updated on 03/Aug/19

Commented by MJS last updated on 03/Aug/19

Commented by MJS last updated on 05/Aug/19
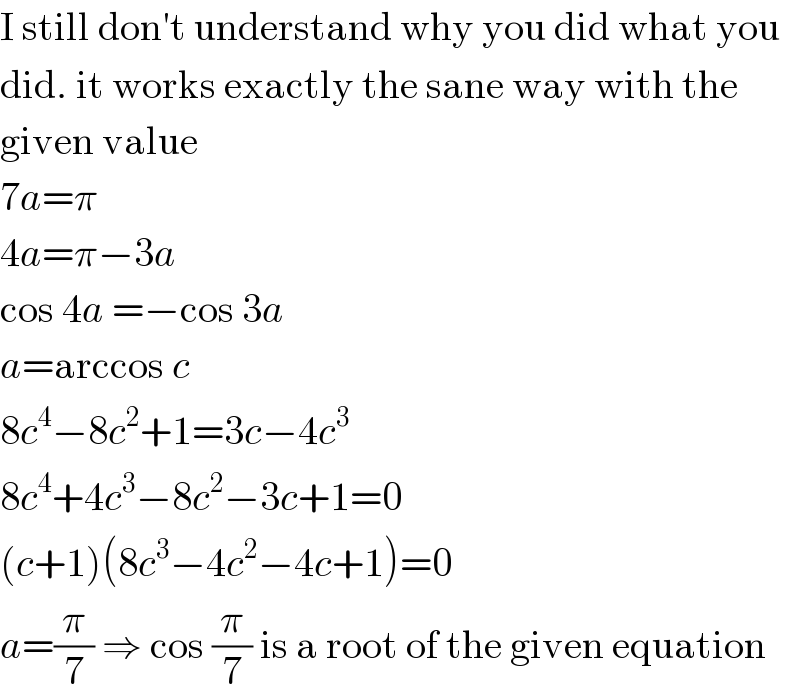
Commented by Tanmay chaudhury last updated on 05/Aug/19

