Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 68309 by ajfour last updated on 08/Sep/19

Commented by ajfour last updated on 08/Sep/19

$$FindABandACintermsofa$$$$and\theta .$$

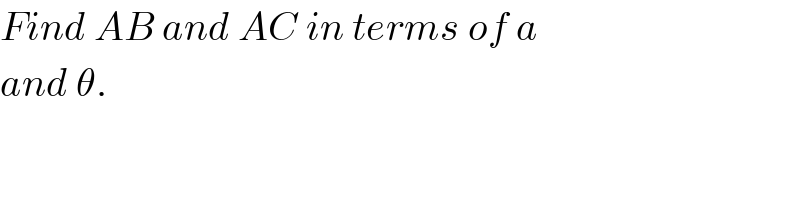
Commented by mr W last updated on 08/Sep/19

$$\frac{AB}{\sin \theta }=\frac{AC}{\sin 2\theta }=\frac{a}{\sin 3\theta }$$$$\Rightarrow AB=\frac{a\sin \theta }{\sin 3\theta }=\frac{a}{3-4{\sin }^2\theta }$$$$\Rightarrow AC=\frac{a\sin 2\theta }{\sin 3\theta }=\frac{2a\cos \theta }{3-4{\sin }^2\theta }$$

Commented by ajfour last updated on 09/Sep/19

$$ThankyouSir.Ishallfinda$$$$betterquestion.$$

Answered by ajfour last updated on 09/Sep/19

$$letAC=apandAB=q$$$$\frac{ap}{\sin 2\theta }=\frac{q}{\sin \theta }\Rightarrow q=\frac{ap}{2\cos \theta }$$$$\cos (\pi -3\theta )=\frac{(\frac{p^2}{4\cos {}^2\theta }+p^2-1)}{\left(\frac{2p^2}{2\cos \theta }\right)}$$$$\Rightarrow (3-4\cos {}^2\theta )p^2=p^2+\frac{p^2}{4\cos {}^2\theta }-1$$$$\Rightarrow \frac{1}{p^2}=\frac{1}{4\cos {}^2\theta }+4\cos {}^2\theta -2$$$$If0<\theta <\frac{\pi }{2}$$$$\Rightarrow \frac{1}{p}=\frac{1}{2\cos \theta }-2\cos \theta =\frac{1-4\cos {}^2\theta }{2\cos \theta }$$$$asAC=apandAB=\frac{ap}{2\cos \theta }$$$$so\mathit{A}\mathit{C}=\frac{2a\cos \theta }{1-4\cos {}^2\theta };\mathit{A}\mathit{B}=\frac{a}{1-4\cos {}^2\theta }$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com