Question and Answers Forum
Question Number 83824 by Power last updated on 06/Mar/20
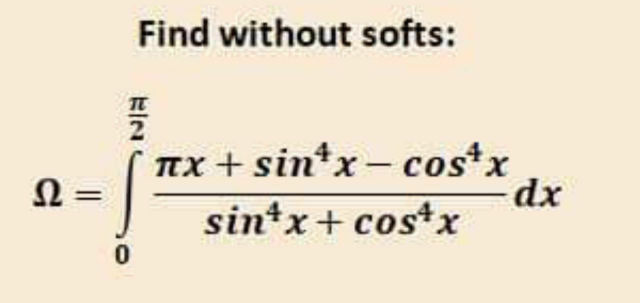
Commented by mathmax by abdo last updated on 06/Mar/20
Answered by TANMAY PANACEA last updated on 06/Mar/20
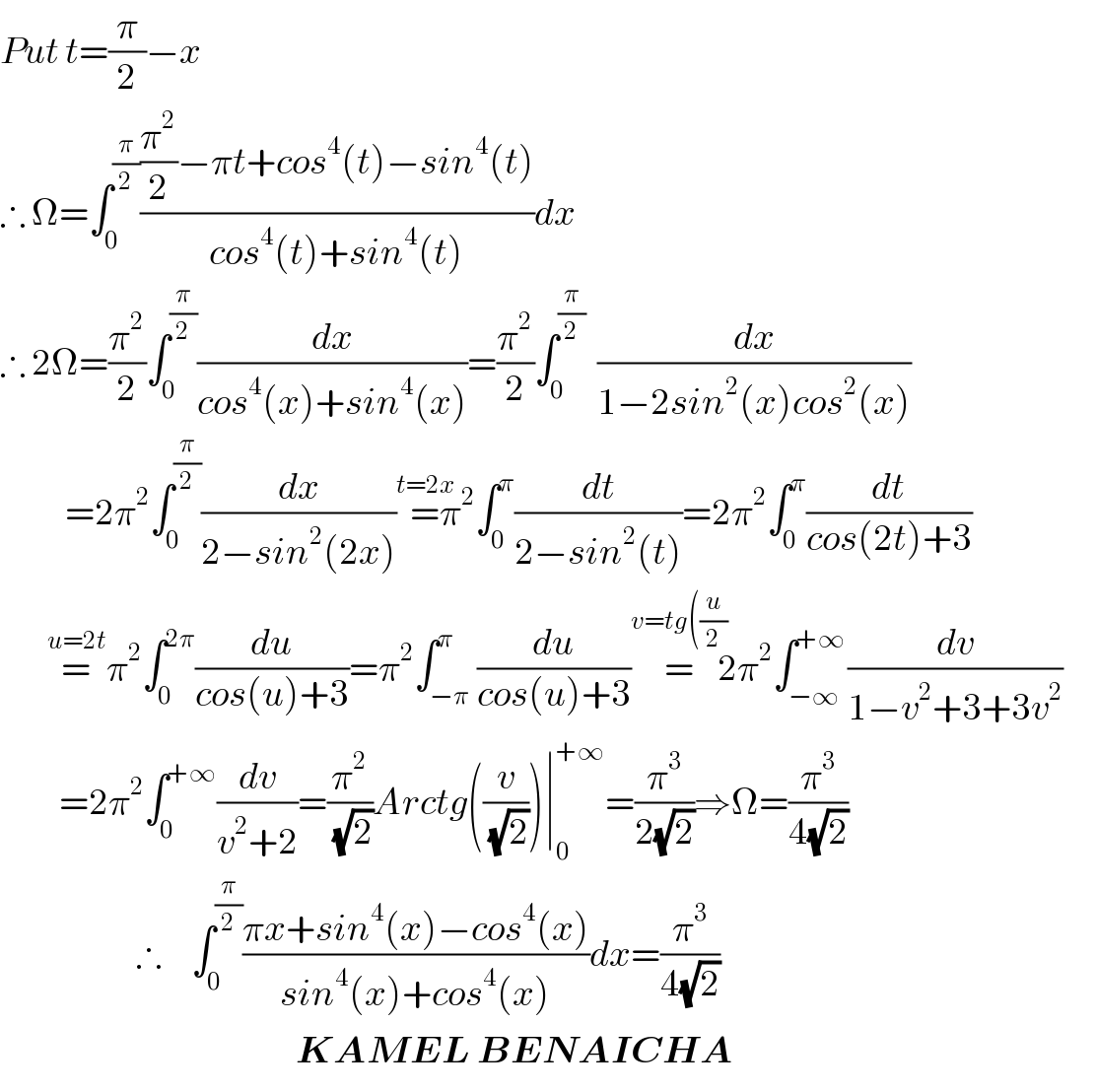
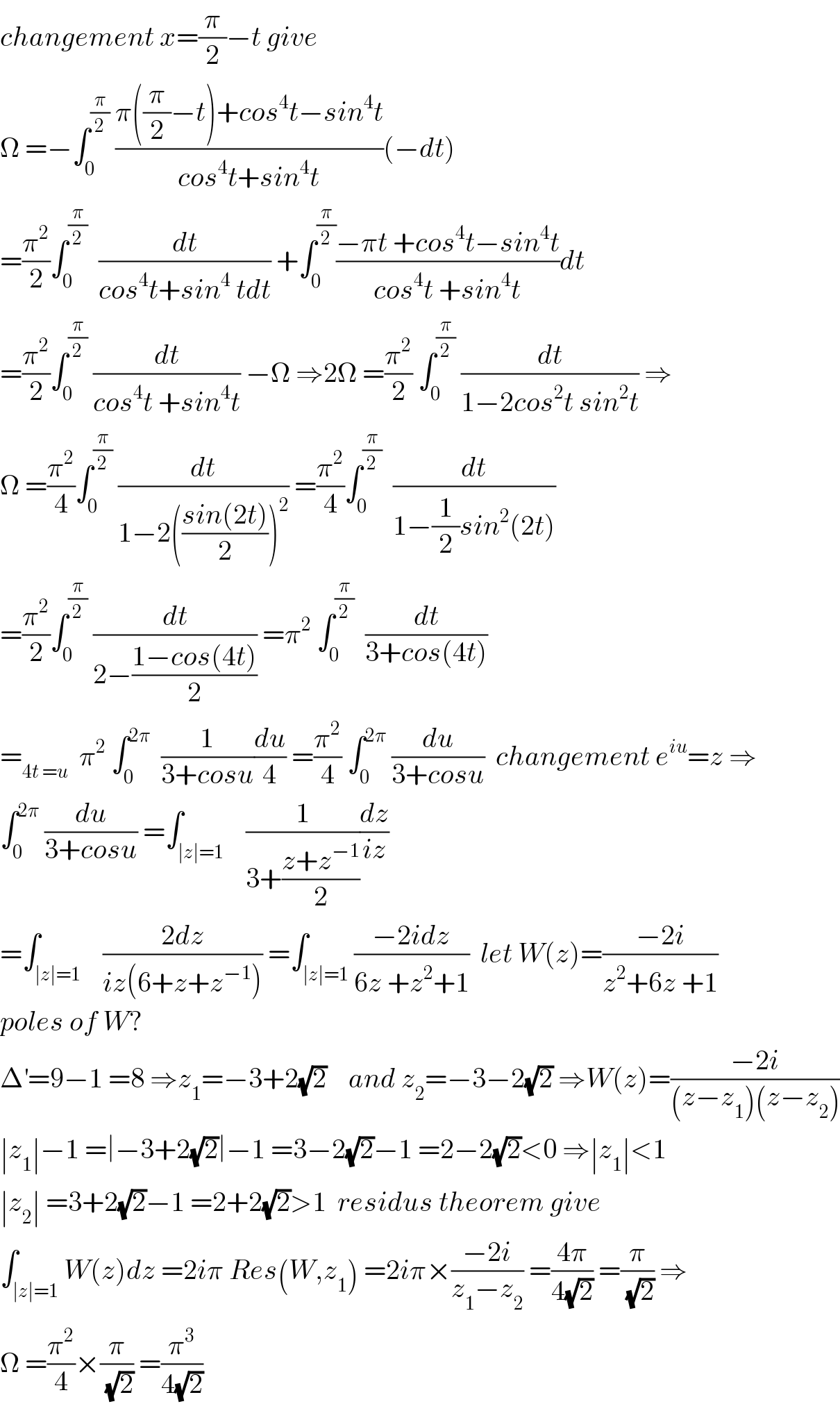
![I=∫_0 ^(π/2) ((π((π/2)−x)+sin^4 ((π/2)−x)−cos^4 ((π/2)−x))/(sin^4 ((π/2)−x)+cos^4 ((π/2)−x)))dx 2I=∫_0 ^(π/2) ((π((π/2)))/(cos^4 x+sin^4 x)) 2I=(π^2 /2)∫_0 ^(π/2) ((sec^2 x×(1+tan^2 x))/(1+tan^4 x))dx I=(π^2 /4)∫_0 ^∞ ((1+t^2 )/(1+t^4 ))dt [t=tanx] I=(π^2 /4)∫_0 ^∞ ((1+(1/t^2 ))/(t^2 +(1/t^2 )))dt =(π^2 /4)∫_0 ^∞ ((d(t−(1/t)))/((t−(1/t))^2 +2)) (π^2 /4)×(1/(√2))∣tan^(−1) (((t−(1/t))/(√2)))∣_0 ^∞ (π^2 /(4(√2)))×{(tan^(−1) ∞−tan^(−1) (−∞)} (π^2 /(4(√2)))×((π/2)+(π/2))=(π^3 /(4(√2))) pls check](Q83829.png)
Commented by mathmax by abdo last updated on 06/Mar/20

Commented by Power last updated on 06/Mar/20

Commented by TANMAY PANACEA last updated on 06/Mar/20

Answered by Kamel Kamel last updated on 07/Mar/20