
Question and Answers Forum
Question Number 91303 by 174 last updated on 29/Apr/20

Commented by mathmax by abdo last updated on 29/Apr/20
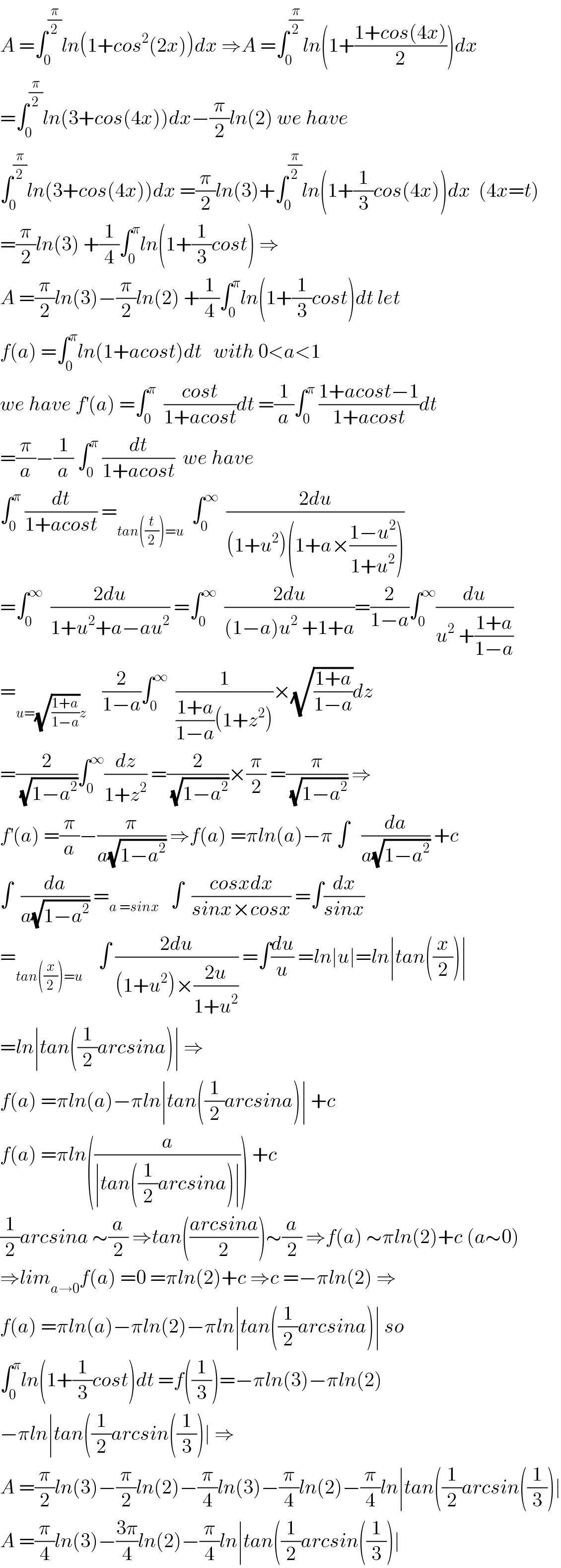
Commented by 174 last updated on 30/Apr/20
thanks alot
Commented by mathmax by abdo last updated on 30/Apr/20

| ||
Question and Answers Forum | ||
Question Number 91303 by 174 last updated on 29/Apr/20 | ||
 | ||
Commented by mathmax by abdo last updated on 29/Apr/20 | ||
 | ||
Commented by 174 last updated on 30/Apr/20 | ||
thanks alot | ||
Commented by mathmax by abdo last updated on 30/Apr/20 | ||
 | ||