
Question and Answers Forum
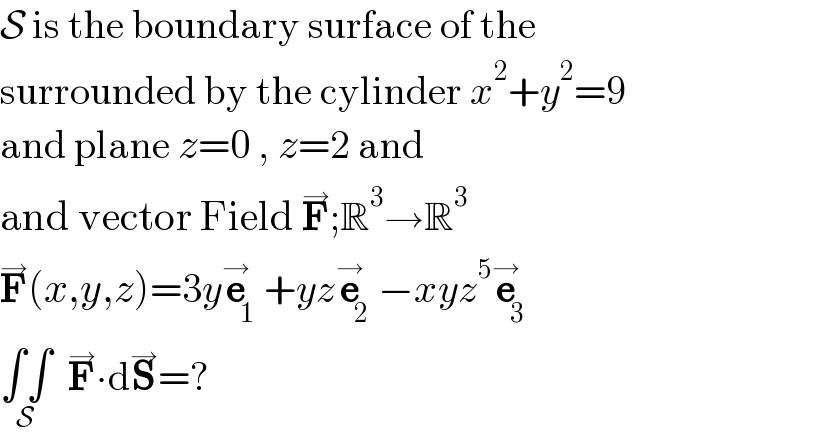
Question Number 216350 by issac last updated on 05/Feb/25
Answered by MrGaster last updated on 06/Feb/25
![∫∫_S F^→ ∙dS^→ =∫∫∫_(V ) ▽∙F^→ dV ▽∙F^→ =(∂/∂x)(3y)+(∂/∂y)(yz)+(∂/∂z)(−xyz^5 )=0+z−5xyz^4 ∫∫∫_V (z−5xyz^4 )dV=∫_0 ^2 ∫_0 ^(2π) ∫_0 ^3 (r cos θ−5r^2 cosθz^4 )r dr dθ dz =∫_0 ^2 ∫_0 ^(2π) [(r^3 /3)cos θ−((5r^4 )/4)cos θz^4 ]_0 ^3 dθ dz =∫_0 ^2 ∫_0 ^(2π) (9 cos θ−((405)/4)cosθz^4 )dθ dz =∫_0 ^2 [9 sinθ−((405)/4)sin θz^4 ]_0 ^(2π) dz=0 ∫∫_S F^→ ∙dS^→ =0](Q216376.png)
| ||
Question and Answers Forum | ||
Question Number 216350 by issac last updated on 05/Feb/25 | ||
 | ||
Answered by MrGaster last updated on 06/Feb/25 | ||
![∫∫_S F^→ ∙dS^→ =∫∫∫_(V ) ▽∙F^→ dV ▽∙F^→ =(∂/∂x)(3y)+(∂/∂y)(yz)+(∂/∂z)(−xyz^5 )=0+z−5xyz^4 ∫∫∫_V (z−5xyz^4 )dV=∫_0 ^2 ∫_0 ^(2π) ∫_0 ^3 (r cos θ−5r^2 cosθz^4 )r dr dθ dz =∫_0 ^2 ∫_0 ^(2π) [(r^3 /3)cos θ−((5r^4 )/4)cos θz^4 ]_0 ^3 dθ dz =∫_0 ^2 ∫_0 ^(2π) (9 cos θ−((405)/4)cosθz^4 )dθ dz =∫_0 ^2 [9 sinθ−((405)/4)sin θz^4 ]_0 ^(2π) dz=0 ∫∫_S F^→ ∙dS^→ =0](Q216376.png) | ||
| ||