
Question and Answers Forum
Question Number 49765 by hassentimol last updated on 10/Dec/18
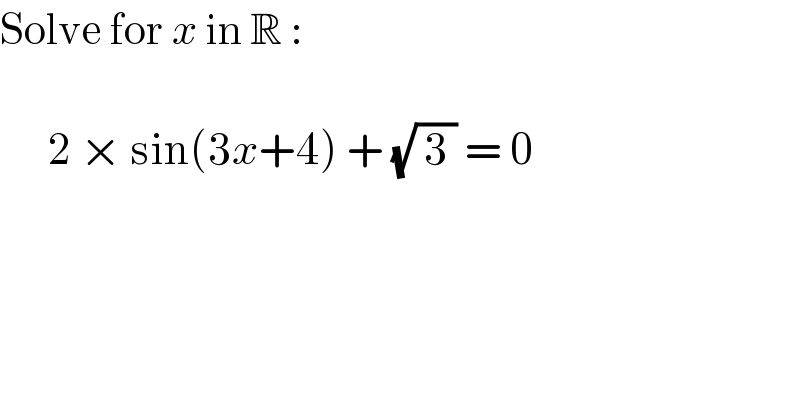
Commented by mathmax by abdo last updated on 27/Feb/20
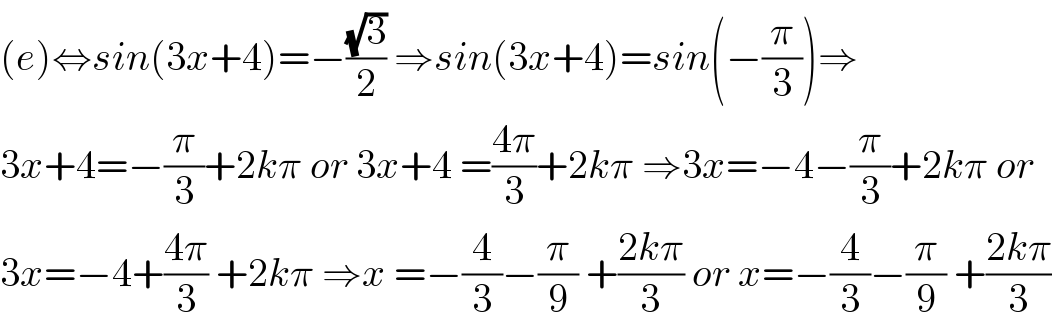
Answered by afachri last updated on 10/Dec/18
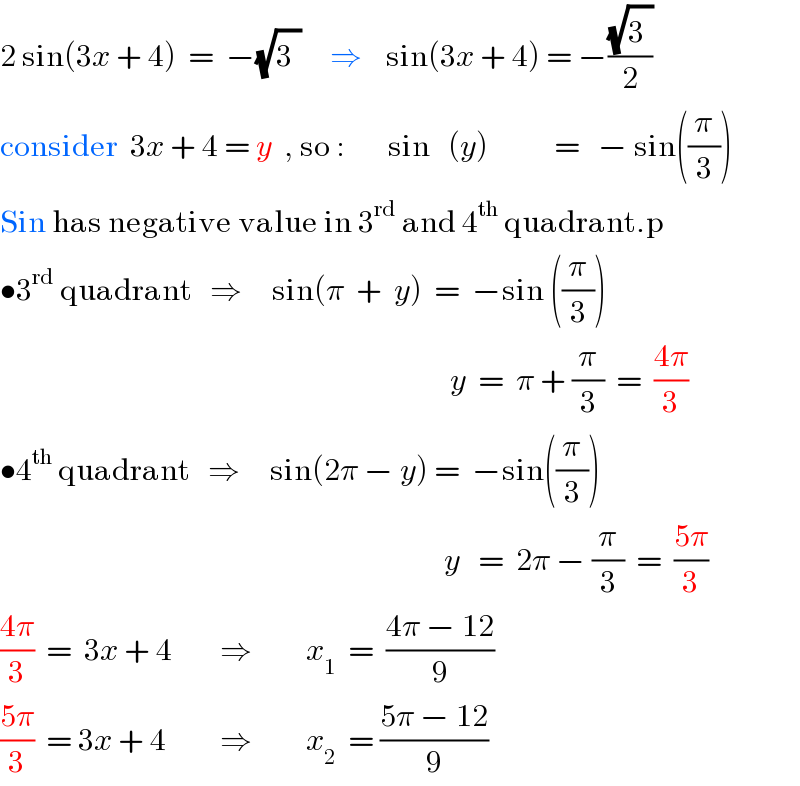
Answered by mr W last updated on 10/Dec/18
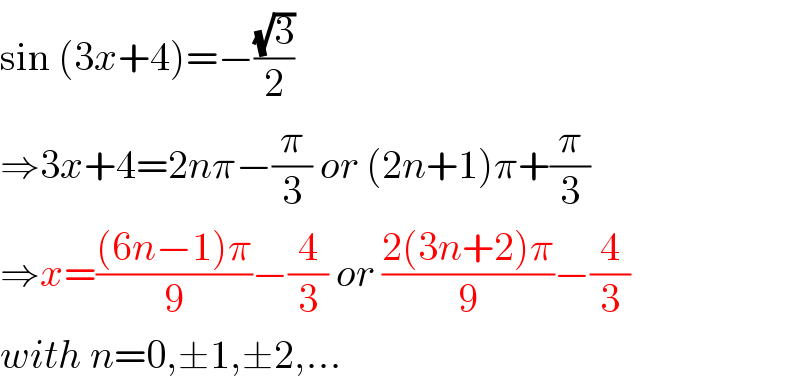
Commented by afachri last updated on 10/Dec/18

Commented by mr W last updated on 10/Dec/18
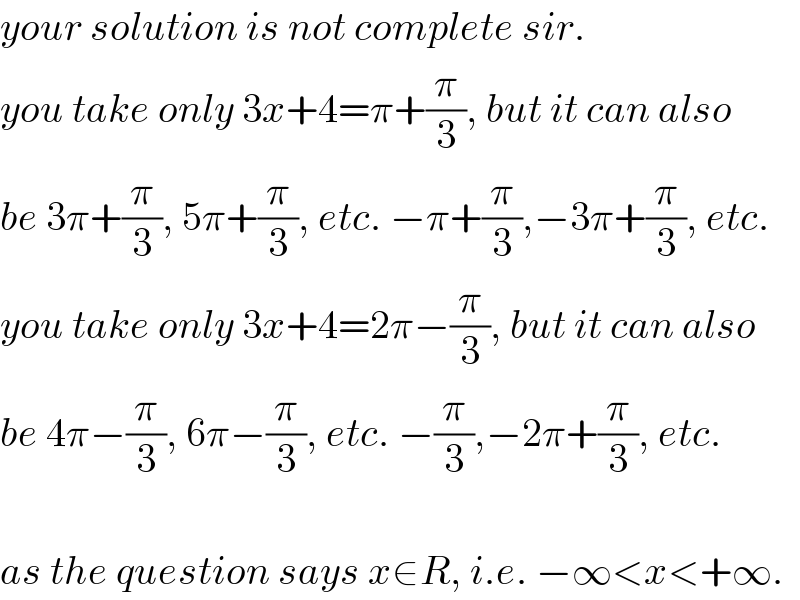
Commented by hassentimol last updated on 10/Dec/18
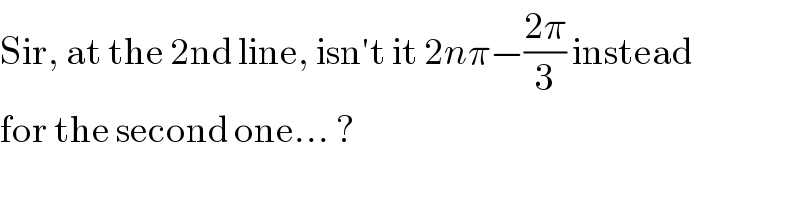
Commented by afachri last updated on 10/Dec/18

Commented by mr W last updated on 10/Dec/18
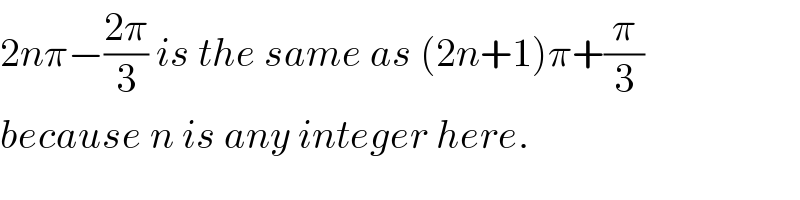
Commented by hassentimol last updated on 10/Dec/18

