
Question and Answers Forum
VectorQuestion and Answers: Page 10







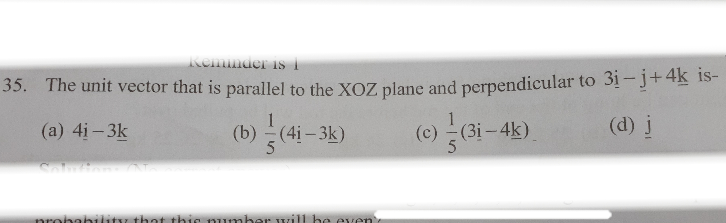




|
Question and Answers Forum |
VectorQuestion and Answers: Page 10 |

|

|
| find the gradient of scalar point function being expressed in term of scalar triple product as u=(a^ ,b^ ,c^ )=a^ .b^ ×c^ |

|

|
| In a parallelogram OABC, OA^⇁ =a^(−⇁) , OC^→ =c^→ , D is a point such that AD^→ :DB^→ =1:2 Express the following in terms of a and c (i)CB^→ (ii)BC^→ (iii)AB^→ (iv) AD^→ (v)OD^→ (vi)DC^→ |

|

|

|
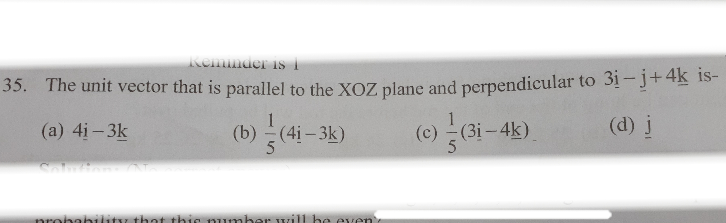
|

|

|
| ∫(((x^(−3) +2x−4)/x)) |
| ∫(dx/(x(√(x^2 +x+1 ))))=? please help |
| the vectors a and b are such that ∣a∣ =3 , ∣b∣=5 and a.b=−14 find ∣a−b∣ |
| please just read equation of a line and a plane in vectors. i don′t understand (r−a)×b=0 ?? |
| (1) A plane contains the lines ((x+1)/2)=((4−y)/2)=((z−2)/3) and r= (2i+2j + 12k)+t(−i+2j +4k). find (a) the angle between these lines. (b) A cartesian equation of the plane. (2) Given the lines l_1 :((x−10)/3)=((y−1)/1)=((z−9)/4) l_2 :r=(−9j+13k)+μ(i+2j−3k) where μ is a parameter; l_3 :((x+10)/4)=((y+5)/3)=((z+4)/1). a) show that the point (4,−1,1) is common to l_1 and l_2 . Find b) the point of intersection of l_2 and l_3 . c) A vector parametric equation of the plane containing the lines l_2 and l_3 . sir Forkum Michael |

|

|
| find the resultant force of a system of three forces O^− P^→ =9N,O^− R^→ =10N and O^− Q^→ 10N acting at point O where angle POR is 135°,angle POQ is 135° and QOR is 90° |
| find the vector sum of two vectors of magnitude of 7 and 8 making an angle of 120° to each other |
| three forces having equal magnitude s of 10N,20N and 30N make angles of 30°,120° and 210° respectively with the positive direction of the x axis. By scale drawing find the magnitude and the direction of the resultant force |
| (√(α^2 −β^2 )) simplify |
| calculate ∫_0 ^1 (x^3 /(2e^(−x) −x^2 +2x−2)) dx |
| If D,E and F are midpoints of the sides BC,CA and AB respectively of the △ABC and O be any point.Prove that OA^→ + OB^→ +OC^→ =OD^→ +OE^→ +OF^→ |
| Who know dynamics about? |