
Question and Answers Forum
VectorQuestion and Answers: Page 7
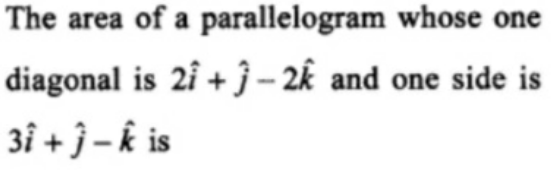


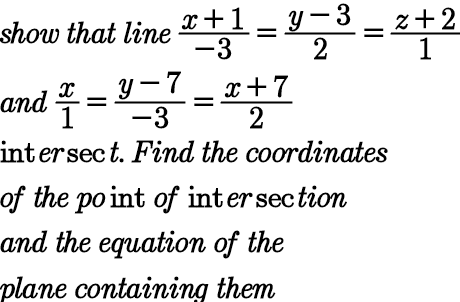
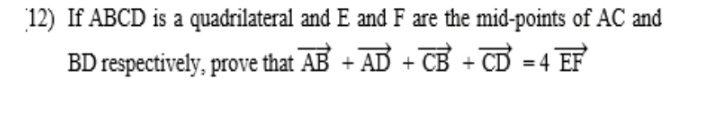

|
Question and Answers Forum |
VectorQuestion and Answers: Page 7 |
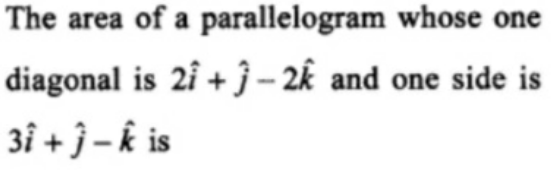
|
| If vector a^→ +b^→ +c^→ =0 ∣a^→ ∣=7, ∣b^→ ∣=3 and ∣c^→ ∣=5 find the angle vector a^→ and c^→ ? |
| ∫sin^(3/2) (x)dx |
| A vector of magnitude 2 along a bisector of the angle between the two vectors 2i^ −2j^ +k^ and i^ +2j^ −2k^ is __ |
| .... nice mathematics.... show that :: Φ = ∫_0 ^( 1) li_2 (x)dx = (π^2 /6) −1 ✓ m.n.july.1970 |
| ...nice math... evaluate :: I =∫_0 ^( (π/4)) ((ln(1+sin(x)))/(cos(x)))dx=??? ...m.n.july.1970... |
| Using displacement vector (((−4)),((−2)) ), what is the image of ((6),(3) ) when translated? |
| convert the plane with cartesian equation: x−3y + 2z = 7 into its vector parametric form. |
| The vectors p,q and r are mutially perpendicularwith ∣q∣=3 and ∣r∣=(√(5.4 )) .If X= 7p+5q+7r and Y=2p+3q−5r are perpendicular, find∣p∣. |
| ⋮^(bobhans) ∫ (dx/( (√(x(√x) −x^2 )))) = ? |

|
| ∫ ((sin x)/(5−sin 2x)) dx ? |
| Find the image of point OP^(→) = p^ in the line r^ =a^ +λb^ . |
| ∫_(π/4) ^(π/2) ln(ln(tan x)) dx |

|
| ∫_0 ^(102) (x−1)(x−2).....(x−100)×((1/(x−1))+(1/(x−2))+...+(1/(x−100)))dx |
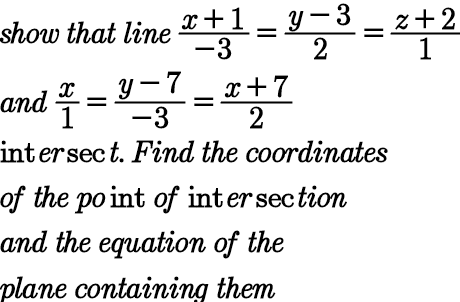
|
| Find the curvature vector and its magnitude at any point r^→ = (θ) of the curve r^→ = (acos θ,asin θ,aθ) .Show the locus of the feet of the ⊥ from the origin to the tangent is a curve that completely lies on the hyperbolic x^2 +y^2 −z^2 = a^2 |
| the vector equations of two lines l_1 and l_2 are given by l_1 :r=5i−j+k+λ(−3i+2j) l_2 :r=2i+3j+2k+μ(2j+k) find thd position vdctor of intersection of thf linds l_1 and l_2 thd cartesian equatkon of the plane π, containing the lines l_(1 ) and l_2 the sine of the anhle between the plane π and the line, l_3 :r=i−5j−2k+s(2i+2j−k) |
| Find the shortest distance from the point P(2,−3,5) to the line L ((x+3)/2)=((y−1)/(−3))=((z−2)/4) |
| determine the value of k such that the point A(4,−2,6) B(0,1,0) C(1,0,−5) and D(1,k,−2) lie on the same plane |
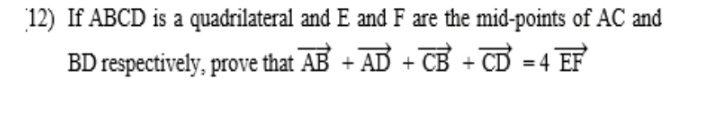
|

|
| find k if the vector (1^ −2,k) in R^3 be a linear combination of the vectors (3,0,2) &(2,−1,−5) |
| find a reduction formulae for I_n = ∫_(−1) ^0 x^n (1 + x)^2 dx |
| calculate∫_0 ^((Π/2) ) (dx/(2+cos2x)) |