
Question and Answers Forum
Vector CalculusQuestion and Answers: Page 4

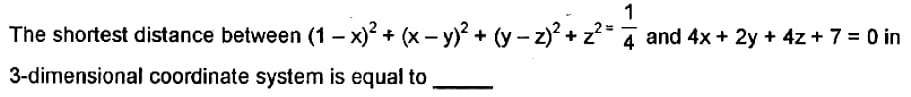
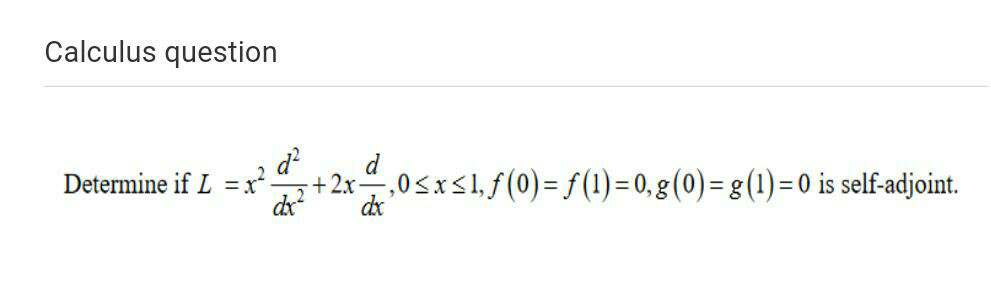





|
Question and Answers Forum |
Vector CalculusQuestion and Answers: Page 4 |
| Evaluate ∫∫_s F^→ .n^ dS where F^→ =4xi^ −2y^2 j^ +z^2 k^ and S is the surface of the cylinder bounded by x^2 +y^2 =4 ,z = 0 and z=3 . |

|
| Find the shortest distance between the skew lines ((x−3)/3) = ((8−y)/1) = ((z−3)/1) and ((x+3)/(−3)) = ((y+7)/2) = ((z−6)/4) . |
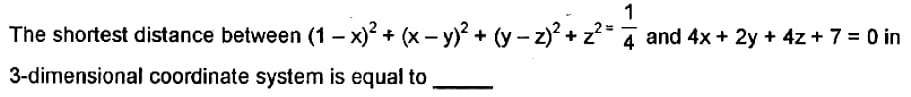
|

|
| find the angle of plane 2x−y+2z=1 and x+3y−2z = 2 |
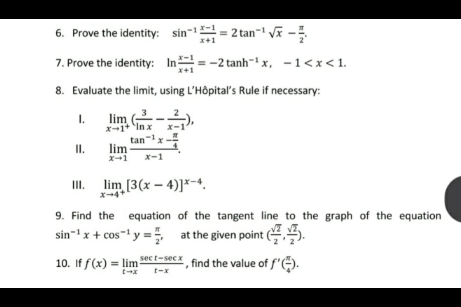
| fond∫((2x)/(1+x^2 ))dx |
| ∫(dx/(1+x^2 )) |
| let c is a constant vector and r^→ =xi^ +yj^ +zk^ then proved that grad ∣c×r^→ ∣^n =n∣c×r^→ ∣^(n−2) c×(r^→ ×c). |
| find grad r^m where r=x^2 +y^2 +z^2 |
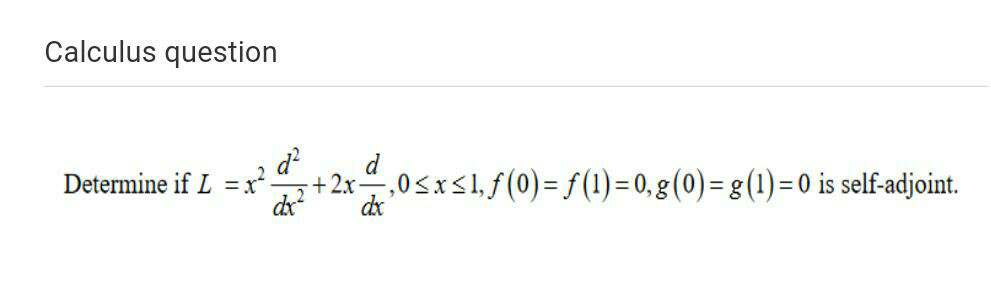
|
| what is the line passing through (2,2,1) and parallel to 2i^ − j^ − k^ ? |
| given vektor a=(3,x,−2) b=(−6,−2,y) . what the value x and y if a and b are parallel? |
| the vector equations of two lines L_1 and L_2 is given by L_1 :r= i−j+3k + λ(i−j +k) L_2 : r= 2i+aj + 6k + μ(2i + j + 3k) where a,λ,μ are real constants. given that L_1 and L_2 intersect find a. the value of the constant a. b. the position vector of the point of intersection between L_1 and L_2 c. the cosine of the acute angle between L_1 and L_2 please help |
| A particle P is projected from a point O at the edge of a cliff 60m from the sea with a velocity of 30ms^(−1) . When P is at a point B where OB is a horizontal, another particle Qsuch that P and Q hit the sea simultaneously at thesame point A. Gven that they strike the sea 6seconds after P was fired ^ calculate a) the sine of the angle of elevation of projection. b) the distance from A to O. c) the time of flight of Q. d) the Range . (take g = 10ms^(−2) ) please help |
| Prove that The necessary and sufficient condition that the curve be plane (curve) is [r′,r′′,r′′′]=0. OR A curve is plane curve iff τ=0. |
| Show that the graph of r = (sin t)i + (2cos t)j + ((√3)sin t)k is a circle |
| find the constant a,b and c so that the direction derivative of Φ=axy^2 +byz+cz^2 x^3 at (1,2,−1) has a maximum of magnitude 64 jn a direction parallel to the z axis. |
| solve4−xy+yz−xz |

|
| lim_(x→0) ((x^x −1)/(xlnx)) |

|
| Calculate, using cartesian coodinates, the following integrals: 1) ∫∫_D dxdy being D={ (x,y)∈R^2 /0≤x≤(1/2),y+x≤1,y≥0} 2) ∫∫_D x^3 ydxdy being D={(x,y)∈R^2 /0≤x≤(1/2),y+x≤1,y≥0} 3) ∫∫_D (x/y)dxdy being D={(x,y)∈R^2 /xy≤16,x≥y,x−6≤y,x≥0,y≥1} Help please! |

|

|

|