
Question and Answers Forum
Question Number 68595 by Abdo msup. last updated on 14/Sep/19
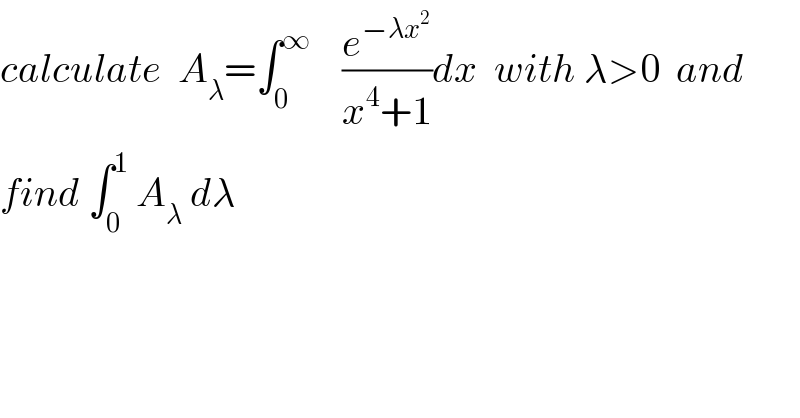
Commented by mathmax by abdo last updated on 14/Sep/19
![A_λ = ∫_0 ^∞ (e^(−λx^2 ) /(x^4 +1))dx ⇒2A_λ =∫_(−∞) ^(+∞) (e^(−λx^2 ) /(x^4 +1))dx let W(z) =(e^(−λz^2 ) /(z^4 +1)) poles of W? we have W(z) =(e^(−λx^2 ) /((z^2 −i)(z^2 +i))) =(e^(−λx^2 ) /((z−(√i))(z+(√i))(z−(√(−i)))(2+(√(−i))))) =(e^(−λx^2 ) /((z−e^((iπ)/4) )(z+e^((iπ)/4) )(z−e^(−((iπ)/4)) )(z+e^(−((iπ)/4)) ))) so the poles of W are +^− e^((iπ)/4) and +^− e^(−((iπ)/4)) residus tbeorem give ∫_(−∞) ^(+∞) W(z)dz =2iπ{Res(W,e^((iπ)/4) )+Res(W,−e^(−((iπ)/4)) )} Res(W,e^((iπ)/4) ) =(e^(−λ(i)) /(2e^((iπ)/4) (2i))) =(1/(4i)) e^(−λi−((iπ)/4)) =(1/(4i))e^(−(λ+(π/4))i) Res(W,−e^(−((iπ)/4)) ) = (e^(−λ(−i)) /((−2i)(−2e^(−((iπ)/4)) ))) =(1/(4i)) e^(λi+((iπ)/4)) =(1/(4i)) e^((λ+(π/4))i) ⇒ ∫_(−∞) ^(+∞) W(z)dz =2iπ{ (1/(4i))e^(−(λ+(π/4))i) +(1/(4i)) e^((λ+(π/4))i) } =(π/2){ 2Re(e^((λ+(π/4))i) ) =π cos((π/4) +λ) ⇒A_λ =(π/2)cos((π/4) +λ) ∫_0 ^1 A_λ dλ =(π/2) ∫_0 ^1 cos(λ+(π/4)) =[sin(λ+(π/4))]_0 ^1 =sin(1+(π/4))−((√2)/2)](Q68687.png)
| ||
Question and Answers Forum | ||
Question Number 68595 by Abdo msup. last updated on 14/Sep/19 | ||
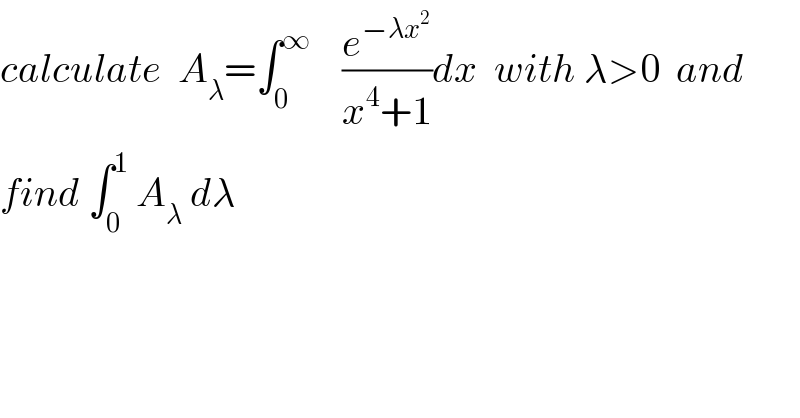 | ||
Commented by mathmax by abdo last updated on 14/Sep/19 | ||
![A_λ = ∫_0 ^∞ (e^(−λx^2 ) /(x^4 +1))dx ⇒2A_λ =∫_(−∞) ^(+∞) (e^(−λx^2 ) /(x^4 +1))dx let W(z) =(e^(−λz^2 ) /(z^4 +1)) poles of W? we have W(z) =(e^(−λx^2 ) /((z^2 −i)(z^2 +i))) =(e^(−λx^2 ) /((z−(√i))(z+(√i))(z−(√(−i)))(2+(√(−i))))) =(e^(−λx^2 ) /((z−e^((iπ)/4) )(z+e^((iπ)/4) )(z−e^(−((iπ)/4)) )(z+e^(−((iπ)/4)) ))) so the poles of W are +^− e^((iπ)/4) and +^− e^(−((iπ)/4)) residus tbeorem give ∫_(−∞) ^(+∞) W(z)dz =2iπ{Res(W,e^((iπ)/4) )+Res(W,−e^(−((iπ)/4)) )} Res(W,e^((iπ)/4) ) =(e^(−λ(i)) /(2e^((iπ)/4) (2i))) =(1/(4i)) e^(−λi−((iπ)/4)) =(1/(4i))e^(−(λ+(π/4))i) Res(W,−e^(−((iπ)/4)) ) = (e^(−λ(−i)) /((−2i)(−2e^(−((iπ)/4)) ))) =(1/(4i)) e^(λi+((iπ)/4)) =(1/(4i)) e^((λ+(π/4))i) ⇒ ∫_(−∞) ^(+∞) W(z)dz =2iπ{ (1/(4i))e^(−(λ+(π/4))i) +(1/(4i)) e^((λ+(π/4))i) } =(π/2){ 2Re(e^((λ+(π/4))i) ) =π cos((π/4) +λ) ⇒A_λ =(π/2)cos((π/4) +λ) ∫_0 ^1 A_λ dλ =(π/2) ∫_0 ^1 cos(λ+(π/4)) =[sin(λ+(π/4))]_0 ^1 =sin(1+(π/4))−((√2)/2)](Q68687.png) | ||