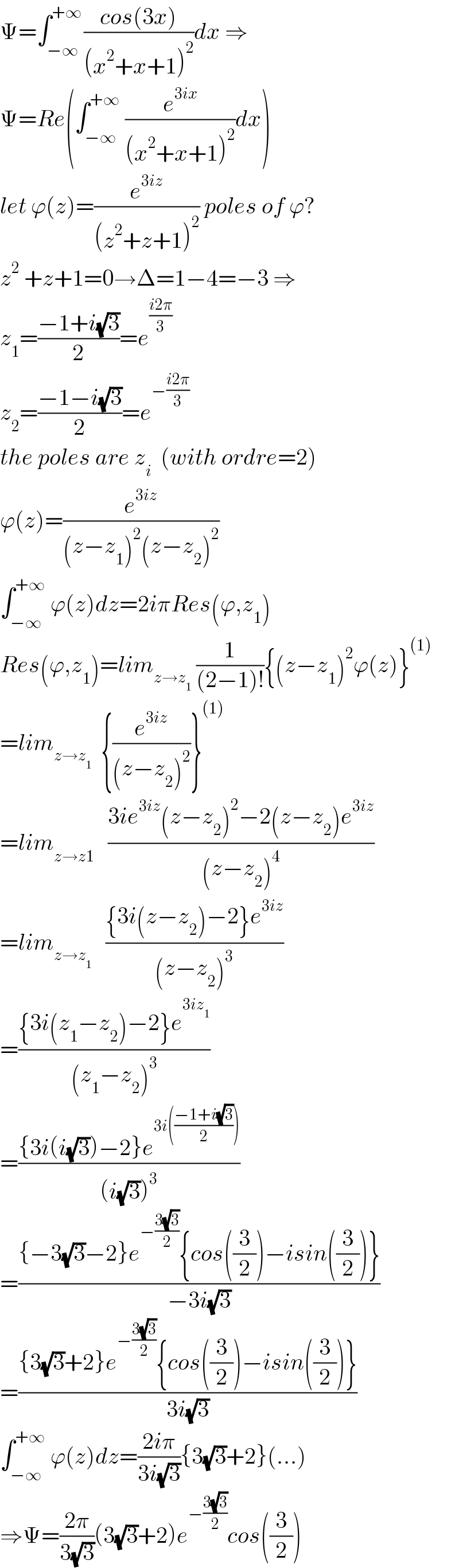calculate ∫_(−∞) ^(+∞) ((cos(3x))/((x^2 +x+1)^2 ))dx


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 162016 by mathmax by abdo last updated on 25/Dec/21

$calculate \int_{- \infty}^{+ \infty} \frac{\cos (3 x)}{{(x^{2} + x + 1)}^{2}} dx$
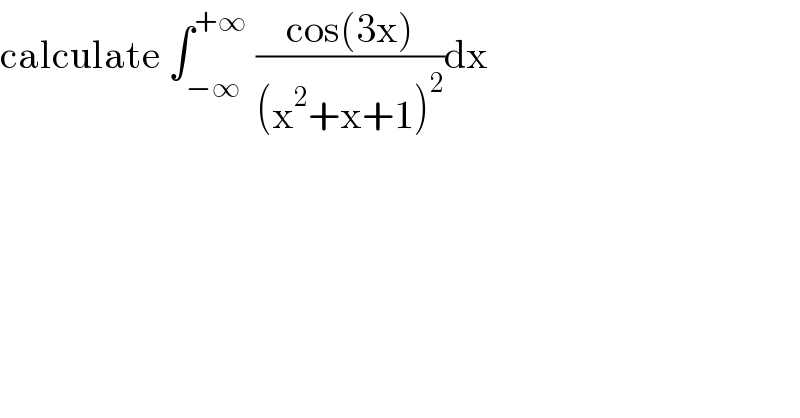
Commented by MJS_new last updated on 25/Dec/21

$I can solve the indefinite integral but {it}^{'} s a$ $long hard way . . .$
Commented by mathmax by abdo last updated on 25/Dec/21

$use residus theorem sir$
	Answered by Ar Brandon last updated on 24/Mar/22
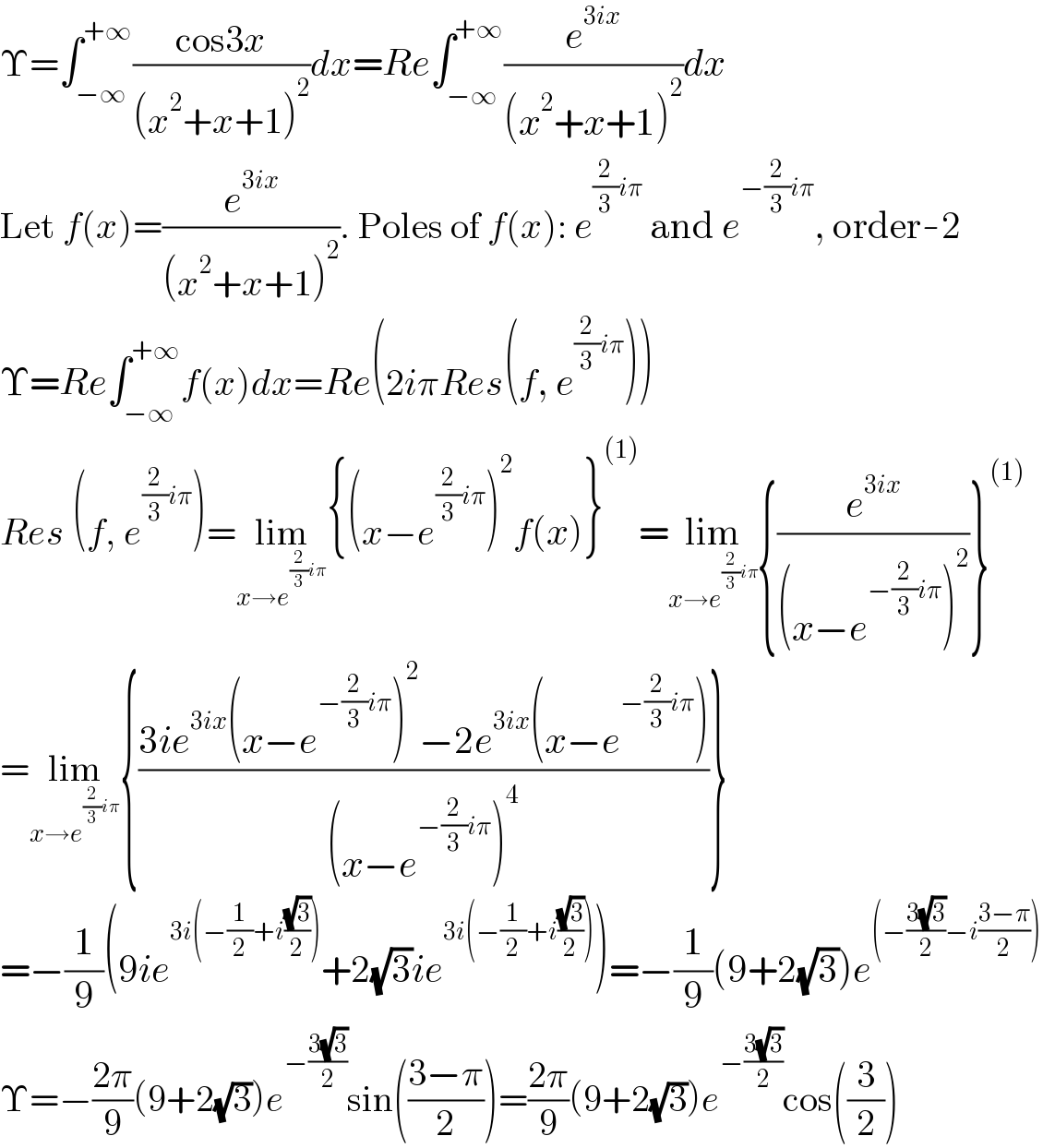
	$Υ=∫_(−∞) ^(+∞) ((cos3x)/((x^2 +x+1)^2 ))dx=Re∫_(−∞) ^(+∞) (e^(3ix) /((x^2 +x+1)^2 ))dx Let f(x)=(e^(3ix) /((x^2 +x+1)^2 )). Poles of f(x): e^((2/3)iπ) and e^(−(2/3)iπ) , order-2 Υ=Re∫_(−∞) ^(+∞) f(x)dx=Re(2iπRes(f, e^((2/3)iπ) )) Res (f, e^((2/3)iπ) )=lim_(x→e^((2/3)iπ) ) {(x−e^((2/3)iπ) )^2 f(x)}^((1)) =lim_(x→e^((2/3)iπ) ) {(e^(3ix) /((x−e^(−(2/3)iπ) )^2 ))}^((1)) =lim_(x→e^((2/3)iπ) ) {((3ie^(3ix) (x−e^(−(2/3)iπ) )^2 −2e^(3ix) (x−e^(−(2/3)iπ) ))/((x−e^(−(2/3)iπ) )^4 ))} =−(1/9)(9ie^(3i(−(1/2)+i((√3)/2))) +2(√3)ie^(3i(−(1/2)+i((√3)/2))) )=−(1/9)(9+2(√3))e^((−((3(√3))/2)−i((3−π)/2))) Υ=−((2π)/9)(9+2(√3))e^(−((3(√3))/2)) sin(((3−π)/2))=((2π)/9)(9+2(√3))e^(−((3(√3))/2)) cos((3/2))$
	$Υ = \int_{- \infty}^{+ \infty} \frac{\cos 3 x}{{(x^{2} + x + 1)}^{2}} d x = R e \int_{- \infty}^{+ \infty} \frac{e^{3 i x}}{{(x^{2} + x + 1)}^{2}} d x$ $Let f (x) = \frac{e^{3 i x}}{{(x^{2} + x + 1)}^{2}} . Poles of f (x) : e^{\frac{2}{3} i π} and e^{- \frac{2}{3} i π}, order - 2$ $Υ = R e \int_{- \infty}^{+ \infty} f (x) d x = R e (2 i π R e s (f, e^{\frac{2}{3} i π}))$ $R e s (f, e^{\frac{2}{3} i π}) = \lim_{x \to e^{\frac{2}{3} i π}} {{(x - e^{\frac{2}{3} i π})}^{2} f (x)}^{(1)} = \lim_{x \to e^{\frac{2}{3} i π}} {\frac{e^{3 i x}}{{(x - e^{- \frac{2}{3} i π})}^{2}}}^{(1)}$ $= \lim_{x \to e^{\frac{2}{3} i π}} {\frac{3 {i e}^{3 i x} {(x - e^{- \frac{2}{3} i π})}^{2} - 2 e^{3 i x} (x - e^{- \frac{2}{3} i π})}{{(x - e^{- \frac{2}{3} i π})}^{4}}}$ $= - \frac{1}{9} (9 {i e}^{3 i (- \frac{1}{2} + i \frac{\sqrt{3}}{2})} + 2 \sqrt{3} {i e}^{3 i (- \frac{1}{2} + i \frac{\sqrt{3}}{2})}) = - \frac{1}{9} (9 + 2 \sqrt{3}) e^{(- \frac{3 \sqrt{3}}{2} - i \frac{3 - π}{2})}$ $Υ = - \frac{2 π}{9} (9 + 2 \sqrt{3}) e^{- \frac{3 \sqrt{3}}{2}} \sin (\frac{3 - π}{2}) = \frac{2 π}{9} (9 + 2 \sqrt{3}) e^{- \frac{3 \sqrt{3}}{2}} \cos (\frac{3}{2})$
	Commented by Mathspace last updated on 26/Dec/21

	$e r r o r o f c a l c u l u s . .$
	Commented by Ar Brandon last updated on 26/Dec/21

	$Thank you for checking, Sir . Rectified!$
	Answered by Mathspace last updated on 26/Dec/21
	$Ψ=∫_(−∞) ^(+∞ ) ((cos(3x))/((x^2 +x+1)^2 ))dx ⇒ Ψ=Re(∫_(−∞) ^(+∞) (e^(3ix) /((x^2 +x+1)^2 ))dx) let ϕ(z)=(e^(3iz) /((z^2 +z+1)^2 )) poles of ϕ? z^2 +z+1=0→Δ=1−4=−3 ⇒ z_1 =((−1+i(√3))/2)=e^((i2π)/3) z_2 =((−1−i(√3))/2)=e^(−((i2π)/3)) the poles are z_i (with ordre=2) ϕ(z)=(e^(3iz) /((z−z_1 )^2 (z−z_2 )^2 )) ∫_(−∞) ^(+∞) ϕ(z)dz=2iπRes(ϕ,z_1 ) Res(ϕ,z_1 )=lim_(z→z_1 ) (1/((2−1)!)){(z−z_1 )^2 ϕ(z)}^((1)) =lim_(z→z_1 ) {(e^(3iz) /((z−z_2 )^2 ))}^((1)) =lim_(z→z1) ((3ie^(3iz) (z−z_2 )^2 −2(z−z_2 )e^(3iz) )/((z−z_2 )^4 )) =lim_(z→z_1 ) (({3i(z−z_2 )−2}e^(3iz) )/((z−z_2 )^3 )) =(({3i(z_1 −z_2 )−2}e^(3iz_1 ) )/((z_1 −z_2 )^3 )) =(({3i(i(√3))−2}e^(3i(((−1+i(√3))/2))) )/((i(√3))^3 )) =(({−3(√3)−2}e^(−((3(√3))/2)) {cos((3/2))−isin((3/2))})/(−3i(√3))) =(({3(√3)+2}e^(−((3(√3))/2)) {cos((3/2))−isin((3/2))})/(3i(√3))) ∫_(−∞) ^(+∞) ϕ(z)dz=((2iπ)/(3i(√3))){3(√3)+2}(...) ⇒Ψ=((2π)/(3(√3)))(3(√3)+2)e^(−((3(√3))/2)) cos((3/2))$
	$Ψ = \int_{- \infty}^{+ \infty} \frac{c o s (3 x)}{{(x^{2} + x + 1)}^{2}} d x \Rightarrow$ $Ψ = R e (\int_{- \infty}^{+ \infty} \frac{e^{3 i x}}{{(x^{2} + x + 1)}^{2}} d x)$ $l e t φ (z) = \frac{e^{3 i z}}{{(z^{2} + z + 1)}^{2}} p o l e s o f φ ?$ $z^{2} + z + 1 = 0 \to Δ = 1 - 4 = - 3 \Rightarrow$ $z_{1} = \frac{- 1 + i \sqrt{3}}{2} = e^{\frac{i 2 π}{3}}$ $z_{2} = \frac{- 1 - i \sqrt{3}}{2} = e^{- \frac{i 2 π}{3}}$ $t h e p o l e s a r e z_{i} (w i t h o r d r e = 2)$ $φ (z) = \frac{e^{3 i z}}{{(z - z_{1})}^{2} {(z - z_{2})}^{2}}$ $\int_{- \infty}^{+ \infty} φ (z) d z = 2 i π R e s (φ, z_{1})$ $R e s (φ, z_{1}) = {l i m}_{z \to z_{1}} \frac{1}{(2 - 1)!} {{(z - z_{1})}^{2} φ (z)}^{(1)}$ $= {l i m}_{z \to z_{1}} {\frac{e^{3 i z}}{{(z - z_{2})}^{2}}}^{(1)}$ $= {l i m}_{z \to z 1} \frac{3 {i e}^{3 i z} {(z - z_{2})}^{2} - 2 (z - z_{2}) e^{3 i z}}{{(z - z_{2})}^{4}}$ $= {l i m}_{z \to z_{1}} \frac{{3 i (z - z_{2}) - 2} e^{3 i z}}{{(z - z_{2})}^{3}}$ $= \frac{{3 i (z_{1} - z_{2}) - 2} e^{3 {i z}_{1}}}{{(z_{1} - z_{2})}^{3}}$ $= \frac{{3 i (i \sqrt{3}) - 2} e^{3 i (\frac{- 1 + i \sqrt{3}}{2})}}{{(i \sqrt{3})}^{3}}$ $= \frac{{- 3 \sqrt{3} - 2} e^{- \frac{3 \sqrt{3}}{2}} {c o s (\frac{3}{2}) - i s i n (\frac{3}{2})}}{- 3 i \sqrt{3}}$ $= \frac{{3 \sqrt{3} + 2} e^{- \frac{3 \sqrt{3}}{2}} {c o s (\frac{3}{2}) - i s i n (\frac{3}{2})}}{3 i \sqrt{3}}$ $\int_{- \infty}^{+ \infty} φ (z) d z = \frac{2 i π}{3 i \sqrt{3}} {3 \sqrt{3} + 2} (. . .)$ $\Rightarrow Ψ = \frac{2 π}{3 \sqrt{3}} (3 \sqrt{3} + 2) e^{- \frac{3 \sqrt{3}}{2}} c o s (\frac{3}{2})$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum