Question and Answers Forum
Question Number 63519 by Rio Michael last updated on 05/Jul/19
Commented by Prithwish sen last updated on 05/Jul/19
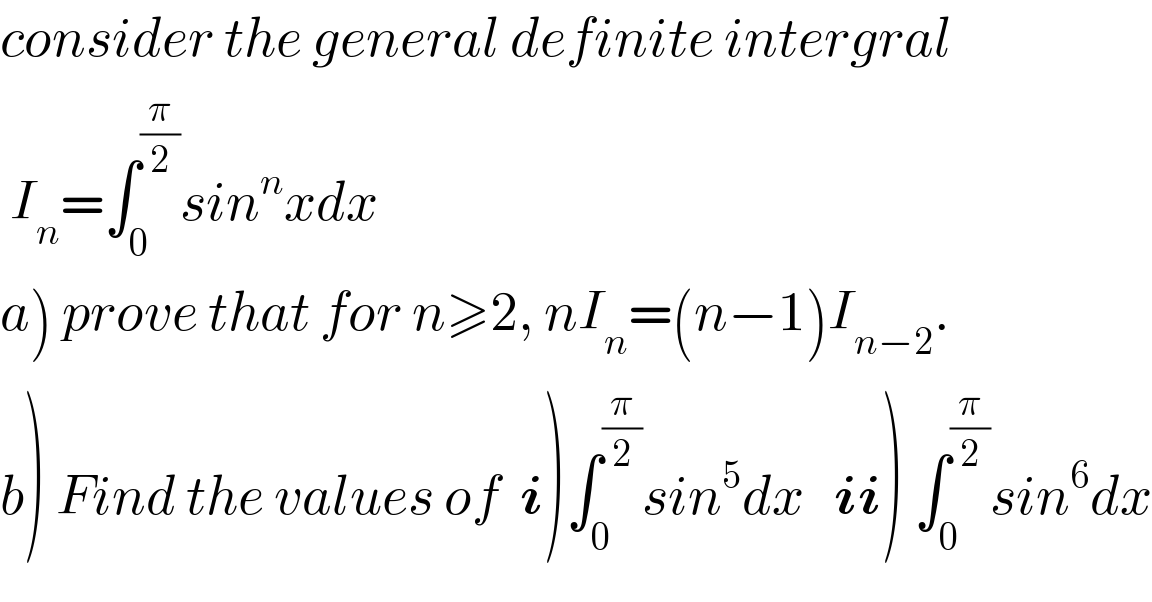
![I_n =∫_0 ^(π/2) sin^(n−1) x sinxdx, using by parts =[−sin^(n−1) xcosx]_0 ^(π/2) + (n−1)∫_0 ^(π/2) sin^(n−2) x(1−sin^2 x)^ dx = (n−1)I_(n−2) −(n−1)I_n nI_n = (n−1)I_(n−2) proved ∫_0 ^(π/2) sin^5 x dx = (((5−1))/5)I_3 = (4/5).(((3−1))/3) I_(3−2) = (4/5).(2/3).I =(8/(15 )) ∫_0 ^(π/2) sinx dx = −(8/(15)) (cosx)_0 ^(π/2) = (8/(15)) similarly you can deduce (ii)](Q63523.png)
Commented by Rio Michael last updated on 05/Jul/19
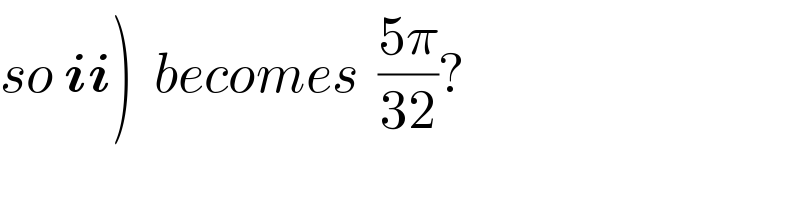
Commented by Prithwish sen last updated on 05/Jul/19

Commented by Rio Michael last updated on 05/Jul/19