
Question and Answers Forum
Question Number 66104 by Rio Michael last updated on 09/Aug/19
Commented by mathmax by abdo last updated on 09/Aug/19
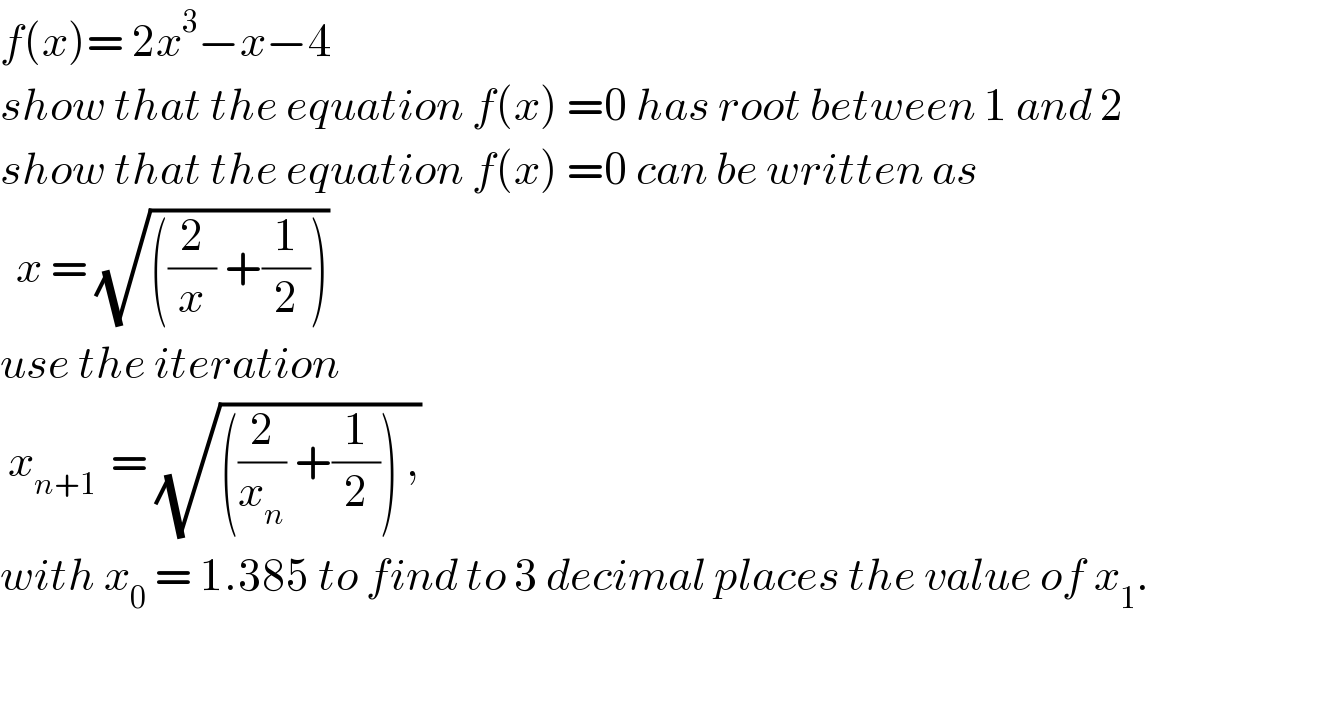
![we have f(x) =2x^3 −x−4 ⇒f^′ (x)=6x^2 −1 =((√6)x−1)((√6)x+1) f^′ (x)=0 ⇔x =+^− (1/(√6)) variation of f(x) x −∞ −(1/(√6)) (1/(√6)) +∞ f^′ (x) + − + f(x) −∞ incr. f(−(1/(√6))) decr f((1/(√6))) incr +∞ f is increasing on [(1/(√6)) ,+∞[ f(1) =2−1−4 =−5 f(2)=16−2−4 =10 ⇒f(1)f(2)<0 ⇒∃ α ∈]1,2[ /f(α)=0 x=(√((2/x)+(1/2)))and x>0 ⇒x^2 =((4+x)/(2x)) ⇒2x^3 =4+x ⇒2x^3 −x−4=0 ⇒ f(x)=0 so f(x)=0 and x>0 ⇔x=(√((2/x)+(1/2))) if we consider the iteration x_(n+1) =(√((2/x_n )+(1/2))) we get x_1 =(√((2/x_0 )+(1/2)))=(√((4+x_0 )/(2x_0 )))=(√((4+1,385)/(2×1,385))) rest to finish the calculus....](Q66167.png)
| ||
Question and Answers Forum | ||
Question Number 66104 by Rio Michael last updated on 09/Aug/19 | ||
 | ||
Commented by mathmax by abdo last updated on 09/Aug/19 | ||
![we have f(x) =2x^3 −x−4 ⇒f^′ (x)=6x^2 −1 =((√6)x−1)((√6)x+1) f^′ (x)=0 ⇔x =+^− (1/(√6)) variation of f(x) x −∞ −(1/(√6)) (1/(√6)) +∞ f^′ (x) + − + f(x) −∞ incr. f(−(1/(√6))) decr f((1/(√6))) incr +∞ f is increasing on [(1/(√6)) ,+∞[ f(1) =2−1−4 =−5 f(2)=16−2−4 =10 ⇒f(1)f(2)<0 ⇒∃ α ∈]1,2[ /f(α)=0 x=(√((2/x)+(1/2)))and x>0 ⇒x^2 =((4+x)/(2x)) ⇒2x^3 =4+x ⇒2x^3 −x−4=0 ⇒ f(x)=0 so f(x)=0 and x>0 ⇔x=(√((2/x)+(1/2))) if we consider the iteration x_(n+1) =(√((2/x_n )+(1/2))) we get x_1 =(√((2/x_0 )+(1/2)))=(√((4+x_0 )/(2x_0 )))=(√((4+1,385)/(2×1,385))) rest to finish the calculus....](Q66167.png) | ||