
Question and Answers Forum
Question Number 87799 by M±th+et£s last updated on 06/Apr/20
![f(x)= { ((ax^2 +bx −1≤x≤0)),((cx^2 +d 0<x≤(1/2))),((bx+d (1/2)<x≤1)) :} f(x) is continuous on[−1,1] prove d=0 c=2b](Q87799.png)
Commented by john santu last updated on 06/Apr/20
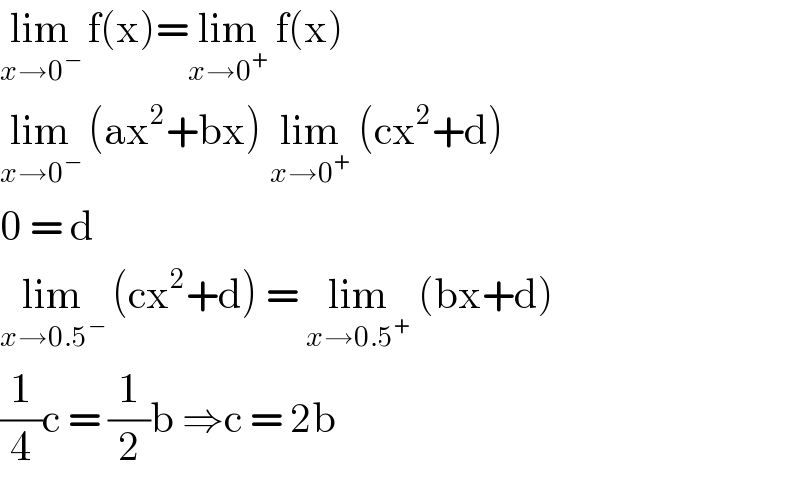
Commented by M±th+et£s last updated on 06/Apr/20

| ||
Question and Answers Forum | ||
Question Number 87799 by M±th+et£s last updated on 06/Apr/20 | ||
![f(x)= { ((ax^2 +bx −1≤x≤0)),((cx^2 +d 0<x≤(1/2))),((bx+d (1/2)<x≤1)) :} f(x) is continuous on[−1,1] prove d=0 c=2b](Q87799.png) | ||
Commented by john santu last updated on 06/Apr/20 | ||
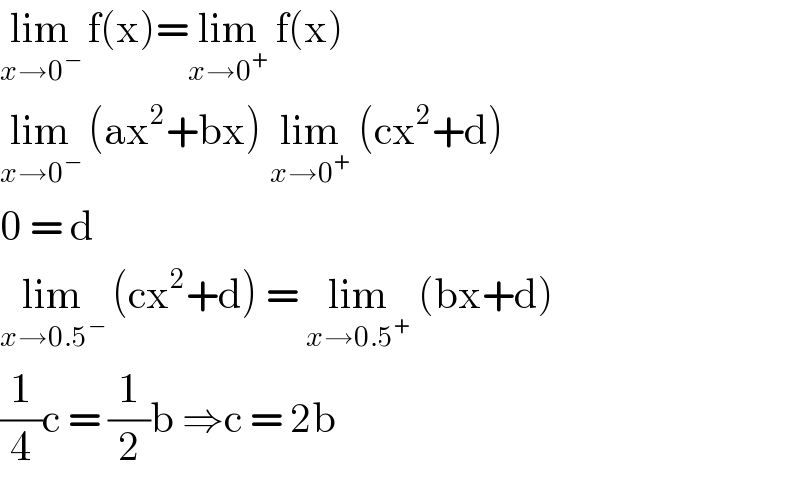 | ||
Commented by M±th+et£s last updated on 06/Apr/20 | ||
 | ||