Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 178793 by infinityaction last updated on 21/Oct/22

$$\mathrm{find}\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{1}{\left(3n\right)!}=1+\frac{1}{3!}+\frac{1}{6!}+\frac{1}{9!}+...$$

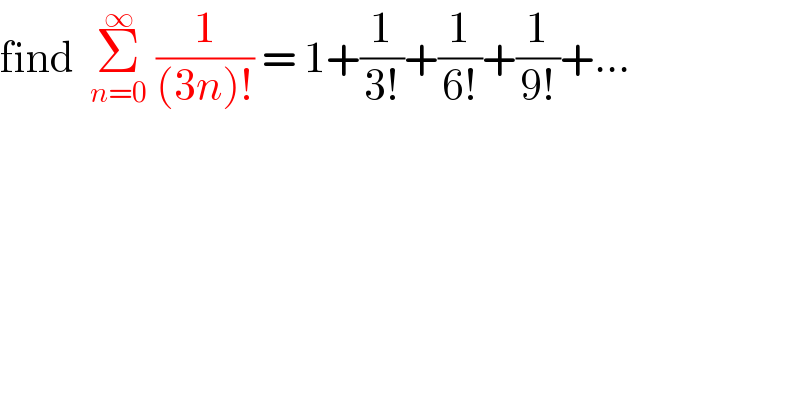
Answered by aleks041103 last updated on 21/Oct/22

$$w=e^{\frac{2\pi i}{3}}\Rightarrow w^2=w^{\ast },w^3=1$$$$e^w=\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{1}{\left(3n\right)!}+\frac{w}{(3n+1)!}+\frac{w^2}{(3n+2)!}=S_0+{wS}_1+w^2{S}_2$$$$e^{w^2}=\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{1}{\left(3n\right)!}+\frac{w^2}{(3n+1)!}+\frac{w}{(3n+2)!}=S_0+w^2{S}_1+{wS}_2$$$$e^1=S_0+S_1+S_2$$$$e^w+e^{w^2}-2e=(w+w^2-2)(S_1+S_2)=-3(S_1+S_2)$$$$\Rightarrow S_0=e-(S_1+S_2)=e+\frac{(exp\left(w\right)+exp\left(w^2\right)-2e)}{3}=$$$$=\frac{e^1+e^w+e^{w^2}}{3}=S_0$$$$w=e^{\frac{2\pi i}{3}}=-\frac{1}{2}+i\frac{\sqrt{3}}{2}$$$$w^2=e^{-\frac{2\pi i}{3}}=-\frac{1}{2}-i\frac{\sqrt{3}}{2}$$$$\Rightarrow e^w=\frac{1}{\sqrt{e}}{e}^{i\sqrt{3}/2},e^{w^2}=\frac{1}{\sqrt{e}}{e}^{-i\sqrt{3}/2}$$$$\Rightarrow e^w+e^{w^2}=\frac{2}{\sqrt{e}}cos\left(\frac{\sqrt{3}}{2}\right)$$$$\Rightarrow \underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{1}{\left(3n\right)!}=\frac{e}{3}+\frac{2}{3\sqrt{e}}cos\left(\sqrt{3}/2\right)$$

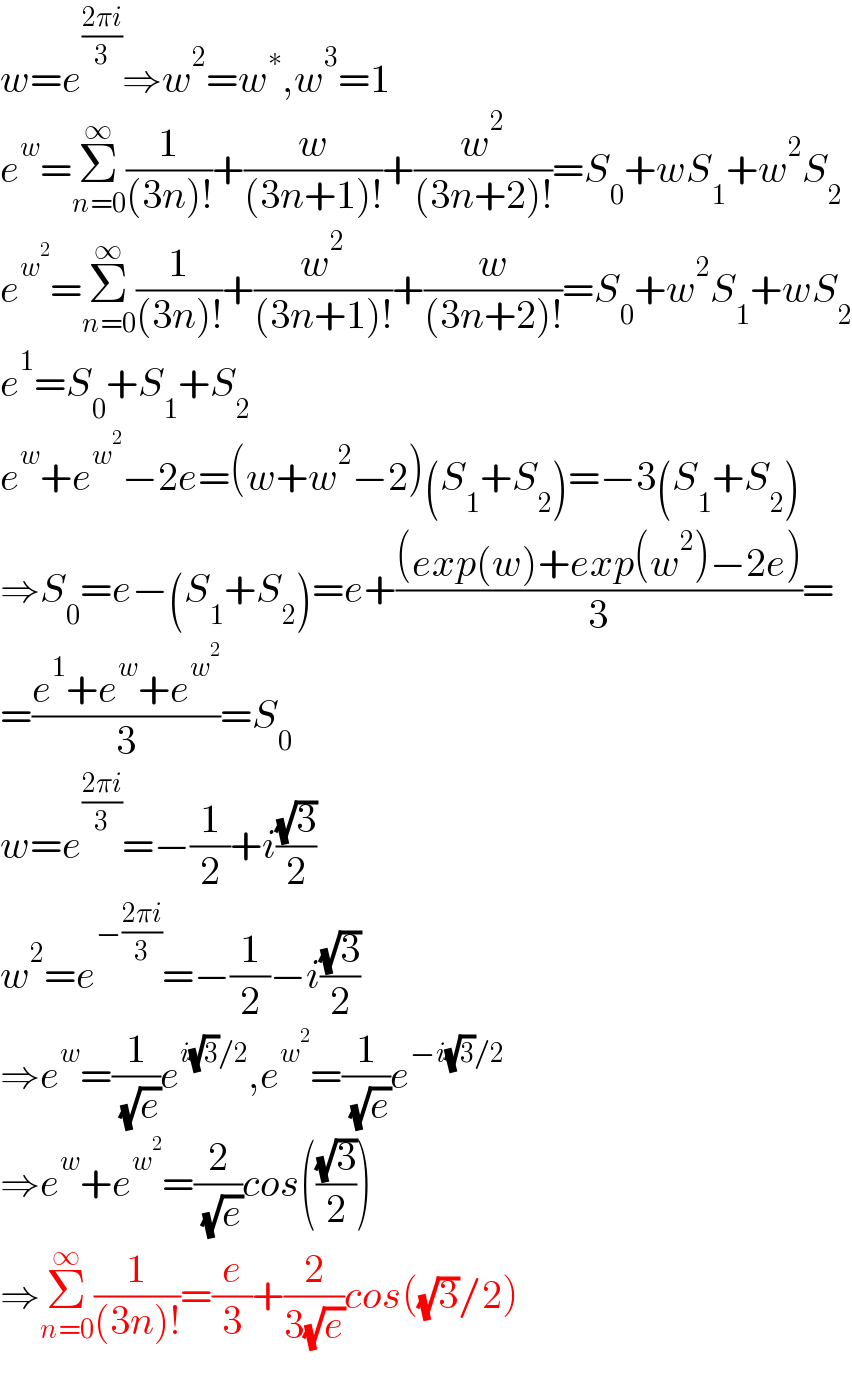
Commented by aleks041103 last updated on 21/Oct/22

Commented by Tawa11 last updated on 22/Oct/22

$$\mathrm{Great}\mathrm{sir}$$

Commented by infinityaction last updated on 22/Oct/22

$$thankssir$$

Answered by ARUNG_Brandon_MBU last updated on 22/Oct/22

$$S=\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{1}{\left(3n\right)!}=1+\frac{1}{3!}+\frac{1}{6!}+\frac{1}{9!}+\cdot \cdot \cdot$$$$=(1+\frac{1}{1!}+\frac{1}{2!}+\frac{1}{3!}+\frac{1}{4!}+\frac{1}{5!}+\frac{1}{6!}+\frac{1}{7!}+\frac{1}{8!}+\frac{1}{9!}+\cdot \cdot \cdot )-(\frac{1}{1!}+\frac{1}{2!}+\frac{1}{4!}+\frac{1}{5!}+\frac{1}{7!}+\frac{1}{8!}+\cdot \cdot \cdot )$$$$=e-(\frac{1}{1!}+\frac{1}{4!}+\frac{1}{7!}+\cdot \cdot \cdot )-(\frac{1}{2!}+\frac{1}{5!}+\frac{1}{8!}+\cdot \cdot \cdot )=e-\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{1}{(3n+1)!}-\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{1}{(3n+2)!}$$$$\Rightarrow e=\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{1}{\left(3n\right)!}+\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{1}{(3n+1)!}+\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{1}{(3n+2)!}$$$$e^x=\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{x^{3n}}{\left(3n\right)!}+\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{x^{3n+1}}{(3n+1)!}+\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{x^{3n+2}}{(3n+2)}\Rightarrow e^x=f\left(x\right)+f{}^{\prime }\left(x\right)+f{}^{″}\left(x\right)$$$$\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{x^n}{\left(3n\right)!}\mathrm{is}\mathrm{solution}\mathrm{to}\mathrm{this}\mathrm{equation}\mathrm{with}f\left(0\right)=1\mathrm{and}f{}^{\prime }\left(0\right)=0$$$$y_{gh}:r^2+r+1=0\Rightarrow r=-\frac{1}{2}\pm i\frac{\sqrt{3}}{2}\Rightarrow y_{gh}=e^{-\frac{x}{2}}(\alpha \cos \left(\frac{\sqrt{3}}{2}x\right)+\beta \sin \left(\frac{\sqrt{3}}{2}x\right))$$$$y_p={ke}^x=y_p^{\prime }=y_p^{″}\Rightarrow e^x={ke}^x+{ke}^x+{ke}^x\Rightarrow k=\frac{1}{3}$$$$f\left(x\right)=y_{gh}+y_p=e^{-\frac{x}{2}}(\alpha \cos \left(\frac{\sqrt{3}}{2}x\right)+\beta \sin \left(\frac{\sqrt{3}}{2}x\right))+\frac{e^x}{3},f\left(0\right)=1\Rightarrow \alpha +\frac{1}{3}=1\Rightarrow \alpha =\frac{2}{3}$$$$f{}^{\prime }\left(x\right)=e^{-\frac{x}{2}}(\frac{\sqrt{3}}{2}\beta \cos \left(\frac{\sqrt{3}}{2}x\right)-\frac{\sqrt{3}}{2}\alpha \sin \left(\frac{\sqrt{3}}{2}x\right))-\frac{1}{2}{e}^{-\frac{x}{2}}(\alpha \cos \left(\frac{\sqrt{3}}{2}x\right)+\beta \sin \left(\frac{\sqrt{3}}{2}x\right))+\frac{e^x}{3}$$$$f{}^{\prime }\left(0\right)=0\Rightarrow \frac{\sqrt{3}}{2}\beta -\frac{\alpha }{2}+\frac{1}{3}=\frac{\sqrt{3}}{2}\beta =0\Rightarrow \beta =0\Rightarrow f\left(x\right)=e^{-\frac{x}{2}}\left(\frac{2}{3}\cos \left(\frac{\sqrt{3}}{2}x\right)\right)+\frac{e^x}{3}$$$$\underset{n=0}{\sum ^{\mathrm{\infty }}}\frac{1}{\left(3n\right)!}=f\left(1\right)=\frac{2}{3\sqrt{e}}\cos \left(\frac{\sqrt{3}}{2}\right)+\frac{e}{3}=\frac{1}{3}(e+\frac{2}{\sqrt{e}}\cos \left(\frac{\sqrt{3}}{2}\right))$$

Commented by infinityaction last updated on 22/Oct/22

$$thanks$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com