
Question and Answers Forum
Question Number 32365 by prof Abdo imad last updated on 23/Mar/18
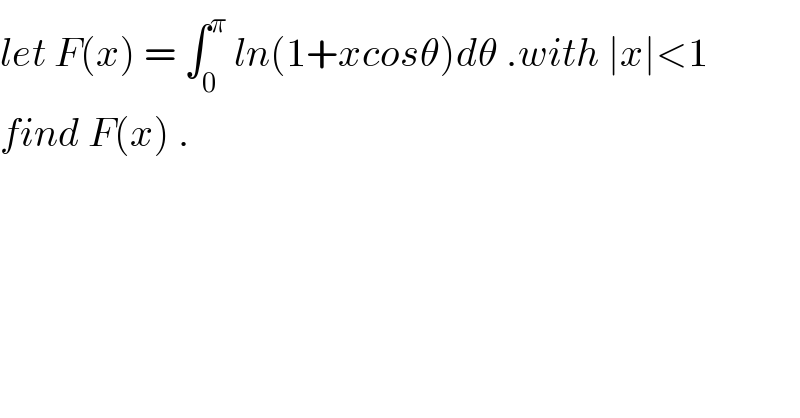
Commented by prof Abdo imad last updated on 25/Mar/18
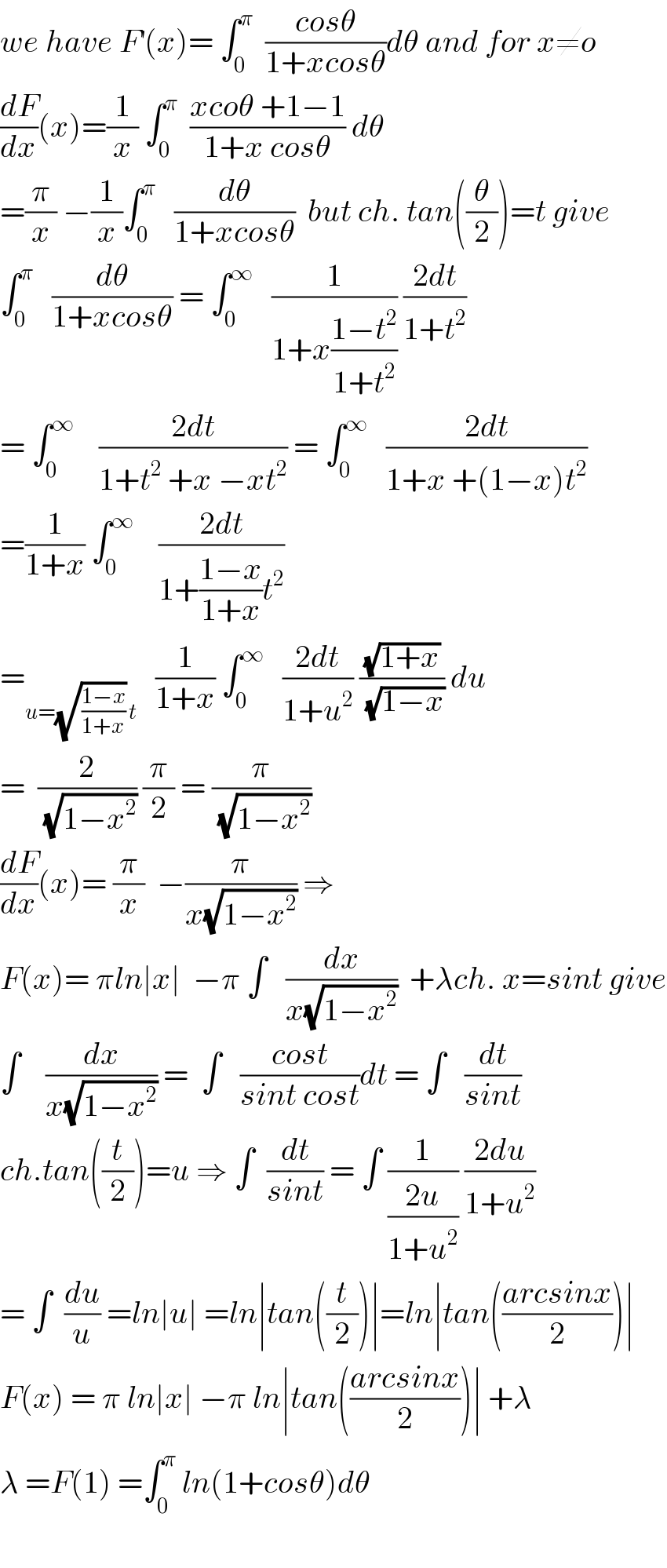
Commented by prof Abdo imad last updated on 25/Mar/18

Commented by prof Abdo imad last updated on 25/Mar/18

