let give the sequence of integrals J_n =∫_0 ^∞ x^n e^(−(x^2 /2)) dx 1) prove that J_n =(n−1)J_(n−2) ∀n≥2 2) calculate J_(2p) and J_(2p+1) by using factoriels. 3) prove that ∀n≥1 J_n ^2 ≤J_(n−1) . J_(n+1) . 4)prove that ((2^(2p) (p!)^2 )/((2p)!)) (1/(√(2p+1))) ≤J_0 ≤ ((2^(2p) (p!)^2 )/((2p)!)) (1/(√(2p))) 5) find a equivalent of ((2^(2p) (p!)^2 )/((2p)!)) (p→+∞)


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 34222 by abdo imad last updated on 03/May/18

$l e t g i v e t h e s e q u e n c e o f i n t e g r a l s$ $J_{n} = \int_{0}^{\infty} x^{n} e^{- \frac{x^{2}}{2}} d x$ $1) p r o v e t h a t J_{n} = (n - 1) J_{n - 2} \forall n ⩾ 2$ $2) c a l c u l a t e J_{2 p} a n d J_{2 p + 1} b y u s i n g f a c t o r i e l s .$ $3) p r o v e t h a t \forall n ⩾ 1 J_{n}^{2} ⩽ J_{n - 1} . J_{n + 1} .$ $4) p r o v e t h a t \frac{2^{2 p} {(p!)}^{2}}{(2 p)!} \frac{1}{\sqrt{2 p + 1}} ⩽ J_{0} ⩽ \frac{2^{2 p} {(p!)}^{2}}{(2 p)!} \frac{1}{\sqrt{2 p}}$ $5) f i n d a e q u i v a l e n t o f \frac{2^{2 p} {(p!)}^{2}}{(2 p)!} (p \to + \infty)$
Commented by candre last updated on 03/May/18

$J_{n} = \underset{0}{\int^{\infty}} x^{n} e^{- \frac{x^{2}}{2}} d x$ $u = x^{n - 1} \Rightarrow d u = (n - 1) x^{n - 2} d x$ $d v = {x e}^{- \frac{x^{2}}{2}} d x \Rightarrow v = - e^{- \frac{x^{2}}{2}}$ $= - {[x^{n - 1} e^{- \frac{x^{2}}{2}}]}_{0}^{\infty} + (n - 1) \int x^{n - 2} e^{- \frac{x^{2}}{2}} d x$ $= (n - 1) J_{n - 2}$ $\int {x e}^{- \frac{x^{2}}{2}} d x = - \int e^{u} d u = - e^{u} = - e^{- \frac{x^{2}}{2}}$ $u = - \frac{x^{2}}{2} \Rightarrow d u = - x d x$
Commented by candre last updated on 03/May/18

$J_{0} = \underset{0}{\int^{\infty}} e^{- \frac{x^{2}}{2}} d x$ $J_{0}^{2} = {(\underset{0}{\int^{\infty}} e^{- \frac{x^{2}}{2}} d x)}^{2} = \underset{0}{\int^{\infty}} e^{- x^{2} / 2} d x \underset{0}{\int^{\infty}} e^{- y^{2} / 2} d y$ $= \underset{0}{\int^{\infty}} \underset{0}{\int^{\infty}} e^{- \frac{x^{2} + y^{2}}{2}} d x d y$ $= \underset{0}{\int^{π / 2}} \underset{0}{\int^{\infty}} e^{- \frac{r^{2}}{2}} r d r d θ$ $= \underset{0}{\int^{π / 2}} d θ \underset{0}{\int^{\infty}} e^{- r^{2} / 2} r d r$ $u = - r^{2} / 2 \Rightarrow d u = - r d r$ $= \frac{π}{2} \cdot \underset{- \infty}{\int^{0}} e^{u} d u$ $= \frac{π}{2}$ $J_{0} = \sqrt{\frac{π}{2}}$
Commented by candre last updated on 03/May/18

$J_{2 p} :$ $J_{2} = 1 \cdot J_{0}$ $J_{4} = 3 \cdot J_{2} = 3 \cdot 1 \cdot J_{0}$ $J_{6} = 5 \cdot J_{4} = 5 \cdot 3 \cdot 1 \cdot J_{0}$ $J_{2 p} = (2 p - 1)!! J_{0}$ $J_{2 p + 1} :$ $J_{3} = 2 \cdot J_{1}$ $J_{5} = 4 \cdot J_{3} = 4 \cdot 2 \cdot J_{1}$ $J_{2 p + 1} = (2 p)!! J_{1} = 2^{p} p! J_{1}$
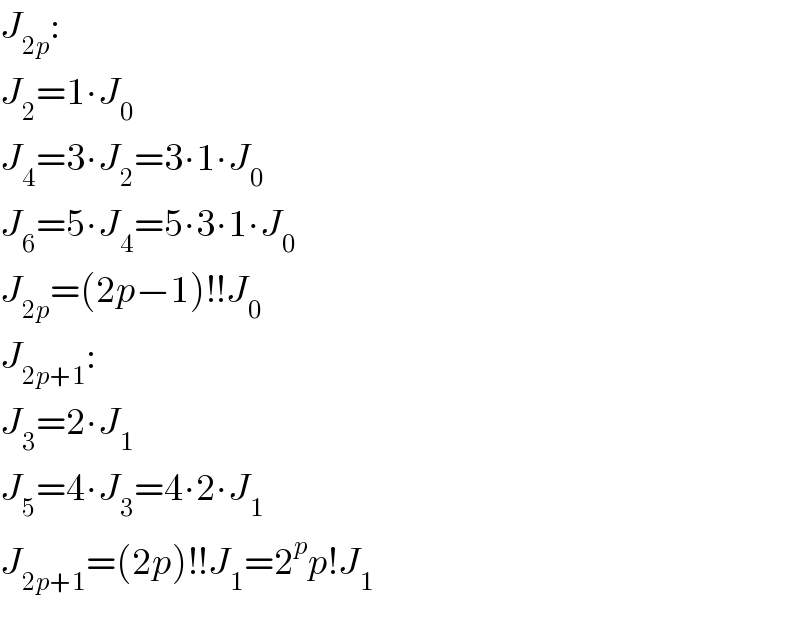
Commented by abdo mathsup 649 cc last updated on 05/May/18

$1) l e t p r o v e t h a t$ $J_{n + 1} = {n J}_{n - 1}$ $J_{n + 1} = \int_{0}^{\infty} x^{n + 1} e^{- \frac{x^{2}}{2}} d x$ $= - \int_{0}^{\infty} x^{n} (- {x e}^{- \frac{x^{2}}{2}} d x) = - ({[x^{n} e^{- \frac{x^{2}}{2}}]}_{0}^{+ \infty} - \int_{0}^{\infty} n x^{n - 1} e^{- \frac{x^{2}}{2}} d x)$ $= n \int_{0}^{\infty} x^{n - 1} e^{- \frac{x^{2}}{2}} d x = n J_{n - 1} \Rightarrow$ $J_{n} = (n - 1) J_{n - 2} \forall n ⩾ 2$ $2) J_{2 p} = (2 p - 1) J_{2 p - 2} \Rightarrow$ $\prod_{p = 1}^{n} J_{2 p} = \prod_{p = 1}^{n} J_{2 p - 2} \prod_{p = 1}^{n} (2 p - 1) \Rightarrow$ $J_{2} . J_{4} . . . . . J_{2 n} = 1.3.5 . . . . (2 n - 1) J_{0} . J_{2} . . . . J_{2 n - 2} \Rightarrow$ $J_{2 n} = 1.3.5 . . . . (2 n - 1) J_{0}$ $= \frac{1.2.3.4 . . . . . . (2 n - 1) (2 n)}{2^{n} n!} J_{0}$ $= \frac{(2 n)!}{2^{n} (n!)} J_{0}$ $J_{0} = \int_{0}^{\infty} e^{- \frac{x^{2}}{2}} d x =_{\sqrt{2} t = x} \int_{0}^{\infty} e^{- t^{2}} \sqrt{2} d t = \sqrt{2} \frac{\sqrt{π}}{2} = \sqrt{\frac{π}{2}}$ $\Rightarrow J_{2 n} = \frac{(2 n)!}{2^{n} n!} \sqrt{\frac{π}{2}}$ $J_{2 p + 1} = 2 p J_{2 p - 1} \Rightarrow \prod_{p = 1}^{n} J_{2 p + 1} = \prod_{p = 1}^{n} (2 p) \prod_{p = 1}^{n} J_{2 p - 1}$ $\Rightarrow J_{3} . J_{5} . . . . . J_{2 n + 1} = 2^{n} n! J_{1} . J_{3} . . . . J_{2 n - 1}$ $\Rightarrow J_{2 n + 1} = 2^{n} n! J_{1}$ $J_{1} = \int_{0}^{\infty} x e^{- \frac{x^{2}}{2}} d x = - \int_{0}^{\infty} (- x) e^{- \frac{x^{2}}{2}} d x$ $= - {[e^{- \frac{x^{2}}{2}}]}_{0}^{+ \infty} = 1 \Rightarrow J_{2 n + 1} = 2^{n} (n!) .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum