
Question and Answers Forum
Question Number 36429 by prof Abdo imad last updated on 02/Jun/18
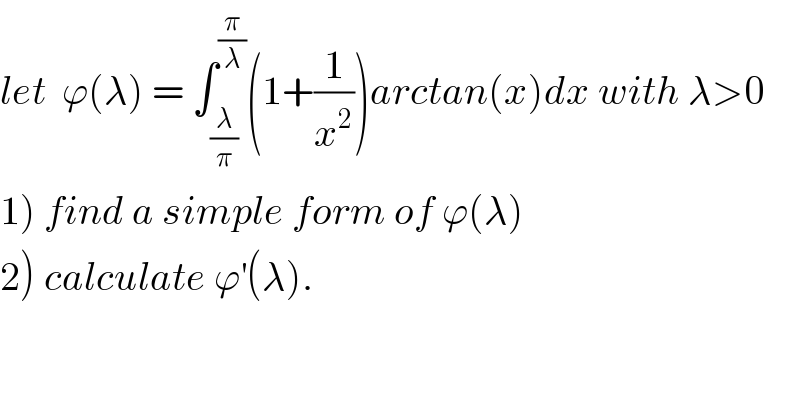
Commented by abdo mathsup 649 cc last updated on 03/Jun/18
![let integrate by parts u^′ =1+(1/x^2 ) and v^′ = arctan(x) ϕ(λ) = [(1−(1/x))arctanx]_(λ/π) ^(π/λ) −∫_(λ/π) ^(π/λ) (1−(1/x)) (dx/(1+x^2 )) =(1−(λ/π))arctan((π/λ)) −(1−(π/λ)) arctan((λ/π)) −∫_(λ/π) ^(π/λ) (((x−1)dx)/(x(1+x^2 ))) but ∫_(λ/π) ^(π/λ) ((x−1)/(x(1+x^2 )))dx = ∫_(λ/π) ^(π/λ) (dx/(1+x^2 )) −∫_(λ/π) ^(π/λ) (dx/(x(1+x^2 ))) = arctan((π/λ)) −arctan((λ/π)) −∫_(λ/π) ^(π/λ) (dx/(x(1+x^2 ))) but F(x)= (1/(x(1+x^2 ))) = (a/x) +((bx+c)/(x^2 +1)) a= 1 and lim_(x→+∞) x F(x)=0= a+b ⇒b=−1 c=0 ⇒ ∫_(λ/π) ^(π/λ) (dx/(x(1+x^2 ))) = ∫_(λ/π) ^(π/λ) { (1/x) −(x/(1+x^2 ))}dx =[ln ∣x∣ −(1/2)ln(1+x^2 )]_(λ/π) ^(π/λ) =[ln∣ (x/(√(1+x^2 )))∣]_(λ/π) ^(π/λ) = ln∣ (π/(λ(√(1+((π/λ))^2 ))))∣−ln∣ (λ/(π(√(1+((λ/π))^2 )))) ϕ(λ) =(1−(λ/π))arctan((π/λ)) −(1−(π/λ))arctan((λ/π)) −ln∣(π/(λ(√(1+((π/λ))^2 ))))∣ +ln∣ (λ/(π(√(1+((λ/π))^2 ))))∣ .](Q36523.png)
Answered by tanmay.chaudhury50@gmail.com last updated on 02/Jun/18
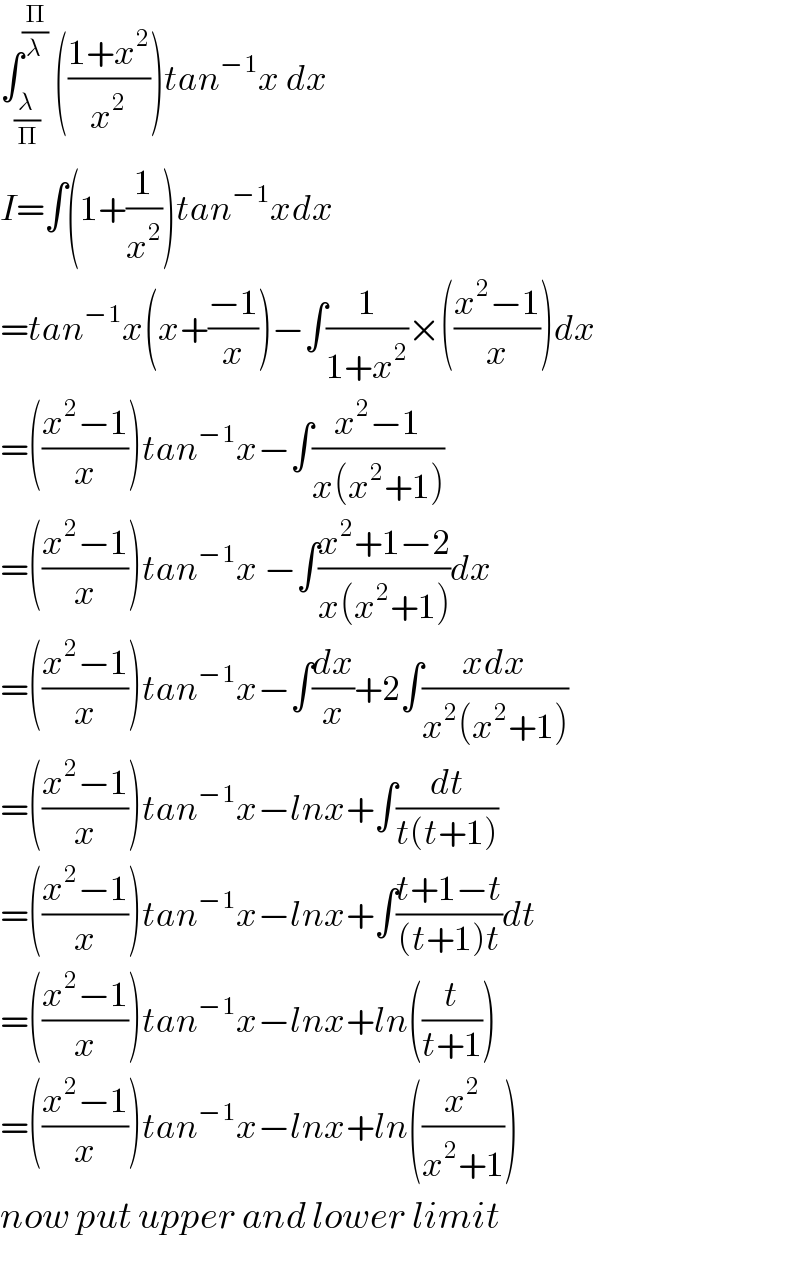
| ||
Question and Answers Forum | ||
Question Number 36429 by prof Abdo imad last updated on 02/Jun/18 | ||
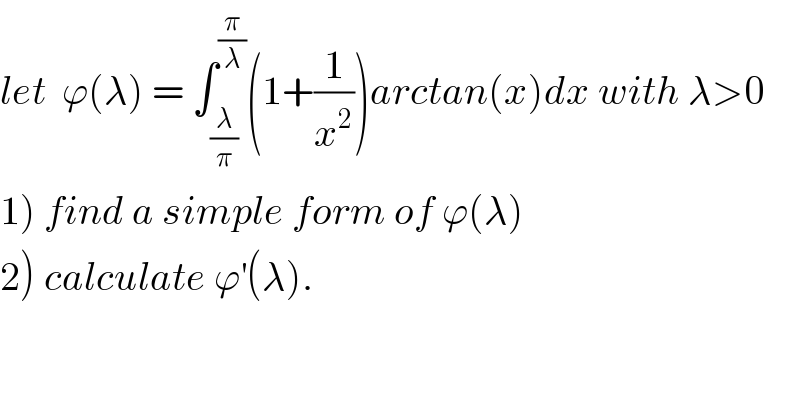 | ||
Commented by abdo mathsup 649 cc last updated on 03/Jun/18 | ||
![let integrate by parts u^′ =1+(1/x^2 ) and v^′ = arctan(x) ϕ(λ) = [(1−(1/x))arctanx]_(λ/π) ^(π/λ) −∫_(λ/π) ^(π/λ) (1−(1/x)) (dx/(1+x^2 )) =(1−(λ/π))arctan((π/λ)) −(1−(π/λ)) arctan((λ/π)) −∫_(λ/π) ^(π/λ) (((x−1)dx)/(x(1+x^2 ))) but ∫_(λ/π) ^(π/λ) ((x−1)/(x(1+x^2 )))dx = ∫_(λ/π) ^(π/λ) (dx/(1+x^2 )) −∫_(λ/π) ^(π/λ) (dx/(x(1+x^2 ))) = arctan((π/λ)) −arctan((λ/π)) −∫_(λ/π) ^(π/λ) (dx/(x(1+x^2 ))) but F(x)= (1/(x(1+x^2 ))) = (a/x) +((bx+c)/(x^2 +1)) a= 1 and lim_(x→+∞) x F(x)=0= a+b ⇒b=−1 c=0 ⇒ ∫_(λ/π) ^(π/λ) (dx/(x(1+x^2 ))) = ∫_(λ/π) ^(π/λ) { (1/x) −(x/(1+x^2 ))}dx =[ln ∣x∣ −(1/2)ln(1+x^2 )]_(λ/π) ^(π/λ) =[ln∣ (x/(√(1+x^2 )))∣]_(λ/π) ^(π/λ) = ln∣ (π/(λ(√(1+((π/λ))^2 ))))∣−ln∣ (λ/(π(√(1+((λ/π))^2 )))) ϕ(λ) =(1−(λ/π))arctan((π/λ)) −(1−(π/λ))arctan((λ/π)) −ln∣(π/(λ(√(1+((π/λ))^2 ))))∣ +ln∣ (λ/(π(√(1+((λ/π))^2 ))))∣ .](Q36523.png) | ||
Answered by tanmay.chaudhury50@gmail.com last updated on 02/Jun/18 | ||
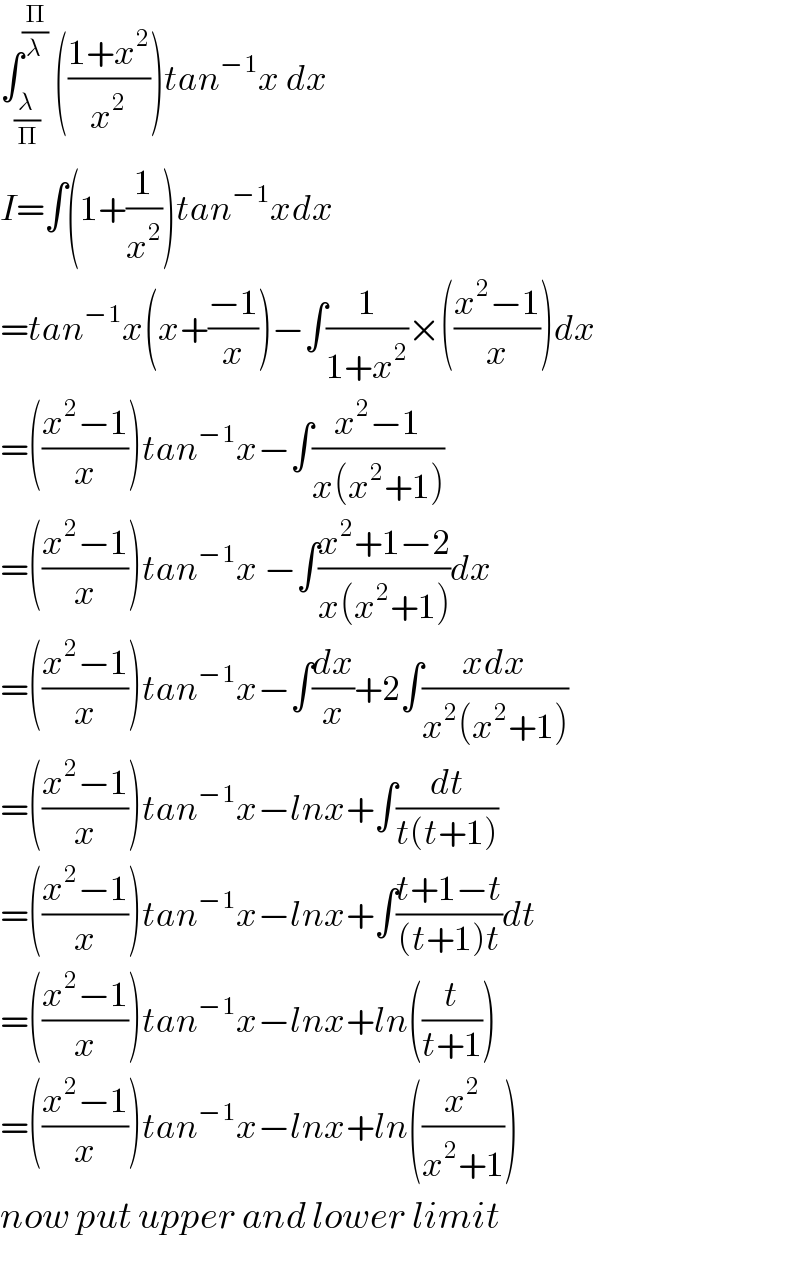 | ||
| ||